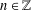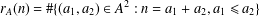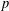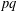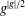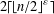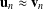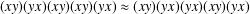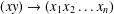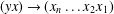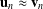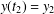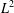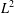Research Article
A UNIQUE REPRESENTATION BI-BASIS FOR THE INTEGERS. II
- Part of:
-
- Published online by Cambridge University Press:
- 08 January 2016, pp. 1-6
-
- Article
-
- You have access
- Export citation
UNIVERSAL QUADRATIC FORMS AND ELEMENTS OF SMALL NORM IN REAL QUADRATIC FIELDS
- Part of:
-
- Published online by Cambridge University Press:
- 11 January 2016, pp. 7-14
-
- Article
-
- You have access
- Export citation
ON EXCEPTIONAL SETS: THE SOLUTION OF A PROBLEM POSED BY K. MAHLER
- Part of:
-
- Published online by Cambridge University Press:
- 12 May 2016, pp. 15-19
-
- Article
-
- You have access
- Export citation
ON ARC-REGULAR FROBENIUS METACIRCULANTS
- Part of:
-
- Published online by Cambridge University Press:
- 10 February 2016, pp. 20-29
-
- Article
-
- You have access
- Export citation
A CHARACTER-THEORETIC CRITERION FOR THE SOLVABILITY OF FINITE GROUPS
- Part of:
-
- Published online by Cambridge University Press:
- 20 January 2016, pp. 30-35
-
- Article
-
- You have access
- Export citation
SYMMETRY CLASSES OF TENSORS ASSOCIATED TO NONABELIAN GROUPS OF ORDER
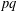 $pq$
$pq$
- Part of:
-
- Published online by Cambridge University Press:
- 16 March 2016, pp. 36-42
-
- Article
-
- You have access
- Export citation
FINDING INVOLUTIONS WITH SMALL SUPPORT
- Part of:
-
- Published online by Cambridge University Press:
- 11 January 2016, pp. 43-47
-
- Article
-
- You have access
- Export citation
GROUPS OF INFINITE RANK WITH NORMALITY CONDITIONS ON SUBGROUPS WITH SMALL NORMAL CLOSURE
- Part of:
-
- Published online by Cambridge University Press:
- 11 November 2015, pp. 48-53
-
- Article
-
- You have access
- Export citation
THE FINITE BASIS PROBLEM FOR THE MONOID OF TWO-BY-TWO UPPER TRIANGULAR TROPICAL MATRICES
- Part of:
-
- Published online by Cambridge University Press:
- 08 January 2016, pp. 54-64
-
- Article
-
- You have access
- Export citation
AN OPEN MAPPING THEOREM
- Part of:
-
- Published online by Cambridge University Press:
- 08 January 2016, pp. 65-69
-
- Article
-
- You have access
- Export citation
RESOLVABILITY OF MEASURABLE SPACES
- Part of:
-
- Published online by Cambridge University Press:
- 11 January 2016, pp. 70-79
-
- Article
-
- You have access
- Export citation
ON THE EXISTENCE OF FINITE CRITICAL TRAJECTORIES IN A FAMILY OF QUADRATIC DIFFERENTIALS
- Part of:
-
- Published online by Cambridge University Press:
- 16 March 2016, pp. 80-91
-
- Article
-
- You have access
- Export citation
A GENERALISATION OF THE CLUNIE–SHEIL-SMALL THEOREM
- Part of:
-
- Published online by Cambridge University Press:
- 17 February 2016, pp. 92-100
-
- Article
-
- You have access
- Export citation
DIFFERENTIATING SOLUTIONS OF A BOUNDARY VALUE PROBLEM ON A TIME SCALE
- Part of:
-
- Published online by Cambridge University Press:
- 01 April 2016, pp. 101-109
-
- Article
-
- You have access
- Export citation
THE QUENCHING OF SOLUTIONS OF A REACTION–DIFFUSION EQUATION WITH FREE BOUNDARIES
- Part of:
-
- Published online by Cambridge University Press:
- 22 January 2016, pp. 110-120
-
- Article
-
- You have access
- Export citation
A NOTE ON THE ENDPOINT REGULARITY OF THE HARDY–LITTLEWOOD MAXIMAL FUNCTIONS
- Part of:
-
- Published online by Cambridge University Press:
- 11 December 2015, pp. 121-130
-
- Article
-
- You have access
- Export citation
SMOOTHNESS OF CONVOLUTION PRODUCTS OF ORBITAL MEASURES ON RANK ONE COMPACT SYMMETRIC SPACES
- Part of:
-
- Published online by Cambridge University Press:
- 12 February 2016, pp. 131-143
-
- Article
-
- You have access
- Export citation
SEMIGROUPS OF COMPOSITION OPERATORS ON LOCAL DIRICHLET SPACES
- Part of:
-
- Published online by Cambridge University Press:
- 16 March 2016, pp. 144-154
-
- Article
-
- You have access
- Export citation
THE GREEN–OSHER INEQUALITY IN RELATIVE GEOMETRY
- Part of:
-
- Published online by Cambridge University Press:
- 17 February 2016, pp. 155-164
-
- Article
-
- You have access
- Export citation
Abstracts of Australasian PhD Theses
SELF-COMPLEMENTARY VERTEX-TRANSITIVE GRAPHS
- Part of:
-
- Published online by Cambridge University Press:
- 07 June 2016, pp. 165-166
-
- Article
-
- You have access
- Export citation