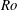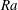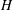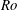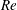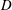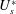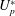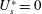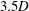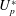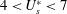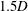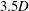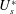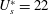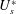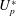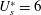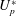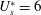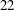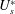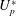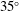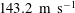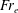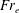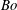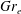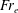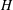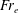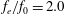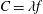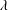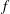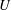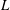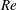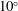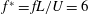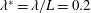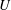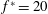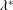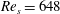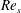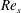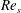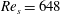Capillary ripples in thin viscous films are important features of coating and lubrication flows. Here, we present experiments based on digital holographic microscopy, measuring with nanoscale resolution the morphology of capillary ripples ahead of a viscous drop spreading on a prewetted surface. Our experiments reveal that upon increasing the spreading velocity, the amplitude of the ripples first increases and subsequently decreases. Above a critical spreading velocity, the ripples even disappear completely and this transition is accompanied by a divergence of the ripple wavelength. These observations are explained quantitatively using linear wave analysis, beyond the usual lubrication approximation, illustrating that new phenomena arise when the capillary number becomes of the order of unity.

Rapid depressurization is a fluid phenomenon that occurs in many industrial and natural applications. Its behaviour is often complicated by the formation, propagation and interaction of waves. In this work, we perform computer simulations of the rapid depressurization of a gas–solid mixture in a shock tube. Our problem set-up mimics previously performed experiments, which have been historically used as a laboratory surrogate for volcanic eruptions. The simulations are carried out with a discontinuous Galerkin compressible fluid solver with four-way coupled Lagrangian particle tracking capabilities. The results give an unprecedented look into the complex multiphase physics at work in this problem. Different regimes have been characterized in a regime map that highlights the key observations. While the mean flow behaviour is in good agreement with experiments, the simulations show unexpected accelerations of the particle front as it expands. Additionally, a new lifting mechanism for gas bubble (void) growth inside the gas–solid mixture is detailed.

Spanwise rotating plane Poiseuille flow (RPPF) is one of the canonical flow problems to study the effect of system rotation on wall-bounded shear flows and has been studied a lot in the past. In the present work, a two-dimensional-three-component (2D/3C) model for RPPF is introduced and it is shown that the present model is equivalent to a thermal convection problem with unit Prandtl number. For low Reynolds number cases, the model can be used to study the stability behaviour of the roll cells. It is found that the neutral stability curves, critical eigensolutions and critical streamfunctions of RPPF at different rotation numbers ($Ro$) almost collapse with the help of a rescaling with a newly defined Rayleigh number $Ra$ and channel height $H$. Analytic expressions for the critical Reynolds number and critical wavenumber at different $Ro$ can be obtained. For a turbulent state with high Reynolds number, the 2D/3C model for RPPF is self-sustained even without extra excitations. Simulation results also show that the profiles of mean streamwise velocity and Reynolds shear stress from the 2D/3C model share the same linear laws as the fully three-dimensional cases, although differences on the intercepts can be observed. The contours of streamwise velocity fluctuations behave like plumes in the linear law region. We also provide an explanation to the linear mean velocity profiles observed at high rotation numbers.

Laser-induced forward transfer (LIFT) is a nozzle-free printing technology that can be used for two- and three-dimensional printing. In LIFT, a laser pulse creates an impulse inside a thin film of material that results in the formation of a liquid jet. We experimentally study LIFT of viscoplastic materials by visualizing the process of jetting with high-speed imaging. The shape of the jet depends on the laser energy, focal height, surface tension and material rheology. We theoretically identify the characteristic jetting velocity and how it depends on the control parameters, and define non-dimensional groups to classify the regimes of jetting. Based on the results, we propose the optimal conditions for printing with LIFT technology.

We quantify the importance of turbulent flow on the propagation of hydraulic fractures (HF) accounting for the addition of friction reducing agents to the fracturing fluid (slickwater fluid). The addition in small quantities of a high molecular weight polymer to water is sufficient to drastically reduce friction of turbulent flow. The maximum drag reduction (MDR) asymptote is always reached during industrial-like injections. The energy required for pumping is thus drastically reduced, allowing for high volume high rate hydraulic fracturing operations at a reasonable cost. We investigate the propagation of a hydraulic fracture propagating in an elastic impermeable homogeneous solid under a constant (and possibly very high) injection rate accounting for laminar and turbulent flow conditions with or without the addition of friction reducers. We solve the near-tip HF problem and estimate the extent of the laminar boundary layer near the fracture tip as a function of a tip Reynolds number for slickwater. We obtain different propagation scalings and transition time scales. This allows us to easily quantify the growth of a radial HF from the early-time turbulent regime(s) to the late-time laminar regimes. Depending on the material and injection parameters, some propagation regimes may actually be bypassed. We derive both accurate and approximate solutions for the growth of radial HF in the different limiting flow regimes (turbulent smooth, rough, MDR) for the zero fracture toughness limit (corresponding to the early stage of propagation of a radial HF). We also investigate numerically the transition(s) between the early-time MDR regime to the late-time laminar regimes (viscosity and toughness) for slickwater fluid. Our results indicate that the effect of turbulent flow on high rate slickwater HF propagation is limited and matters only at early times (at most during the first minutes for industrial hydraulic fracturing operations).

Flow-induced vibration (FIV) of an elastically mounted circular cylinder with an attached splitter plate in uniform flow is studied numerically via a stabilized space–time finite element method. The Reynolds number based on the cylinder diameter 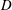$D$ and the free-stream speed is restricted to 150. The ratio of the density of the body to that of the fluid, for the major part of the study, is 10. Two different reduced speeds are defined to quantify the compliance of the elastic support and flexibility of the splitter plate, respectively: 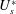$U_{s}^{\ast }$ based on the natural frequency of the spring–mass system and 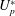$U_{p}^{\ast }$ based on the fundamental natural frequency of the plate. Flow past a stationary cylinder (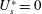$U_{s}^{\ast }=0$) with a flexible splitter plate of length 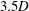$3.5D$ is studied at different values of 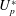$U_{p}^{\ast }$. The vibration response of the plate exhibits lock-in with various eigenmodes of the plate in different ranges of $U_{p}^{\ast }$. The onsets of these lock-in regions are abrupt and hysteretic. The elastically mounted cylinder, without the splitter plate, undergoes large-amplitude vortex-induced vibration (VIV) for 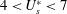$4<U_{s}^{\ast }<7$. These large-amplitude oscillations are a consequence of synchronization, wherein the vortex shedding frequency locks in to the cylinder oscillation frequency. A rigid splitter plate attached to the cylinder reduces significantly the peak amplitude during VIV. Increasing the length of the plate from 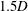$1.5D$ to 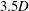$3.5D$ only marginally affects the peak amplitude. It, however, leads to a wider range of lock-in. Unlike the case of an isolated cylinder, the lock-in and desynchronization regimes are not well demarcated in the presence of the splitter plate. Further, galloping is observed beyond a critical value of $U_{s}^{\ast }$; the amplitude of vibration increases with an increase in 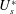$U_{s}^{\ast }$ while the vibration frequency is relatively low and remains nearly constant. Increase in plate length delays, in terms of $U_{s}^{\ast }$, the onset of galloping. It is also found that the flexibility of the plate affects the maximum oscillation amplitude in the VIV regime. It also dictates the presence/absence of galloping. The system behaves similar to an isolated cylinder for a very flexible plate. The response is devoid of galloping, but relatively large amplitude of oscillation is observed during lock-in. The behaviour of the cylinder with a stiff plate is similar to that with the rigid one. The galloping instability sets in when the flexibility of the plate is less than a certain value ($U_{p}^{\ast }<4.7$, approximately for 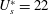$U_{s}^{\ast }=22$). The VIV and galloping are separated by a range of 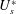$U_{s}^{\ast }$ in which the flow is either steady, for longer plates, or exhibits very weak vortex shedding. In the VIV regime, the plate tip and cylinder vibrate in phase for low $U_{p}^{\ast }$; their motion is out of phase for larger $U_{p}^{\ast }$. The change in phase is also associated with change in the frequency of vibration. At low $U_{p}^{\ast }$, the frequency of vibration is close to the first natural frequency of the system, while at high 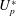$U_{p}^{\ast }$ it becomes closer to the second natural frequency. The vibration amplitude of the cylinder is close to maximum in the VIV regime for 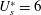$U_{s}^{\ast }=6$. Computations for various 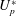$U_{p}^{\ast }$, for 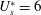$U_{s}^{\ast }=6$ and 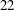$22$, are utilized to determine optimal flexibility that leads to minimal FIV. The effect of the length of the flexible splitter plate, mass ratio and damping ratio is studied. A strategy is proposed to utilize the computations from various combinations of 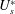$U_{s}^{\ast }$ and 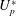$U_{p}^{\ast }$ to choose the appropriate flexibility of the attached splitter plate to minimize FIV.

The azimuthal structure and the generation process of steepened acoustic waves are investigated in the near field of temporal round jets at Mach numbers of 2 and 3. Initially, the shear layers of the jets are in a laminar state and display instability waves whose main properties are close to those predicted from linear temporal analysis. Then, they transition to a turbulent state and generate high-intensity Mach waves displaying sharp compressions typical of those recorded for jets producing crackle noise. These waves are first shown to be poorly reproduced when only the axisymmetric mode is considered, but to be well captured with the first five azimuthal modes. Their generation process is investigated by performing conditional averages of the flow and acoustic fields triggered by the detection of intense positive pressure peak close to the jets. No steepened waves are visible in the conditionally averaged pressure profiles when the procedure involves only one azimuthal mode at a time. However, sharp compressions are obtained based on the first five modes taken together. In that case, the steep compressions are correlated over a limited portion of the jet circumference and are steeper as more azimuthal modes are considered. Moreover, a direct link is established between the steepened waves and the supersonic convection of large-scale coherent flow structures located in the supersonic core of the jets. This indicates that these waves constitute an extreme, nonlinear case of Mach wave radiation by these structures. In addition, the capacity of flow structures to generate sharp, steepened waves is related to their shapes. More particularly, flow structures with a large extent in the radial direction are shown to produce stronger and steeper Mach waves than those that are elongated in the flow direction.

We have investigated the dynamics of a monotrichous bacteria cell near a wall boundary, taking elastic hook flexibility into consideration. Combining theoretical linear stability analysis and direct numerical computations via the boundary element method, we have found that the elastohydrodynamic coupling between the hook elasticity and cell rotational motion enables a stable vertical spinning behaviour like a low-Reynolds-number spinning top. The forwardly rotated flagellum, which generates the force exertion pushing towards the cell body, typically destabilizes the vertical upright position and leads to a boundary-following motion. In contrast, the backward rotation of the flagellum, generating a force pulling the cell body, contributes to stable upright behaviour in a large range of hook rigidity. Further numerical investigations have demonstrated that the non-spherical geometry of the cell body and boundary adhesive interactions affect the bacterial dynamics, leading to complex behaviours such as horizontal spinning and unstable vertical spinning motions, both of which are experimentally observed in Pseudomonas aeruginosa bacteria. These results highlight the rich diversity of bacterial surface motility emerging from mechanical boundary interactions coupled with the cell swimming and hook flexibility.

Atomization of a smooth laminar liquid sheet produced by the oblique impingement of two liquid jets and subjected to transverse acoustic forcing in quiescent ambient is investigated. The acoustic forcing perturbs the liquid sheet perpendicular to its plane, thereby setting up a train of sinuous waves propagating radially outwards from the impingement point. These sheet undulations grow as the wave speed decreases towards the edge of the sheet and the sheet characteristics, like intact length and mean drop size, reduce drastically as compared to the natural breakup. Our observations show that the effect of the acoustic field is perceptible over a continuous range of forcing frequencies. Beyond a certain forcing frequency, called the cutoff frequency, the effect of the external acoustic field ceases. The cutoff frequency is found to be an increasing function of the Weber number. Our measurements of the characteristics of spatially amplifying sinuous waves show that the instabilities responsible for the natural sheet breakup augment in the presence of external forcing. Combining the experimental observations and measurements, we conclude that the linear theory of aerodynamic interaction (Squire’s theory) (Squire, Brit. J. Appl. Phys., vol. 4 (6), 1953, pp. 167–169) predicts the important features of this phenomenon reasonably well.

Direct numerical simulation (DNS) is used to study a separated and rapidly reattached turbulent boundary layer over an idealized $35^{\circ }$ infinite swept wing. The separation and reattachment are induced by a transpiration profile at fixed distance above the layer, with the pressure gradient applied to a well-defined, fully developed, zero-pressure-gradient (ZPG) collateral state. To isolate the influence of the sweep, results are compared with one of our earlier DNS of an unswept flow, with the same chordwise transpiration distribution and appropriate upstream momentum thickness. The independence principle (IP) traditionally proposed for swept wings, which is exact for laminar flows, is found to be close to valid in some regions (bridging the separation/reattachment zone) and to fail in others (in the ZPG layers upstream and downstream of the separation). This is assessed primarily through the skin friction and integral thicknesses. The regions in which the IP is approximately valid correspond to regions of diminished Reynolds-stress divergence, compared to the pressure-gradient magnitude. The mean-velocity profiles exhibit significant skewing as the flow develops, while the velocity magnitude departs only slightly from the ZPG logarithmic profile, even above the separation zone. Implications for Reynolds-averaged turbulence modelling are discussed.

We investigate the impact and penetration of a solid sphere passing through gelatine at various impact speeds up to $143.2~\text{m}~\text{s}^{-1}$. Tests were performed with several concentrations of gelatine. Impacts for low elastic Froude number $\mathit{Fr}_{e}$, a ratio between inertia and gelatine elasticity, resulted in rebound. Higher $\mathit{Fr}_{e}$ values resulted in penetration, forming cavities with prominent surface textures. The overall shape of the cavities resembles those observed in water-entry experiments, yet they appear in a different order with respect to increasing inertia: rebound, quasi-seal, deep-seal, shallow-seal and surface-seal. Remarkably, similar to the $We$–$Bo$ phase diagram in water-entry experiments, the elastic Froude number $\mathit{Fr}_{e}$ and elastic Grashof number $\mathit{Gr}_{e}$ (a ratio between gravity and gelatine elasticity) classify all five different phenomena into distinguishable regimes. We find that $\mathit{Fr}_{e}$ can be a good indicator to describe the cavity length $H$, particularly in the shallow-seal regime. Finally, the evolution of cavity shape, pinch-off depth, and lower cavity radius are investigated for different $\mathit{Fr}_{e}$ values.

The physics of a rotary wing in forward flight are highly complex, particularly when flow separation is involved. The purpose of this work is to assess the role of three-dimensional (3-D) vortex dynamics, with a focus on Coriolis forces, in the evolution of vortices in the reverse flow region of a rotating wing. High-fidelity numerical simulations were performed to recreate the flow about a representative rotating wing in forward flight. A vorticity transport analysis was performed to quantify and compare the magnitudes of 2-D flow physics, vortex tilting and Coriolis effects in the resulting flow fields. Three-dimensional vortex dynamics was found to have a very small impact on the growth and behaviour of vortices in the reverse flow region; in fact, the rate of vortex growth was successfully modelled using a simple 2-D vortex method. The small role of 3-D physics was attributed to the Coriolis and vortex tilting terms being approximately equal and opposite to one another. This ultimately lead to vortex behaviour that more closely resembled a surging wing as opposed to a conventional rotating wing, a feature unique to the reverse flow region.

The current paper presents an experimental study of the energy budget of a two-dimensional internal wave attractor in a trapezoidal domain filled with uniformly stratified fluid. The injected energy flux and the dissipation rate are simultaneously measured from a two-dimensional, two-component, experimental velocity field. The pressure perturbation field needed to quantify the injected energy is determined from the linear inviscid theory. The dissipation rate in the bulk of the domain is directly computed from the measurements, while the energy sink occurring in the boundary layers is estimated using the theoretical expression for the velocity field in the boundary layers, derived recently by Beckebanze et al. (J. Fluid Mech., vol. 841, 2018, pp. 614–635). In the linear regime, we show that the energy budget is closed, in the steady state and also in the transient regime, by taking into account the bulk dissipation and, more importantly, the dissipation in the boundary layers, without any adjustable parameters. The dependence of the different sources on the thickness of the experimental set-up is also discussed. In the nonlinear regime, the analysis is extended by estimating the dissipation due to the secondary waves generated by triadic resonant instabilities, showing the importance of the energy transfer from large scales to small scales. The method tested here on internal wave attractors can be generalized straightforwardly to any quasi-two-dimensional stratified flow.

The flow over a square cylinder controlled by a slot synthetic jet positioned at the front surface is investigated experimentally at different excitation frequencies. The Reynolds number based on the free-stream velocity and the side length of the square cylinder is 1000. The flow visualization was conducted using the laser-induced fluorescence technique. The velocity fields upstream and downstream of the square cylinder were measured synchronously with the two-dimensional time-resolved particle image velocimetry technique. Both the evolution of vortex structures and the characteristic frequencies of upstream and downstream flow fields are presented. The flow dynamics vary significantly with the excitation frequency at a fixed stroke length. During one excitation cycle, the synthetic jet vortex pair deflects to one side and later swings to the other side at a quite small excitation frequency of $f_{e}/f_{0}=0.6$, while it only deflects toward one side and does not turn to the other side at $f_{e}/f_{0}=1.0$. Compared with the natural case, the wake characteristics for the above two cases are not changed much by the synthetic jet adopted. At a moderate excitation frequency of $f_{e}/f_{0}=2.0$, the synthetic jet deflects upwards and downwards alternatively. The upstream flow field has a dominant frequency identical to half of the excitation frequency. Under the perturbations of the synthetic jet, two wake vortex pairs are formed per shedding cycle with a shedding frequency equal to that of the square cylinder without control. At a higher excitation frequency of $f_{e}/f_{0}=3.4$, the synthetic jet keeps deflecting to one side, and the upstream flow field is governed by the excitation frequency. The flow separation on the deflected side is suppressed effectively, and no periodic vortex shedding can be observed in the wake. Statistically, the velocity profiles also change with control. The recirculation bubble length in the wake is shortened, and the time-averaged velocity fluctuation is weakened remarkably. The control effects of the synthetic jet and the continuous jet are compared in this paper when placed at the front surface of a square cylinder.

Oceanic internal waves can be decomposed into an infinite set of modes, and the dominant internal mode 1 waves have been extensively investigated. Although mode 2 waves have been observed, they have not received comparable attention, especially the generation mechanisms. In this work, we examine the generation of mode 2 internal waves by the interaction of mode 1 waves with topography. We use a coupled linear long-wave theory with mode coupling through topography, combined with evolution using a Korteweg–de Vries model, to predict the mode 2 wave amplitude, in an ideal three-layer fluid model, in a smooth density stratification and in two realistic oceanic settings. We find that the mode 2 wave amplitude is usually much smaller than the incident mode 1 wave amplitude and is quite sensitive to the pycnocline thickness, topographic slope and background stratification.

Many aquatic animals propel themselves by generating backward traveling waves over their body, which is thought to reattach the flow when the wave speed ($C=\unicode[STIX]{x1D706}f$, where $\unicode[STIX]{x1D706}$ is wavelength and $f$ is frequency) is larger than the swimming speed ($U$). This has inspired the use of travelling waves, which have recently been generated at low amplitudes using smart materials, to reduce flow separation on an inclined plate. To see if low-amplitude travelling waves (amplitude approximately 0.01 of chord length $L$) can reduce the separation on an inclined plate, large-eddy simulations are performed. The simulations are carried out for Reynolds number ($Re$) 20 000 and an angle of attack of $10^{\circ }$ with different wavelengths and frequencies. The travelling waves at a low reduced frequency ($f^{\ast }=fL/U=6$ and $\unicode[STIX]{x1D706}^{\ast }=\unicode[STIX]{x1D706}/L=0.2$, where $U$ is free-stream velocity) do not affect the flow separation and aerodynamic performance compared to the flat inclined plate. Nevertheless, increasing the wave speed by increasing the reduced frequency to 20 and 30 reduces flow separation. However, increasing the wave speed by increasing the wavelength, in contrast to the common belief, does not monotonically reduce the flow separation. In fact, increasing the wave speed by increasing the wavelength from 0.15 to 0.5 at constant frequency $f^{\ast }=20$ increases the separation, but increasing from 0.5 to 1.0 and 2.0, interestingly, reduces flow separation. These observations indicate that the wave speed is not the only parameter for flow reattachment, but both wavelength and frequency individually impact flow separation by affecting two competing but interconnected mechanisms: the axial momentum, imparted onto the fluid by the undulations, tends to reattach the flow but the lateral velocity tends to detach it. In fact, increasing $f^{\ast }$ and $\unicode[STIX]{x1D706}^{\ast }$ increases both the axial momentum and the lateral velocity, which are competing to attach and detach the flow, respectively.

The mean dynamics in oscillatory channel flow is examined to investigate the dynamical mechanisms underlying the transition to turbulence in oscillatory wall-bounded flow. The analyses employ direct numerical simulation data acquired at three Stokes Reynolds numbers: $Re_{s}=648$, 801 and 1009, where the lower $Re_{s}$ flow is transitional over the entire cycle and the two higher $Re_{s}$ flows exhibit flow characteristics similar to steady turbulent wall-bounded flow during part of the cycle. The flow evolution over a half-period of oscillation for all three $Re_{s}$ is as follows: near-wall streamwise velocity streaks develop during the early accelerating portion of the cycle; then at some later point in the cycle that depends on $Re_{s}$, the near-wall streaks breakdown (demarking the onset of the nonlinear development stage), and the near-wall Reynolds stress grows explosively; the Reynolds stress remains elevated for part of the cycle before diminishing (yet remaining finite) during the late decelerating portion of the cycle. This process is then repeated indefinitely. The present findings demonstrate that transition to turbulence occurs when the nonlinear development stage begins during the accelerating portion of the cycle. This crucially leads to the diminishing importance of the centreline momentum source, the emergence of a locally accelerating/decelerating internal layer centred about the edge of the Stokes layer and the wall-normal rearrangement of the mean forces prior to the start of the decelerating portion of the cycle. The rearrangement of mean forces culminates in a four layer structure in the mean balance of forces. This is significant on a number of accounts since empirical and theoretical evidence suggests that the formation of a four layer structure is an important characteristic of a self-similar hierarchal structure that underlies logarithmic dependence of the mean velocity profile in steady turbulent wall-bounded flows (Klewicki et al., J. Fluid Mech., vol. 638, 2009, pp. 73–93). When the nonlinear development stage begins during the decelerating portion of the cycle (i.e. at $Re_{s}=648$), a four layer structure is not observed in the mean balance of forces and the flow remains weakly transitional over the entire cycle.

The turbulent flow over monochromatic waves of moderate steepness is studied by means of wall resolved large eddy simulations. The simulations cover a range of wave ages and Reynolds numbers. At low wave ages the form drag is highly sensitive to Reynolds number changes, and the interaction between turbulent and wave-induced stresses increases with Reynolds number. At higher wave ages, the flow enters a quasi-laminar regime where wave-induced stresses are primarily balanced by viscous stresses, and the form drag displays a simple Reynolds number dependence. To exploit the quasi-laminar response to the wave kinematics, we split the flow field into a laminar wave-generated response and a turbulent shear flow. The former is driven by the non-homogeneous boundary conditions, whereas the latter is driven by the laminar solution as well as turbulent stresses. For high wave ages, the splitting enables approximate functional dependencies for the form drag to be formulated. In the low wave age regime, where the wave-induced stresses are tightly connected with higher harmonics in the turbulent stresses, the flow is more challenging to analyse. Nevertheless, the importance of higher harmonics in the turbulent stresses can be quantified by explicitly choosing which modes to include in the split-system forcing.

Stability of a solitary wave disturbed by a submerged flat sill is investigated experimentally. For sills narrow compared with the solitary wave, the transmitted waves are found to be unaffected in waveform and amplitude. A wider sill disturbs the solitary wave resulting in the formation of a dispersive wavetrain following the transmitted wave. In some cases, the wave amplitude recovers, despite being perturbed, to the state of an unobstructed solitary-wave state at a certain distance beyond the sill. Wider sills cause wave breaking that occurs over the sill or, in some cases, after the wave passes through the sill. Details of waveform transformation leading toward the breaking and subsequent energy dissipation are discussed.

Turbulence structure resulting from multi-fluid or multi-species, variable-density isotropic turbulence interaction with a Mach 2 shock is studied using turbulence-resolving shock-capturing simulations and Eulerian (grid) and Lagrangian (particle) methods. The complex roles that density plays in the modification of turbulence by the shock wave are identified. Statistical analyses of the velocity gradient tensor (VGT) show that density variations significantly change the turbulence structure and flow topology. Specifically, a stronger symmetrization of the joint probability density function (PDF) of second and third invariants of the anisotropic VGT, PDF$(Q^{\ast },R^{\ast })$, as well as the PDF of the vortex stretching contribution to the enstrophy equation, are observed in the multi-species case. Furthermore, subsequent to the interaction with the shock, turbulent statistics also acquire a differential distribution in regions having different densities. This results in a nearly symmetric PDF$(Q^{\ast },R^{\ast })$ in heavy-fluid regions, while the light-fluid regions retain the characteristic tear-drop shape. To understand this behaviour and the return to ‘standard’ turbulence structure as the flow evolves away from the shock, Lagrangian dynamics of the VGT and its invariants is studied by considering particle residence times and conditional particle variables in different flow regions. 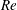The pressure Hessian contributions to the VGT invariants transport equations are shown to be not only affected by the shock wave, but also by the density in the multi-fluid case, making them critically important to the flow dynamics and turbulence structure.