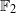 ${ \mathbb{F} }_{2} $-COHOMOLOGY RINGS OF
${ \mathbb{F} }_{2} $-COHOMOLOGY RINGS OF 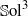 $ \mathbb{S} {\text{ol} }^{3} $-MANIFOLDS
$ \mathbb{S} {\text{ol} }^{3} $-MANIFOLDS
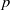 $p$-GROUPS
$p$-GROUPS
 $k$-HESSIAN OPERATORS WITH RESPECT TO LOCAL UNIFORM CONVERGENCE
$k$-HESSIAN OPERATORS WITH RESPECT TO LOCAL UNIFORM CONVERGENCE
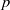 $p$-LAPLACIAN
$p$-LAPLACIAN
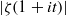 $\vert \zeta (1+ it)\vert $
$\vert \zeta (1+ it)\vert $
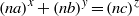 $\mathop{(na)}\nolimits ^{x} + \mathop{(nb)}\nolimits ^{y} = \mathop{(nc)}\nolimits ^{z} $
$\mathop{(na)}\nolimits ^{x} + \mathop{(nb)}\nolimits ^{y} = \mathop{(nc)}\nolimits ^{z} $
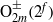 ${ \text{O} }_{2m}^{\pm } ({2}^{f} )$ IN
${ \text{O} }_{2m}^{\pm } ({2}^{f} )$ IN 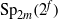 ${\text{Sp} }_{2m} ({2}^{f} )$
${\text{Sp} }_{2m} ({2}^{f} )$