We obtain a uniform linear bound for the Chevalley function at a point in the source of an analytic mapping that is regular in the sense of Gabrielov. There is a version of Chevalley’s lemma also along a fibre, or at a point of the image of a proper analytic mapping. We get a uniform linear bound for the Chevalley function of a closed Nash (or formally Nash) subanalytic set.

We explicitly construct the canonical rational models of Shimura curves, both analytically in terms of modular forms and algebraically in terms of coefficients of genus 2 curves, in the cases of quaternion algebras of discriminant 6 and 10. This emulates the classical construction in the elliptic curve case. We also give families of genus 2 $\text{QM}$ curves, whose Jacobians are the corresponding abelian surfaces on the Shimura curve, and with coefficients that are modular forms of weight 12. We apply these results to show that our $j$-functions are supported exactly at those primes where the genus 2 curve does not admit potentially good reduction, and construct fields where this potentially good reduction is attained. Finally, using $j$, we construct the fields of moduli and definition for some moduli problems associated to the Atkin–Lehner group actions.

This paper is a continuation of three recent articles concerning the structure of hyperinvariant subspace lattices of operators on a (separable, infinite dimensional) Hilbert space $\mathcal{H}$. We show herein, in particular, that there exists a “universal” fixed block-diagonal operator $B$ on $\mathcal{H}$ such that if $\varepsilon >0$ is given and $T$ is an arbitrary nonalgebraic operator on $\mathcal{H}$, then there exists a compact operator $K$ of norm less than $\varepsilon $ such that (i) Hlat$(T)$ is isomorphic as a complete lattice to Hlat$(B+K)$ and (ii) $B+K$ is a quasidiagonal, ${{C}_{00}}$, $(\text{BCP})$-operator with spectrum and left essential spectrum the unit disc. In the last four sections of the paper, we investigate the possible structures of the hyperlattice of an arbitrary algebraic operator. Contrary to existing conjectures, Hlat$(T)$ need not be generated by the ranges and kernels of the powers of $T$ in the nilpotent case. In fact, this lattice can be infinite.

Soit ${{F}_{0}}$ un corps local non archimédien de caractéristique nulle et de caractéristique résiduelle impaire. J. Rogawski a montré l’existence du changement de base entre le groupe unitaire en trois variables $U(2,1)({{F}_{0}})$, défini relativement à une extension quadratique $F$ de ${{F}_{0}}$, et le groupe linéaire $\text{GL}(3,F)$. Par ailleurs, nous avons décrit les représentations supercuspidales irréductibles de $U(2,1)({{F}_{0}})$ comme induites à partir d’un sous-groupe compact ouvert de $U(2,1)({{F}_{0}})$, description analogue à celle des représentations admissibles irréductibles de $\text{GL}(3,F)$ obtenue par C. Bushnell et P. Kutzko. A partir de ces descriptions, nous construisons explicitement le changement de base des représentations très cuspidales de $U(2,1)({{F}_{0}})$.

Maximum principles for subharmonic functions in the framework of quasi-regular local semi-Dirichlet forms admitting lower bounds are presented. As applications, we give weak and strong maximum principles for (local) subsolutions of a second order elliptic differential operator on the domain of Euclidean space under conditions on coefficients, which partially generalize the results by Stampacchia.

We observe that the small quantum product of the generalized flag manifold $G/B$ is a product operation $\star $ on ${{H}^{*}}(G/B)\otimes \mathbb{R}\left[ {{q}_{1,...,}}{{q}_{l}} \right]$ uniquely determined by the facts that it is a deformation of the cup product on ${{H}^{*}}(G/B)$; it is commutative, associative, and graded with respect to deg$({{q}_{i}})=4$; it satisfies a certain relation (of degree two); and the corresponding Dubrovin connection is flat. Previously, we proved that these properties alone imply the presentation of the ring $({{H}^{*}}(G/B)\otimes \mathbb{R}\left[ {{q}_{1,...,}}{{q}_{l}} \right]\star )$ in terms of generators and relations. In this paper we use the above observations to give conceptually new proofs of other fundamental results of the quantum Schubert calculus for $G/B$: the quantum Chevalley formula of D. Peterson (see also Fulton and Woodward) and the “quantization by standard monomials” formula of Fomin, Gelfand, and Postnikov for $G=SL(n,\mathbb{C})$. The main idea of the proofs is the same as in Amarzaya–Guest: from the quantum $\mathcal{D}$-module of $G/B$ one can decode all information about the quantum cohomology of this space.

Let $A$ be a unital commutative associative algebra over a field of characteristic zero, $\mathfrak{k}$ a Lie algebra, and $\mathfrak{z}$ a vector space, considered as a trivial module of the Lie algebra $\mathfrak{g}:=A\otimes \mathfrak{k}$. In this paper, we give a description of the cohomology space ${{H}^{2}}(\mathfrak{g},\mathfrak{z})$ in terms of easily accessible data associated with $A$ and $\mathfrak{k}$. We also discuss the topological situation, where $A$ and $\mathfrak{k}$ are locally convex algebras.

The Kronecker modules, 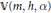$\mathbb{V}\left( m,h,\alpha \right)$, where 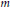$m$ is a positive integer, $h$ is a height function, and $\alpha $ is a 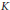$K$-linear functional on the space 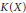$K(X)$ of rational functions in one variable 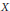$X$ over an algebraically closed field 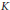$K$, are models for the family of all torsion-free rank-2 modules that are extensions of finite-dimensional rank-1 modules. Every such module comes with a regulating polynomial $f$ in 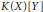$K(X)[Y]$. When the endomorphism algebra of 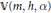$\mathbb{V}\left( m,h,\alpha \right)$ is commutative and non-trivial, the regulator $f$ must be quadratic in $Y$. If $f$ has one repeated root in 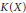$K(X)$, the endomorphism algebra is the trivial extension $K\ltimes S$ for some vector space 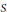$S$. If $f$ has distinct roots in 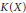$K(X)$, then the endomorphisms form a structure that we call a bridge. These include the coordinate rings of some curves. Regardless of the number of roots in the regulator, those End 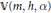$\mathbb{V}\left( m,h,\alpha \right)$ that are domains have zero radical. In addition, each semi-local End 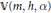$\mathbb{V}\left( m,h,\alpha \right)$ must be either a trivial extension $K\ltimes S$ or the product 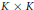$K\times K$.