Hostname: page-component-586b7cd67f-vdxz6 Total loading time: 0 Render date: 2024-11-30T21:18:31.776Z Has data issue: false hasContentIssue false
  • English
  • Français

The Second Cohomology of Current Algebras of General Lie Algebras

Published online by Cambridge University Press:  20 November 2018

Karl-Hermann Neeb  and
Friedrich Wagemann
Karl-Hermann Neeb
Affiliation:
Fachbereich Mathematik, Technische Universität Darmstadt, 64285 Darmstadt, Germany e-mail:[email protected]
Friedrich Wagemann
Affiliation:
Laboratoire de Mathématiques Jean Leray, Faculté des Sciences et Techniques, Université de Nantes, 44322 Nantes cedex 3, France e-mail:[email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

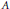Let $A$ be a unital commutative associative algebra over a field of characteristic zero, $\mathfrak{k}$ a Lie algebra, and 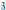$\mathfrak{z}$ a vector space, considered as a trivial module of the Lie algebra 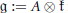$\mathfrak{g}:=A\otimes \mathfrak{k}$. In this paper, we give a description of the cohomology space ${{H}^{2}}(\mathfrak{g},\mathfrak{z})$ in terms of easily accessible data associated with 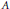$A$ and $\mathfrak{k}$. We also discuss the topological situation, where 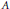$A$ and $\mathfrak{k}$ are locally convex algebras.

Keywords

17B5617B65current algebraLie algebra cohomologyLie algebra homologyinvariant bilinear formcentral extension
Type
Research Article
Information
Canadian Journal of Mathematics , Volume 60 , Issue 4 , 01 August 2008 , pp. 892 - 922
Copyright
Copyright © Canadian Mathematical Society 2008

References

[Bo97] Bordemann, M., Nondegenerate invariant bilinear forms on nonassociative algebras. Acta Math. Univ. Commenianae 66(1997), no. 2, 151–201.Google Scholar
[Bou90] Bourbaki, N., Algebra. I. Ch. 1–3, Springer-Verlag, Berlin, 1990.Google Scholar
[ChE48] Chevalley, C., and Eilenberg, S., Cohomology theory of Lie groups and Lie algebras , Trans. Amer. Math. Soc. 63(1948), 85–124.Google Scholar
[Co85] Connes, A., Non-commutative differential geometry , Publ. Math. Inst. Hautes études Sci. Publ. Math. 62(1985), 257–360.Google Scholar
[Fu86] Fuks, D. B., Cohomology of Infinite Dimensional Lie Algebras. Contemp. Sov.Math., Consultants Bureau, New York, 1986.Google Scholar
[Go55] Goldberg, S. I., On the Euler characteristic of a Lie algebra. Amer.Math. Monthly 62(1955), 239–240.Google Scholar
[Ha92] Haddi, A., Homologie des algèbres de Lie étendues à une algèbre commutative. Comm. Algebra 20(1992), no. 4, 1145–1166.Google Scholar
[HS53] Hochschild, G. and Serre, J.-P., Cohomology of Lie algebras. Ann. of Math. 57(1953), 591–603.Google Scholar
[KL82] Kassel, C., and Loday, J.-L., Extensions centrales d’algèbres de Lie. Ann. Inst. Fourier 32(1982), no. 4, 119–142.Google Scholar
[Kos50] Koszul, J.-L., Homologie et cohomologie des algèbres de Lie. Bull. Soc. Math. France 78(1950), 65–127.Google Scholar
[Lo98] Loday, J.-L., Cyclic Homology. Grundlehren der MathematischenWissenschaften 301, Springer-Verlag, Berlin, 1998 [Ma02] P. Maier, Central extensions of topological current algebras. In: Geometry and Analysis on Finite- and Infinite-Dimensional Lie Groups. Banach Center Publications 55, Polish Academy of Science,Warszawa, 2002, pp. 61–76.Google Scholar
[MN03] Maier, P., and Neeb, K.-H., Central extensions of current groups. Math. Ann. 326(2003), no. 2, 367–415.Google Scholar
[MR93] Medina, A. and Revoy, P., Algèbres de Lie orthogonales, Modules orthogonaux. Comm. Algebra 21(1993), no. 7, 2295–2315.Google Scholar
[MP95] Moody, R. V., and Pianzola, A., Lie Algebras with Triangular Decompositions. Canadian Mathematical Society Series of Monographs and Advanced Texts. John Wiley and Sons, New York, 1995.Google Scholar
[Ne02] Neeb, K.-H., Central extensions of infinite-dimensional Lie groups. Ann. Inst. Fourier (Grenoble) 52(2002), 1365–1442. [Ne02b] , Universal central extensions of Lie groups. Acta Appl. Math. 73(2002), no. 1-2, 175–219.Google Scholar
[Ne04] Neeb, K.-H., Current groups for non-compact manifolds and their central extensions. In: Infinite Dimensional Groups and Manifolds. IRMA Lect. Math. Theor. Phys. 5, de Gruyter Verlag, Berlin, 2004, pp. 109–183 .Google Scholar
[Ne05] Neeb, K.-H., Non-abelian Extensions of topological Lie algebras. Comm. Algebra 34(2006), no. 3, 991–1041.Google Scholar
[Pe97] Pelc, O., A new family of solvable self-dual Lie algebras. J. Math. Phys. 38(1997), no. 7, 3832–3840.Google Scholar
[Zus94] Zusmanovich, P., The second homology group of current Lie algebras. Astérisque 226, 1994, no. 11, 435–452.Google Scholar