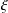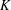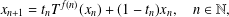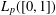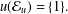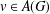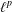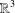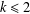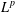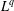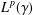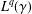Research Article
SHRINKING PROJECTION ALGORITHMS FOR THE SPLIT COMMON NULL POINT PROBLEM
- Part of:
-
- Published online by Cambridge University Press:
- 29 March 2017, pp. 299-306
-
- Article
-
- You have access
- Export citation
ITERATED LOGARITHM SPEED OF RETURN TIMES
- Part of:
-
- Published online by Cambridge University Press:
- 04 October 2017, pp. 468-478
-
- Article
-
- You have access
- Export citation
 $K$-SPHERICAL FUNCTIONS ON ABELIAN SEMIGROUPS
$K$-SPHERICAL FUNCTIONS ON ABELIAN SEMIGROUPS
- Part of:
-
- Published online by Cambridge University Press:
- 13 June 2017, pp. 479-486
-
- Article
-
- You have access
- Export citation
COMPATIBLE LOCALLY CONVEX TOPOLOGIES ON NORMED SPACES: CARDINALITY ASPECTS
- Part of:
-
- Published online by Cambridge University Press:
- 13 March 2017, pp. 139-145
-
- Article
-
- You have access
- Export citation
FIBONACCI–MANN ITERATION FOR MONOTONE ASYMPTOTICALLY NONEXPANSIVE MAPPINGS
- Part of:
-
- Published online by Cambridge University Press:
- 13 March 2017, pp. 307-316
-
- Article
-
- You have access
- Export citation
ON THE EXISTENCE OF
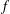 $f$-MAXIMAL SPACELIKE HYPERSURFACES IN CERTAIN WEIGHTED MANIFOLDS
$f$-MAXIMAL SPACELIKE HYPERSURFACES IN CERTAIN WEIGHTED MANIFOLDS
- Part of:
-
- Published online by Cambridge University Press:
- 02 May 2017, pp. 317-325
-
- Article
-
- You have access
- Export citation
SOME CONVERGENCE THEOREMS IN FOURIER ALGEBRAS
- Part of:
-
- Published online by Cambridge University Press:
- 03 July 2017, pp. 487-495
-
- Article
-
- You have access
- Export citation
ON MULTIPLIERS BETWEEN SOME SPACES OF HOLOMORPHIC FUNCTIONS
- Part of:
-
- Published online by Cambridge University Press:
- 29 March 2017, pp. 146-153
-
- Article
-
- You have access
- Export citation
FUNCTIONAL VERSIONS OF SOME REFINED AND REVERSED OPERATOR MEAN INEQUALITIES
- Part of:
-
- Published online by Cambridge University Press:
- 10 August 2017, pp. 496-503
-
- Article
-
- You have access
- Export citation
SELF-SHRINKERS WITH SECOND FUNDAMENTAL FORM OF CONSTANT LENGTH
- Part of:
-
- Published online by Cambridge University Press:
- 02 March 2017, pp. 326-332
-
- Article
-
- You have access
- Export citation
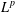 $L^{p}$–
$L^{p}$–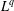 $L^{q}$ OFF-DIAGONAL ESTIMATES FOR THE ORNSTEIN–UHLENBECK SEMIGROUP: SOME POSITIVE AND NEGATIVE RESULTS
$L^{q}$ OFF-DIAGONAL ESTIMATES FOR THE ORNSTEIN–UHLENBECK SEMIGROUP: SOME POSITIVE AND NEGATIVE RESULTS
- Part of:
-
- Published online by Cambridge University Press:
- 06 February 2017, pp. 154-161
-
- Article
-
- You have access
- Export citation
A SCHWARZ LEMMA FOR
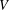 $V$-HARMONIC MAPS AND THEIR APPLICATIONS
$V$-HARMONIC MAPS AND THEIR APPLICATIONS
- Part of:
-
- Published online by Cambridge University Press:
- 17 August 2017, pp. 504-512
-
- Article
-
- You have access
- Export citation
COMPLETE CONVERGENCE FOR ARRAYS AND THE LAW OF THE SINGLE LOGARITHM
- Part of:
-
- Published online by Cambridge University Press:
- 29 August 2017, pp. 333-344
-
- Article
-
- You have access
- Export citation
ON THE CONVERGENCE RATE OF THE KRASNOSEL’SKIĬ–MANN ITERATION
- Part of:
-
- Published online by Cambridge University Press:
- 05 January 2017, pp. 162-170
-
- Article
-
- You have access
- Open access
- Export citation
A SPECIAL CLASS OF QUASI-CYCLIC CODES
- Part of:
-
- Published online by Cambridge University Press:
- 10 August 2017, pp. 513-518
-
- Article
-
- You have access
- Export citation
Abstracts of Australasian PhD Theses
POWER OF POSTS: A QUANTITATIVE ANALYSIS OF FACEBOOK ELECTION CAMPAIGNING INTERACTIONS
- Part of:
-
- Published online by Cambridge University Press:
- 05 July 2017, pp. 345-347
-
- Article
-
- You have access
- Export citation
Research Article
ON KILLERS OF CABLE KNOT GROUPS
- Part of:
-
- Published online by Cambridge University Press:
- 06 February 2017, pp. 171-176
-
- Article
-
- You have access
- Export citation
Front Cover (OFC, IFC) and matter
BAZ volume 96 Issue 1 Cover and Front matter
-
- Published online by Cambridge University Press:
- 06 July 2017, pp. f1-f2
-
- Article
-
- You have access
- Export citation
Abstracts of Australasian PhD Theses
NOVEL PREPROCESSING APPROACHES FOR OMICS DATA TYPES AND THEIR PERFORMANCE EVALUATION
- Part of:
-
- Published online by Cambridge University Press:
- 05 July 2017, pp. 348-349
-
- Article
-
- You have access
- Export citation
DYNAMICS OF MATHEMATICAL MODELS FOR BIOREACTORS
- Part of:
-
- Published online by Cambridge University Press:
- 28 September 2017, pp. 519-520
-
- Article
-
- You have access
- Export citation