Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Zhao, Guangwen
2018.
A volume decreasing theorem for
$$\mathbf{V}$$
V
-harmonic maps and applications.
Archiv der Mathematik,
Vol. 110,
Issue. 6,
p.
629.
Savas-Halilaj, Andreas
2019.
A Schwarz–Pick lemma for minimal maps.
Annals of Global Analysis and Geometry,
Vol. 56,
Issue. 2,
p.
193.
Dong, Yuxin
Ren, Yibin
and
Yu, Weike
2021.
Schwarz Type Lemmas for Pseudo-Hermitian Manifolds.
The Journal of Geometric Analysis,
Vol. 31,
Issue. 3,
p.
3161.
Chen, Qun
Li, Kaipeng
and
Qiu, Hongbing
2022.
A Schwarz lemma and a Liouville theorem for generalized harmonic maps.
Nonlinear Analysis,
Vol. 214,
Issue. ,
p.
112556.
Zhao, Guangwen
2022.
Holomorphic maps into almost Kähler manifolds with constrained energy density.
International Journal of Geometric Methods in Modern Physics,
Vol. 19,
Issue. 08,
Wang, Jie
2024.
A Schwarz lemma of harmonic maps into metric spaces.
Electronic Research Archive,
Vol. 32,
Issue. 11,
p.
5966.
Huang, Xin
and
Yu, Weike
2024.
A Generalization of the Schwarz Lemma for Transversally Harmonic Maps.
The Journal of Geometric Analysis,
Vol. 34,
Issue. 2,
Huang, Xin
2024.
A Schwarz lemma for transversally V-harmonic maps between Riemannian foliated manifolds.
Acta Mathematica Scientia,
Vol. 44,
Issue. 6,
p.
2509.
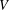 $V$-HARMONIC MAPS AND THEIR APPLICATIONS
$V$-HARMONIC MAPS AND THEIR APPLICATIONS $V$-harmonic maps of generalised dilatation between Riemannian manifolds. We apply the result to obtain corresponding results for Weyl harmonic maps of generalised dilatation from conformal Weyl manifolds to Riemannian manifolds and holomorphic maps from almost Hermitian manifolds to quasi-Kähler and almost Kähler manifolds.
$V$-harmonic maps of generalised dilatation between Riemannian manifolds. We apply the result to obtain corresponding results for Weyl harmonic maps of generalised dilatation from conformal Weyl manifolds to Riemannian manifolds and holomorphic maps from almost Hermitian manifolds to quasi-Kähler and almost Kähler manifolds.