Research Article
Cardinalities of locally compact groups and their Stone-Čech compactifications
-
- Published online by Cambridge University Press:
- 17 April 2009, pp. 353-364
-
- Article
-
- You have access
- Export citation
Extreme points in spaces between Dirichlet and Vanishing Mean Oscillation
-
- Published online by Cambridge University Press:
- 17 April 2009, pp. 365-375
-
- Article
-
- You have access
- Export citation
Weighted discrepancy of Faure-Niederreiter nets for a certain sequence of weights
-
- Published online by Cambridge University Press:
- 17 April 2009, pp. 377-382
-
- Article
-
- You have access
- Export citation
One-sided estimates for quasimonotone increasing functions
-
- Published online by Cambridge University Press:
- 17 April 2009, pp. 383-392
-
- Article
-
- You have access
- Export citation
Derivatives of kernels associated to complex subelliptic operators
-
- Published online by Cambridge University Press:
- 17 April 2009, pp. 393-406
-
- Article
-
- You have access
- Export citation
On incidence rings of group automata
-
- Published online by Cambridge University Press:
- 17 April 2009, pp. 407-411
-
- Article
-
- You have access
- Export citation
Unbounded principal eigenfunctions and the logistic equation on RN
-
- Published online by Cambridge University Press:
- 17 April 2009, pp. 413-427
-
- Article
-
- You have access
- Export citation
S-strictly quasi-concave vector maximisation
-
- Published online by Cambridge University Press:
- 17 April 2009, pp. 429-443
-
- Article
-
- You have access
- Export citation
Structure of the kernels associated with invariant subspaces of the Bergman shift
-
- Published online by Cambridge University Press:
- 17 April 2009, pp. 445-457
-
- Article
-
- You have access
- Export citation
The uniform law of large numbers for the Kaplan-Meier integral process
-
- Published online by Cambridge University Press:
- 17 April 2009, pp. 459-465
-
- Article
-
- You have access
- Export citation
The uniform central limit theorem for the Kaplan-Meier integral process
-
- Published online by Cambridge University Press:
- 17 April 2009, pp. 467-480
-
- Article
-
- You have access
- Export citation
Global existence and comparison theorem for a nonlinear parabolic equation
-
- Published online by Cambridge University Press:
- 17 April 2009, pp. 481-492
-
- Article
-
- You have access
- Export citation
Real hypersurfaces in complex two-plane Grassmannians with parallel shape operator
-
- Published online by Cambridge University Press:
- 17 April 2009, pp. 493-502
-
- Article
-
- You have access
- Export citation
On A Banach space property of Trubnikov
-
- Published online by Cambridge University Press:
- 17 April 2009, pp. 503-510
-
- Article
-
- You have access
- Export citation
Determination of torsion Abelian groups by their automorphism groups
-
- Published online by Cambridge University Press:
- 17 April 2009, pp. 511-519
-
- Article
-
- You have access
- Export citation
Correction
Erratum: Two hyperbolic Shwarz lemmas
-
- Published online by Cambridge University Press:
- 17 April 2009, pp. 521-522
-
- Article
-
- You have access
- Export citation
Abstracts of Australasian PhD Theses
Stochastic analysis and approximation of fractional diffusion
-
- Published online by Cambridge University Press:
- 17 April 2009, pp. 523-525
-
- Article
-
- You have access
- Export citation
On the use of the bispectrum to detect and model non-linearity
-
- Published online by Cambridge University Press:
- 17 April 2009, pp. 527-528
-
- Article
-
- You have access
- Export citation
Front matter
BAZ volume 67 issue 3 Cover and Front matter
-
- Published online by Cambridge University Press:
- 17 April 2009, pp. f1-f6
-
- Article
-
- You have access
- Export citation
Back matter
BAZ volume 67 issue 3 Cover and Back matter
-
- Published online by Cambridge University Press:
- 17 April 2009, pp. b1-b2
-
- Article
-
- You have access
- Export citation

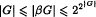 where |
where |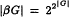 and if
and if 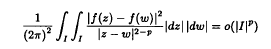
 of the unit disc . It turns out that there is a positive sesquianalytic kernel
of the unit disc . It turns out that there is a positive sesquianalytic kernel 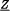
 , where
, where 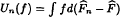 be the function-indexed Kaplan-Meier integral process constructed from the random censorship model. We study a uniform version of the law of large numbers of Glivenko-Cantelli type for {
be the function-indexed Kaplan-Meier integral process constructed from the random censorship model. We study a uniform version of the law of large numbers of Glivenko-Cantelli type for {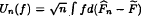 be the Kaplan-Meier integral process constructed from the random censorship model. We prove a uniform central limit theorem for {
be the Kaplan-Meier integral process constructed from the random censorship model. We prove a uniform central limit theorem for {