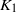28 results
The abelianization of the elementary group of rank two
- Part of
-
- Journal:
- Proceedings of the Edinburgh Mathematical Society , First View
- Published online by Cambridge University Press:
- 20 January 2025, pp. 1-19
-
- Article
- Export citation
Higher semiadditive algebraic K-theory and redshift
- Part of
-
- Journal:
- Compositio Mathematica / Volume 160 / Issue 2 / February 2024
- Published online by Cambridge University Press:
- 15 December 2023, pp. 237-287
- Print publication:
- February 2024
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Fixed point sets and the fundamental group II: Euler characteristics
- Part of
-
- Journal:
- Proceedings of the Royal Society of Edinburgh. Section A: Mathematics / Volume 154 / Issue 6 / December 2024
- Published online by Cambridge University Press:
- 10 October 2023, pp. 1661-1680
- Print publication:
- December 2024
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Fixed point sets and the fundamental group I: semi-free actions on G-CW-complexes
-
- Journal:
- Proceedings of the Royal Society of Edinburgh. Section A: Mathematics / Volume 154 / Issue 6 / December 2024
- Published online by Cambridge University Press:
- 03 August 2023, pp. 1639-1660
- Print publication:
- December 2024
-
- Article
-
- You have access
- HTML
- Export citation
The localisation theorem for the K-theory of stable ∞-categories
- Part of
-
- Journal:
- Proceedings of the Royal Society of Edinburgh. Section A: Mathematics / Volume 154 / Issue 6 / December 2024
- Published online by Cambridge University Press:
- 20 July 2023, pp. 1749-1785
- Print publication:
- December 2024
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
On
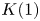 $K(1)$-local
$K(1)$-local 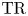 $\mathrm {TR}$
$\mathrm {TR}$
- Part of
-
- Journal:
- Compositio Mathematica / Volume 157 / Issue 5 / May 2021
- Published online by Cambridge University Press:
- 04 May 2021, pp. 1079-1119
- Print publication:
- May 2021
-
- Article
- Export citation
ISOMORPHISMS UP TO BOUNDED TORSION BETWEEN RELATIVE
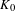 $K_{0}$-GROUPS AND CHOW GROUPS WITH MODULUS
$K_{0}$-GROUPS AND CHOW GROUPS WITH MODULUS
- Part of
-
- Journal:
- Journal of the Institute of Mathematics of Jussieu / Volume 20 / Issue 6 / November 2021
- Published online by Cambridge University Press:
- 18 March 2020, pp. 1947-1968
- Print publication:
- November 2021
-
- Article
-
- You have access
- Open access
- Export citation
Excision in algebraic
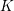 $K$-theory revisited
$K$-theory revisited
- Part of
-
- Journal:
- Compositio Mathematica / Volume 154 / Issue 9 / September 2018
- Published online by Cambridge University Press:
- 06 August 2018, pp. 1801-1814
- Print publication:
- September 2018
-
- Article
- Export citation
 $K$-THEORY OF MONOID ALGEBRAS AND A QUESTION OF GUBELADZE
$K$-THEORY OF MONOID ALGEBRAS AND A QUESTION OF GUBELADZE
- Part of
-
- Journal:
- Journal of the Institute of Mathematics of Jussieu / Volume 18 / Issue 5 / September 2019
- Published online by Cambridge University Press:
- 10 August 2017, pp. 1051-1085
- Print publication:
- September 2019
-
- Article
- Export citation
ON THE MOTIVIC SPECTRAL SEQUENCE
- Part of
-
- Journal:
- Journal of the Institute of Mathematics of Jussieu / Volume 17 / Issue 1 / February 2018
- Published online by Cambridge University Press:
- 25 November 2015, pp. 137-170
- Print publication:
- February 2018
-
- Article
- Export citation
On exact
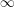 $\infty$-categories and the Theorem of the Heart
$\infty$-categories and the Theorem of the Heart
- Part of
-
- Journal:
- Compositio Mathematica / Volume 151 / Issue 11 / November 2015
- Published online by Cambridge University Press:
- 19 August 2015, pp. 2160-2186
- Print publication:
- November 2015
-
- Article
- Export citation
The triangulated category of K-motives
-
- Journal:
- Journal of K-Theory / Volume 14 / Issue 1 / August 2014
- Published online by Cambridge University Press:
- 12 May 2014, pp. 103-137
- Print publication:
- August 2014
-
- Article
- Export citation
Noncommutative motives of Azumaya algebras
- Part of
-
- Journal:
- Journal of the Institute of Mathematics of Jussieu / Volume 14 / Issue 2 / April 2015
- Published online by Cambridge University Press:
- 10 March 2014, pp. 379-403
- Print publication:
- April 2015
-
- Article
- Export citation
On the algebraic K-theory of truncated polynomial algebras in several variables
-
- Journal:
- Journal of K-Theory / Volume 13 / Issue 1 / February 2014
- Published online by Cambridge University Press:
- 18 February 2014, pp. 57-81
- Print publication:
- February 2014
-
- Article
- Export citation
Connective Algebraic K-theory
-
- Journal:
- Journal of K-Theory / Volume 13 / Issue 1 / February 2014
- Published online by Cambridge University Press:
- 02 January 2014, pp. 9-56
- Print publication:
- February 2014
-
- Article
- Export citation
K′-Theory of a Local Ring of Finite Cohen-Macaulay Type
-
- Journal:
- Journal of K-Theory / Volume 12 / Issue 3 / December 2013
- Published online by Cambridge University Press:
- 07 August 2013, pp. 405-432
- Print publication:
- December 2013
-
- Article
- Export citation
Relative reciprocities on Dedekind domains
-
- Journal:
- Journal of K-Theory / Volume 12 / Issue 1 / August 2013
- Published online by Cambridge University Press:
- 03 June 2013, pp. 125-136
- Print publication:
- August 2013
-
- Article
- Export citation
Quillen's work on the Adams Conjecture
-
- Journal:
- Journal of K-Theory / Volume 11 / Issue 3 / June 2013
- Published online by Cambridge University Press:
- 11 March 2013, pp. 517-526
- Print publication:
- June 2013
-
- Article
- Export citation
QUASI MULTIPLICATION AND
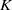 $K$-GROUPS
$K$-GROUPS
- Part of
-
- Journal:
- Journal of the Australian Mathematical Society / Volume 94 / Issue 2 / April 2013
- Published online by Cambridge University Press:
- 28 February 2013, pp. 257-267
- Print publication:
- April 2013
-
- Article
-
- You have access
- Export citation
K1 of Exact Categories by Mirror Image Sequences
-
- Journal:
- Journal of K-Theory / Volume 11 / Issue 1 / February 2013
- Published online by Cambridge University Press:
- 19 April 2012, pp. 155-181
- Print publication:
- February 2013
-
- Article
- Export citation

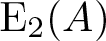
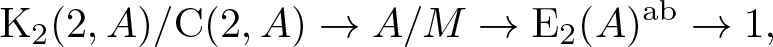
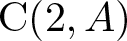

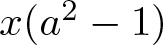
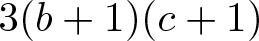
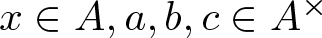
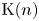
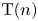

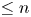
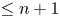
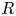
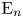
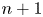
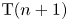

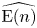


















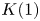
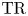
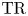
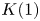
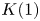
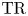
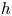
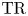
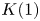
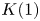
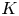
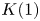

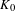
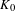
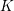
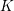
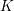

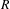
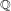
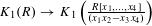
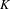
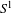
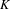
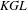
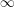
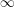
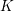
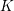
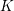
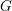

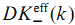
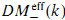 . To each smooth
. To each smooth  is associated in the category
is associated in the category 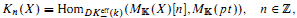
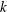
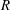
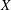
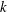
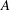
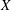

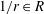
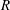
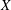
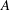
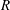
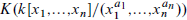 . This naturally leads to a new generalization of the big Witt vectors. If
. This naturally leads to a new generalization of the big Witt vectors. If 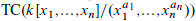 as the iterated homotopy cofiber of an
as the iterated homotopy cofiber of an  , defined by taking the −1 connective cover of algebraic
, defined by taking the −1 connective cover of algebraic 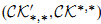 when the base scheme is the spectrum of a field
when the base scheme is the spectrum of a field 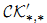 may be viewed as connective algebraic
may be viewed as connective algebraic 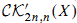 for
for 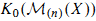 in
in 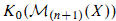 , where
, where 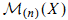 is the abelian category of coherent sheaves on
is the abelian category of coherent sheaves on 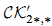 with the universal oriented Borel-Moore homology theory
with the universal oriented Borel-Moore homology theory 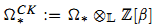 having formal group law
having formal group law