We establish multiplicity results for the following class of quasilinear problems
P
\begin{equation*} \left\{ \begin{array}{@{}l} -\Delta_{\Phi}u=f(x,u) \quad \mbox{in} \quad \Omega, \\ u=0 \quad \mbox{on} \quad \partial \Omega, \end{array} \right. \end{equation*}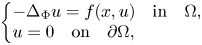
where
$\Delta _{\Phi }u=\text {div}(\varphi (x,|\nabla u|)\nabla u)$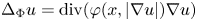
for a generalized N-function
$\Phi (x,t)=\int _{0}^{|t|}\varphi (x,s)s\,ds$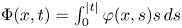
. We consider
$\Omega \subset \mathbb {R}^{N}$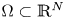
to be a smooth bounded domain that contains two disjoint open regions
$\Omega _N$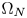
and
$\Omega _p$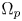
such that
$\overline {\Omega _N}\cap \overline {\Omega _p}=\emptyset$
. The main feature of the problem
$(P)$
is that the operator
$-\Delta _{\Phi }$
behaves like
$-\Delta _N$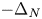
on
$\Omega _N$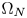
and
$-\Delta _p$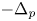
on
$\Omega _p$
. We assume the nonlinearity
$f:\Omega \times \mathbb {R}\to \mathbb {R}$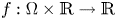
of two different types, but both behave like
$e^{\alpha |t|^{\frac {N}{N-1}}}$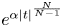
on
$\Omega _N$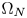
and
$|t|^{p^{*}-2}t$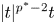
on
$\Omega _p$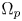
as
$|t|$
is large enough, for some
$\alpha >0$
and
$p^{*}=\frac {Np}{N-p}$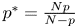
being the critical Sobolev exponent for
$1< p< N$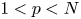
. In this context, for one type of nonlinearity
$f$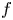
, we provide a multiplicity of solutions in a general smooth bounded domain and for another type of nonlinearity
$f$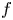
, in an annular domain
$\Omega$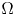
, we establish existence of multiple solutions for the problem
$(P)$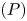
that are non-radial and rotationally non-equivalent.