No CrossRef data available.
Article contents
On the algebraicity about the Hodge numbers of the Hilbert schemes of algebraic surfaces
Published online by Cambridge University Press: 19 April 2022
Abstract
Hilbert schemes are an object arising from geometry and are closely related to physics and modular forms. Recently, there have been investigations from number theorists about the Betti numbers and Hodge numbers of the Hilbert schemes of points of an algebraic surface. In this paper, we prove that Göttsche's generating function of the Hodge numbers of Hilbert schemes of $n$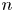 points of an algebraic surface is algebraic at a CM point $\tau$
points of an algebraic surface is algebraic at a CM point $\tau$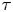 and rational numbers $z_1$
and rational numbers $z_1$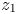 and $z_2$
and $z_2$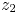 . Our result gives a refinement of the algebraicity on Betti numbers.
. Our result gives a refinement of the algebraicity on Betti numbers.
MSC classification
- Type
- Research Article
- Information
- Copyright
- Copyright © The Author(s), 2022. Published by Cambridge University Press on Behalf of The Edinburgh Mathematical Society



