Most cited
This page lists all time most cited articles for this title. Please use the publication date filters on the left if you would like to restrict this list to recently published content, for example to articles published in the last three years. The number of times each article was cited is displayed to the right of its title and can be clicked to access a list of all titles this article has been cited by.
- Cited by 1
Centers and Azumaya loci for finite W-algebras in positive characteristic
- Part of:
-
- Published online by Cambridge University Press:
- 24 May 2021, pp. 35-66
-
- Article
- Export citation
- Cited by 1
An infinite integral
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 323-324
-
- Article
- Export citation
- Cited by 1
Modified propagation functions in perturbation theory
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 638-641
-
- Article
- Export citation
- Cited by 1
Projections of Poisson cut-outs in the Heisenberg group and the visual 3-sphere
- Part of:
-
- Published online by Cambridge University Press:
- 08 March 2021, pp. 197-230
-
- Article
- Export citation
- Cited by 1
Norm preserving interpolation sets for polydisc algebras
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 291-303
-
- Article
- Export citation
- Cited by 1
On loci which have two systems of generating spaces
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 319-324
-
- Article
- Export citation
- Cited by 1
Still another way to calculate representations of the three-dimensional pure rotation group
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 273-275
-
- Article
- Export citation
- Cited by 1
Kernels with only a finite number of characteristic values
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 257-262
-
- Article
- Export citation
- Cited by 1
The thermal boundary layer in the flow between converging plane walls
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 225-229
-
- Article
- Export citation
- Cited by 1
On continuous functions with graphs of σ-finite linear measure
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 33-43
-
- Article
- Export citation
- Cited by 1
Further transformable nth order differential equations with transition points of particular order m
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 145-157
-
- Article
- Export citation
- Cited by 1
Escaping Fatou components of transcendental self-maps of the punctured plane
- Part of:
-
- Published online by Cambridge University Press:
- 28 November 2019, pp. 265-289
-
- Article
- Export citation
- Cited by 1
On the forced torsional oscillations of an elastic cylinder
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 955-967
-
- Article
- Export citation
- Cited by 1
Integrals involving classical polynomials
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 607-611
-
- Article
- Export citation
- Cited by 1
The L1-norm of exponential sums in
 d
d
-
- Published online by Cambridge University Press:
- 16 January 2013, pp. 381-392
-
- Article
- Export citation
- Cited by 1
Generic maps of the projective plane with a single triple point
-
- Published online by Cambridge University Press:
- 22 February 2012, pp. 455-472
-
- Article
- Export citation
- Cited by 1
On a conjecture of D. E. Littlewood
-
- Published online by Cambridge University Press:
- 24 October 2008, p. 203
-
- Article
- Export citation
- Cited by 1
Extracting almost symmetric sequences in rearrangement invariant spaces
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 303-316
-
- Article
- Export citation
- Cited by 1
PSP volume 59 issue 2 Cover and Back matter
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. b1-b2
-
- Article
-
- You have access
- Export citation
- Cited by 1
Inclusion conditions for Hurwitzian and Schur sets in
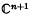
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 113-120
-
- Article
- Export citation


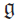

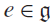
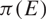

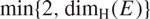
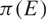
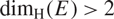
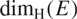
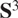
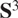
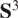

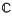

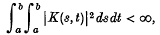
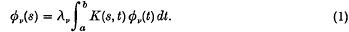

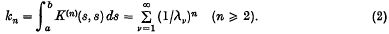

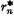 and
and  , respectively, as the minimum and maximum moduli of the zeros of
, respectively, as the minimum and maximum moduli of the zeros of 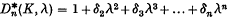 , the
, the 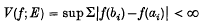
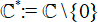
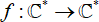
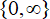
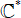


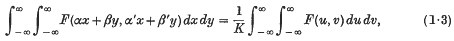

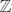
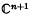
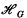 of
of 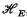 , where
, where 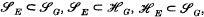 where
where 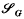 denotes the Schur set of
denotes the Schur set of 