Research Article
A note on some intermediate propositional calculi
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 329-333
-
- Article
- Export citation
Functors and ordinal notations. I: A functorial construction of the veblen hierarchy
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 713-729
-
- Article
- Export citation
Magidor-Malitz quantifiers in modules
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 1-8
-
- Article
- Export citation
Simplified morasses with linear limits
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 1001-1021
-
- Article
- Export citation
Other
Foreword to the Revised Edition
-
- Published online by Cambridge University Press:
- 25 July 2022, p. i
-
- Article
- Export citation
Research Article
Some properties of invariant sets
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 9-21
-
- Article
- Export citation
Relevant entailment—semantics and formal systems
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 334-342
-
- Article
- Export citation
Other
A Bibliography of Symbolic Logic
-
- Published online by Cambridge University Press:
- 25 July 2022, pp. 1-96
-
- Article
- Export citation
Research Article
An interpretation of Martin-Löf's type theory in a type-free theory of propositions
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 730-753
-
- Article
- Export citation
Diamonds, uniformization
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 1022-1033
-
- Article
- Export citation
More on proper forcing
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 1034-1038
-
- Article
- Export citation
Additions and Corrections
Additions and Corrections to a Bibliography of Symbolic Logic
-
- Published online by Cambridge University Press:
- 25 July 2022, pp. 98-113
-
- Article
- Export citation
Research Article
Stepping up lemmas in definable partitions
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 22-31
-
- Article
- Export citation
Self-reference with negative types
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 754-773
-
- Article
- Export citation
Banach games
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 343-375
-
- Article
- Export citation
The strength of nonstandard methods in arithmetic
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 1039-1058
-
- Article
- Export citation
Addendum
Addendum
-
- Published online by Cambridge University Press:
- 25 July 2022, pp. 115-132
-
- Article
- Export citation
Research Article
A recursion theoretic analysis of the clopen Ramsey theorem
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 376-400
-
- Article
- Export citation
Monotone reducibility and the family of infinite sets
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 774-782
-
- Article
- Export citation
Some model theory of modules. III. On infiniteness of sets definable in modules
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 32-46
-
- Article
- Export citation

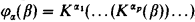 , with
, with 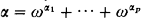 (not necessarily a Cantor normal form) and
(not necessarily a Cantor normal form) and 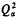 -core sentences and positive primitive formulas. For complete extensions of the elementary theory of R-modules it follows that all Ramsey quantifiers (ℵ
-core sentences and positive primitive formulas. For complete extensions of the elementary theory of R-modules it follows that all Ramsey quantifiers (ℵ ,
, 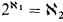 ,
, 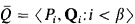
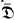 -
-
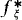 :
: 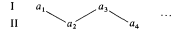
 exists and
exists and 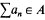 .
.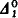 sets are all reducible to the (
sets are all reducible to the (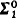 but not
but not 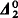 sets, which are all in turn reducible to the strictly
sets, which are all in turn reducible to the strictly  sets. In addition, the nontrivial
sets. In addition, the nontrivial  sets all have the same degree for
sets all have the same degree for 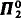 monotone sets which have different monotone degrees. We show that every
monotone sets which have different monotone degrees. We show that every