There is renewed interest in direct-drive inertial confinement fusion, following the milestone December 2022 3.15 MJ ignition result on the National Ignition Facility. A key obstacle is the control of the two-plasmon decay instability. Here, recent advances in inhomogeneous turbulence theory are applied to the broadband parametric instability problem for the first time. A novel dispersion relation is derived for the two-plasmon decay in a uniform plasma valid under broad-bandwidth laser fields with arbitrary power spectra. The effects of temporal incoherence on the instability are then studied. In the limit of large bandwidth, the well-known scaling relations for the growth rate are recovered, but it is shown that the result is more sensitive to the spectral shape of the laser pulse rather than to its coherence time. The range of wavenumbers of the excited plasma waves is shown to be substantially broadened, suggesting that the absolute instability is favoured in regions further away from the quarter critical density. The intermediate-bandwidth regime is explored numerically – the growth rate is reduced to half its monochromatic value for laser intensities of $10^{15} \ \text {W}\ \text {cm}^{-2}$ and relatively modest bandwidths of $5 \ \text {THz}$. The instability-quenching properties of a spectrum of discrete lines spread over some bandwidth have also been studied. The reduction in the growth rate is found to be somewhat lower compared with the continuous case but is still significant, despite the fact that, formally, the coherence time of such a laser pulse is infinite.

Optimising stellarators for quasisymmetry leads to strongly reduced collisional transport and energetic particle losses compared with unoptimised configurations. Although stellarators with precise quasisymmetry have been obtained in the past, it remains unclear how broad the parameter space is where good quasisymmetry may be achieved. We study the range of aspect ratios and rotational transform values for which stellarators with excellent quasisymmetry on the boundary can be obtained. A large number of Fourier harmonics is included in the boundary representation, which is made computationally tractable by the use of adjoint methods to enable fast gradient-based optimisation and by the direct optimisation of vacuum magnetic fields, which converge more robustly compared with solutions from magnetohydrostatics. Several novel configurations are presented, including stellarators with record levels of quasisymmetry on a surface, three field period quasiaxisymmetric stellarators with substantial magnetic shear, and compact quasisymmetric stellarators at low aspect ratios similar to tokamaks.

We study the tearing instability of a current sheet in a relativistic pair plasma with a power-law distribution function. We first estimate the growth rate analytically and then confirm the analytical results by solving numerically the dispersion equation, taking into account all exact particle trajectories within the reconnecting layer. We found that the instability is suppressed when the particle spectrum becomes harder.

We present a framework for analysing plasma flow in a rotating mirror. By making a series of physical assumptions, we reduce the magnetohydrodynamic (MHD) equations in a three-dimensional cylindrical system to a one-dimensional system in a shallow, cuboidal channel within a transverse magnetic field, similar to the Hartmann flow in ducts. We then solve the system both numerically and analytically for a range of values of the Hartmann number and calculate the dependence of the plasma flow speed on the thickness of the insulating end cap. We observe that the mean flow overshoots and decelerates before achieving a steady-state value, a phenomenon that the analytical model cannot capture. This overshoot is directly proportional to the thickness of the insulating end cap and the external electric field, with a weak dependence on the external magnetic field. Our simplified model can act as a benchmark for future simulations of the supersonic mirror device CMFX (centrifugal magnetic fusion experiment), which will employ more sophisticated physics and realistic magnetic field geometries.

On the Experimental Advanced Superconducting Tokamak (EAST), the electron cyclotron wave (ECW) and lower hybrid wave (LHW) are actively used to achieve a high-performance plasma. Turbulence associated with the combined heating experiment is studied numerically based on the gyrokinetic toroidal code (GTC). The linear simulation results show that the unstable mode peaks at $k_{\theta }\rho _{s}\approx 0.65$ and $k_{\theta }\rho _{s}\approx 1.42$. Meanwhile, all of the frequencies of these instabilities are positive, which suggests that the collisionless trapped electron mode (CTEM) is the dominant instability. In the process of nonlinear simulations, a higher transport level is locally achieved during the two waves combined heating due to a formation of a steeper electron temperature gradient. In addition, a low-frequency geodesic acoustic mode (GAM) is observed in the nonlinear stage. Effects of the electron beta, the dimensionless ratio of $T_e/T_i$ and $R/L_{T_e}$ on the growth rate of instability, are also discussed in the paper.

Young stellar objects (YSOs) are protostars that exhibit bipolar outflows fed by accretion disks. Theories of the transition between disk and outflow often involve a complex magnetic field structure thought to be created by the disk coiling field lines at the jet base; however, due to limited resolution, these theories cannot be confirmed with observation and thus may benefit from laboratory astrophysics studies. We create a dynamically similar laboratory system by driving a $\sim$1 MA current pulse with a 200 ns rise through a $\approx$2 mm-tall Al cylindrical wire array mounted to a three-dimensional (3-D)-printed, stainless steel scaffolding. This system creates a plasma that converges on the centre axis and ejects cm-scale bipolar outflows. Depending on the chosen 3-D-printed load path, the system may be designed to push the ablated plasma flow radially inwards or off-axis to make rotation. In this paper, we present results from the simplest iteration of the load which generates radially converging streams that launch non-rotating jets. The temperature, velocity and density of the radial inflows and axial outflows are characterized using interferometry, gated optical and ultraviolet imaging, and Thomson scattering diagnostics. We show that experimental measurements of the Reynolds number and sonic Mach number in three different stages of the experiment scale favourably to the observed properties of YSO jets with $Re\sim 10^5\unicode{x2013}10^9$ and $M\sim 1\unicode{x2013}10$, while our magnetic Reynolds number of $Re_M\sim 1\unicode{x2013}15$ indicates that the magnetic field diffuses out of our plasma over multiple hydrodynamical time scales. We compare our results with 3-D numerical simulations in the PERSEUS extended magnetohydrodynamics code.

A new approach for constructing polar-like boundary-conforming coordinates inside a toroid with strongly shaped cross-sections is presented. A coordinate mapping is obtained through a variational approach, which involves identifying extremal points of a proposed action in the mapping space from $[0, 2{\rm \pi} ]^2 \times [0, 1]$ to a toroidal domain in $\mathbb {R}^3$. This approach employs an action built on the squared Jacobian and radial length. Extensive testing is conducted on general toroidal boundaries using a global Fourier–Zernike basis via action minimisation. The results demonstrate successful coordinate construction capable of accurately describing strongly shaped toroidal domains. The coordinate construction is successfully applied to the computation of three-dimensional magnetohydrodynamic equilibria in the GVEC code where the use of traditional coordinate construction by interpolation from the boundary failed.

Dispersion relations of electrostatic surface waves propagating in magnetized plasmas contained in an infinite duct and in an infinite cylindrical column surrounded by vacuum are derived by means of a Vlasov equation and fluid equations, respectively. The kinematic boundary condition imposed on the distribution function, the specular reflection conditions on the four sides of a duct, can be satisfied by placing infinite number of fictitious surface charge sheets spaced by the duct widths. The Vlasov equation that includes these surface charge sheets is solved by summing up the contribution due to the infinite charge sheets. The method of placing appropriate fictitious surface charge sheets enables one to treat the surface waves in bounded plasmas of Cartesian structure with mathematical efficiency, kinetically. The kinetic duct dispersion relation is compared with the dispersion relation for the magnetized cylindrical plasma column. When the square duct cross-sectional area as well as the cylinder radius become infinity, both dispersion relations become the dispersion relation of the upper-hybrid wave.

We compare three different approaches to describe a magnetic island in a generic toroidal plasma: (i) perturbative, from the perspective of the equilibrium magnetic field and the related action in a variational principle formulation; (ii) again perturbative, based on the integrability of a system with a single resonant mode and the application of a canonical transformation onto a new island equilibrium system; and (iii) non-perturbative, making use of a full geometric description of the island considered as a stand-alone plasma domain. For the three approaches, we characterize some observables and discuss the respective limits.

Continuum kinetic simulations are increasingly capable of resolving high-dimensional phase space with advances in computing. These capabilities can be more fully explored by using linear kinetic theory to initialize the self-consistent field and phase space perturbations of kinetic instabilities. The phase space perturbation of a kinetic eigenfunction in unmagnetized plasma has a simple analytic form, and in magnetized plasma may be well approximated by truncation of a cyclotron-harmonic expansion. We catalogue the most common use cases with a historical discussion of kinetic eigenfunctions and by conducting nonlinear Vlasov–Poisson and Vlasov–Maxwell simulations of singlemode and multimode two-stream, loss-cone and Weibel instabilities in unmagnetized and magnetized plasmas with one- and two-dimensional geometries. Applications to quasilinear kinetic theory are discussed and applied to the bump-on-tail instability. In order to compute eigenvalues we present novel representations of the dielectric function for ring distributions in magnetized plasmas with power series, hypergeometric and trigonometric integral forms. Eigenfunction phase space fluctuations are visualized for prototypical cases such as the Bernstein modes to build intuition. In addition, phase portraits are presented for the magnetic well associated with nonlinear saturation of the Weibel instability, distinguishing current-density-generating trapping structures from charge-density-generating ones.

The Earth magnetopause, when sufficiently plane and stationary at a local scale, can be considered as a ‘quasi-tangential’ discontinuity, since the normal component of the magnetic field $B_n$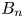 is typically very small but not zero. Contrary to observations, the ‘classic theory of discontinuities’ predicts that rotational and compressional jumps should be mutually exclusive in the general case $B_n \ne 0$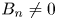, but allows only one exception: the tangential discontinuity provided that $B_n$ 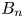is strictly zero. Here we show that finite Larmor radius (FLR) effects play an important role in the quasi-tangential case, whenever the ion Larmor radius is not fully negligible with respect to the magnetopause thickness. By including FLR effects, the results suggest that a rotational discontinuity undergoes a change comparable to the change of a shear Alfvén into a kinetic Alfvén wave when considering linear modes. For this new kind of discontinuity, the co-existence of rotational and compressional variations at the magnetopause does no more imply that this boundary is a strict tangential discontinuity, even in one-dimensional (1-D)-like regions far from X lines if any. This result may lead to important consequences concerning the oldest and most basic questions of magnetospheric physics: how can the magnetopause be open, where and when? While the role of FLR is established theoretically, in this paper we show that it can be proved experimentally. For this, we make use of magnetospheric multiscale mission (MMS) data and process them with the most recent available four spacecraft tools. First, we present the different processing techniques that we use to estimate spatial derivatives, such as $grad(B)$ 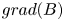and $div(P)$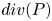, and the magnetopause normal direction. We point out why this normal direction must be determined with extremely high accuracy to make the conclusions unambiguous. Then, the results obtained by these techniques are presented in a detailed case study and on a statistical basis.

A first-order model is derived for quasisymmetric stellarators where the vacuum field due to coils is dominant, but plasma-current-induced terms are not negligible and can contribute to magnetic differential equations, with $\beta$ of the order of the ratio induced to vacuum fields. Under these assumptions, it is proven that the aspect ratio must be large and a simple expression can be obtained for the lowest-order vacuum field. The first-order correction, which involves both vacuum and current-driven fields, is governed by a Grad–Shafranov equation and the requirement that flux surfaces exist. These two equations are not always consistent, and so this model is generally overconstrained, but special solutions exist that satisfy both equations simultaneously. One family of such solutions is the set of first-order near-axis solutions. Thus, the first-order near-axis model is a subset of the model presented here. Several other solutions outside the scope of the near-axis model are also found. A case study comparing one such solution to a VMEC-generated solution shows good agreement.

Physics of the plasma rotation driven by biasing in linear traps is analysed for two limiting cases. The first, relevant for traps with low effective viscosity, considers the line-tying effects to be responsible for the drive as well as for the dissipation of the angular momentum. Meanwhile, in long and thin traps with collisional plasma or developed turbulence, the radial transport of the angular momentum becomes its primary loss channel. The momentum flux goes into the scrape-off layer, which makes conditions there partially responsible for the achievable rotation limits.

We draw attention to an interesting possibility in the design and operation of stellarator fusion reactors, which has hitherto been considered unrealistic under burning-plasma conditions. Thanks to recent advances in stellarator optimisation theory, it appears possible to create a positive (outward-pointing) radial electric field in the plasma core by carefully tailoring the geometry of the magnetic field. This electric field is likely to expel highly charged impurities from the centre of the plasma through neoclassical transport and thus eliminate, or at least mitigate, a long-standing problem in stellarator physics. Further out, the electric field is expected to suddenly change sign from positive to negative, thus creating a region of strongly sheared flow, which could locally suppress turbulent transport and enhance overall energy confinement.

The maser instability associated with the loss-cone distribution has been widely invoked to explain the radio bursts observed in the astrophysical plasma environment, such as aurora and corona. In the laboratory plasma of a tokamak, events reminiscent of these radio bursts have also been frequently observed as an electron cyclotron emission (ECE) burst in the microwave range ($\mathrm{\sim }2{f_{\textrm{ce}}}$ near the last closed flux surface) during transient magnetohydrodynamic events. These bursts have a short duration of ~10 μs and display a radiation spectrum corresponding to a radiation temperature ${T_{e,\textrm{rad}}}$ of over $30\ \textrm{keV}$ while the edge thermal electron temperature ${T_e}$ is only in the range of $1\ \textrm{keV}$. Suprathermal electrons can be generated through magnetic reconnection, and a loss-cone distribution can be generated through open stochastic field lines in the magnetic mirror of the near-edge region of a tokamak plasma. Radiation modelling shows that a sharp distribution gradient $\partial f/\partial {v_ \bot } > 0$ at the loss-cone boundary can cause a negative absorption of ECE radiation through the maser instability. The negative absorption then amplifies the radiation so that the microwave intensity is significantly stronger than the thermal value. The significant ${T_{e,\textrm{rad}}}$ from the simulations suggests the potential role of the loss-cone maser instability in generating the ECE burst in a tokamak.

A collisionless shock structure results from the nonlinear interaction between charged particles and electromagnetic fields. Yet, a collisionless shock is globally governed by the mass, momentum and energy conservation requirements. A stable shock structure must ensure that the fluxes of the conserved quantities are constant on average, and, therefore, is determined by this necessity. Here, we study an observed high upstream temperature high Mach number shock and show that the conservation laws cannot be fulfilled unless the shock is spatially inhomogeneous along the shock front and time-dependent.

We perform experiments in the Large Plasma Device at the University of California, Los Angeles, studying how different end-electrode biasing schemes modify the radial potential profile in the machine. We impose biasing profiles of different polarities and gradient signs on a set of five concentric electrodes placed 12 m downstream from the plasma source. We find that imposing concave-down profiles (negative potential radial gradient) on the electrodes creates radial potential profiles halfway up the plasma column that are comparable to those imposed on the electrodes and a few electron temperature in height, regardless of the biasing polarity. On the other hand, imposing concave-up profiles (positive potential radial gradient) leads to non-monotonic radial potential profiles. This observation can be explained by the current drawn through the electrodes and the parallel plasma resistivity, highlighting their important role in controlling the rotation of plasma. Concave-down plasma potential profiles, obtained by drawing electrons on the axis, are predicted to drive azimuthal drift velocities that can approach significant fractions of the ion sound speed in the central region of the plasma column.

A fusion neutron source (FNS) based on the gas-dynamic trap (GDT, Budker Institute, Novosibirsk) is considered for confinement of two-species plasma heated by neutral beam injection in a regime where the fast ion distribution function is far from Maxwellian. Kinetic instabilities are expected to develop in this regime, and in this paper we investigate the ion-cyclotron instability evolving in moderate densities of pure hydrogen and mixed deuterium–hydrogen target plasmas. The properties of the studied unstable mode, such as its azimuthal wavenumbers, propagation direction and its being affected by changes in the bulk plasma density and composition, allow us to identify it as the drift cyclotron loss cone (DCLC) instability. This mode scatters fast ions and thereby leads to drops in diamagnetic flux signals and increases longitudinal energy and particle losses, with the average energy of the lost ions estimated to be far above the temperature of warm Maxwellian ions. Our interpretation is that the unstable wave grows due to interaction with the fast ions located near the loss cone in the velocity space and scatters them. Applying the method of suppressing the DCLC instability by filling the loss cone with warm plasma, we have determined the values of plasma density and deuterium percentage that allow us to suppress the DCLC instability in the GDT. These findings justify using mixed bulk plasmas in fusion neutron source operation.

Simulating plasma physics on quantum computers is difficult because most problems of interest are nonlinear, but quantum computers are not naturally suitable for nonlinear operations. In weakly nonlinear regimes, plasma problems can be modelled as wave–wave interactions. In this paper, we develop a quantization approach to convert nonlinear wave–wave interaction problems to Hamiltonian simulation problems. We demonstrate our approach using two qubits on a superconducting device. Unlike a photonic device, a superconducting device does not naturally have the desired interactions in its native Hamiltonian. Nevertheless, Hamiltonian simulations can still be performed by decomposing required unitary operations into native gates. To improve experimental results, we employ a range of error-mitigation techniques. Apart from readout error mitigation, we use randomized compilation to transform undiagnosed coherent errors into well-behaved stochastic Pauli channels. Moreover, to compensate for stochastic noise, we rescale exponentially decaying probability amplitudes using rates measured from cycle benchmarking. We carefully consider how different choices of product-formula algorithms affect the overall error and show how a trade-off can be made to best utilize limited quantum resources. This study provides an example of how plasma problems may be solved on near-term quantum computing platforms.

Between a plasma and a solid target lies a positively charged sheath of several Debye lengths $\lambda _{D}$ width, typically much smaller than the characteristic length scale $L$ of the main plasma. This scale separation implies that the asymptotic limit $\epsilon = \lambda _{D} / L \rightarrow 0$ is useful to solve for the plasma-sheath system. In this limit, the Bohm criterion must be satisfied at the sheath entrance. A new derivation of the kinetic criterion, admitting a general ion velocity distribution, is presented. It is proven that, for $\epsilon \rightarrow 0$, the distribution of the velocity component normal to the target, $v_x$, and its first derivative must vanish for $|v_x| \rightarrow 0$ at the sheath entrance. These two conditions can be subsumed into a third integral one after it is integrated by parts twice. A subsequent interchange of the limits $\epsilon \rightarrow 0$ and $|v_x| \rightarrow 0$ is invalid, leading to a divergence which underlies the misconception that the criterion gives undue importance to slow ions.