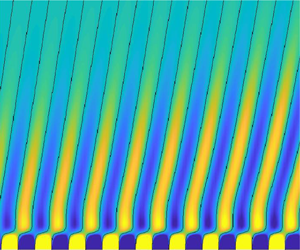
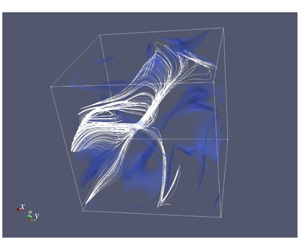
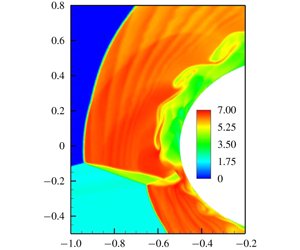
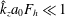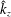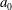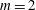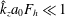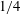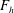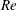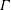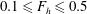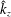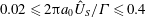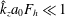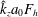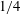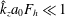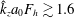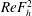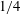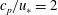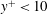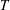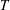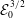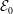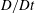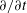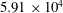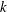In this paper, we investigate the dynamics of an initially vertical vortex embedded in a shear flow in a stratified fluid by means of a long-wavelength analysis. The main goal is to determine, whether or not, the Kelvin–Helmholtz instability can develop unconditionally as speculated by Lilly (J. Atmos. Sci., vol. 40, 1983, pp. 749–761). The analysis is performed in the case of the Lamb–Oseen vortex profile and a shear flow uniform in the horizontal and varying sinusoidally along the vertical using the assumption 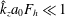 $\hat{k}_{z}a_{0}F_{h}\ll 1$, where
$\hat{k}_{z}a_{0}F_{h}\ll 1$, where 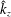 $\hat{k}_{z}$ is the vertical wavenumber,
$\hat{k}_{z}$ is the vertical wavenumber, 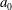 $a_{0}$ the vortex radius and
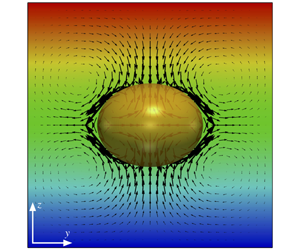
$a_{0}$ the vortex radius and  $F_{h}$ the horizontal Froude number based on the circulation of the vortex. The results show that the vortex axis is advected not only in the direction of the shear flow but also in the perpendicular direction owing to the self-induced motion of the vortex. In addition, internal waves are transiently excited at the beginning, generating an initial non-hydrostatic regime. Their relative effects on the displacements of the vortex axis are weak except initially. The angular velocity of the vortex decays owing to a dynamic effect and viscous effects related to the vertical shear. The former effect is due to the squeezing of isopycnals in the vortex core, which implies a decrease of the vertical vorticity to satisfy potential vorticity conservation. In addition, a horizontal velocity field with an azimuthal wavenumber
$F_{h}$ the horizontal Froude number based on the circulation of the vortex. The results show that the vortex axis is advected not only in the direction of the shear flow but also in the perpendicular direction owing to the self-induced motion of the vortex. In addition, internal waves are transiently excited at the beginning, generating an initial non-hydrostatic regime. Their relative effects on the displacements of the vortex axis are weak except initially. The angular velocity of the vortex decays owing to a dynamic effect and viscous effects related to the vertical shear. The former effect is due to the squeezing of isopycnals in the vortex core, which implies a decrease of the vertical vorticity to satisfy potential vorticity conservation. In addition, a horizontal velocity field with an azimuthal wavenumber 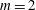 $m=2$ is generated, meaning that the shape of the vortex becomes slightly elliptical. We further show that the minimum asymptotic Richardson number is bounded,
$m=2$ is generated, meaning that the shape of the vortex becomes slightly elliptical. We further show that the minimum asymptotic Richardson number is bounded,  $\min (Ri)>3.43$, when
$\min (Ri)>3.43$, when 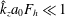 $\hat{k}_{z}a_{0}F_{h}\ll 1$ and therefore cannot go below the critical value
$\hat{k}_{z}a_{0}F_{h}\ll 1$ and therefore cannot go below the critical value 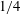 $1/4$. This is because the growth of the vertical shear of the horizontal velocity of the vortex saturates owing to the decay of its angular velocity and because the squeezing of isopycnals increases the stratification strength. This suggests that the shear instability cannot always develop in strongly stratified flows, contrary to the conjecture of Lilly (as above). These predictions will be tested against direct numerical simulations in Part 2.
$1/4$. This is because the growth of the vertical shear of the horizontal velocity of the vortex saturates owing to the decay of its angular velocity and because the squeezing of isopycnals increases the stratification strength. This suggests that the shear instability cannot always develop in strongly stratified flows, contrary to the conjecture of Lilly (as above). These predictions will be tested against direct numerical simulations in Part 2.