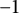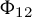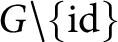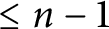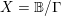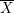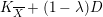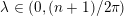6 results
Irregular cusps of orthogonal modular varieties
- Part of
-
- Journal:
- Canadian Journal of Mathematics , First View
- Published online by Cambridge University Press:
- 08 February 2024, pp. 1-31
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
KODAIRA DIMENSION OF UNIVERSAL HOLOMORPHIC SYMPLECTIC VARIETIES
- Part of
-
- Journal:
- Journal of the Institute of Mathematics of Jussieu / Volume 21 / Issue 6 / November 2022
- Published online by Cambridge University Press:
- 02 February 2021, pp. 1849-1866
- Print publication:
- November 2022
-
- Article
-
- You have access
- Open access
- Export citation
Free abelian group actions on normal projective varieties: submaximal dynamical rank case
- Part of
-
- Journal:
- Canadian Journal of Mathematics / Volume 73 / Issue 4 / August 2021
- Published online by Cambridge University Press:
- 14 May 2020, pp. 1057-1073
- Print publication:
- August 2021
-
- Article
- Export citation
SYMBOLIC ANALYTIC SPREAD: UPPER BOUNDS AND APPLICATIONS
- Part of
-
- Journal:
- Journal of the Institute of Mathematics of Jussieu / Volume 20 / Issue 6 / November 2021
- Published online by Cambridge University Press:
- 07 May 2020, pp. 1969-1981
- Print publication:
- November 2021
-
- Article
- Export citation
The Kodaira dimension of complex hyperbolic manifolds with cusps
- Part of
-
- Journal:
- Compositio Mathematica / Volume 154 / Issue 3 / March 2018
- Published online by Cambridge University Press:
- 28 November 2017, pp. 549-564
- Print publication:
- March 2018
-
- Article
- Export citation
The isotriviality of smooth families of canonically polarized manifolds over a special quasi-projective base
- Part of
-
- Journal:
- Compositio Mathematica / Volume 152 / Issue 7 / July 2016
- Published online by Cambridge University Press:
- 26 April 2016, pp. 1421-1434
- Print publication:
- July 2016
-
- Article
- Export citation