We have adapted the Barnes-Hut hierarchical N-body method for cosmological applications. A detailed study of the resulting code yielded the following conclusions: it is SIMPLE, FLEXIBLE, ACCURATE, ROBUST, and EFFICIENT.

In 1981, A. Vilenkin derived the stress tensor for a straight nonconducting cosmic string of linear mass density μ oriented in the z-direction; it is given by Tμν = μdiag(1,0,0,1) δ (x) δ (y). He also showed that in linear approximation the resulting exterior spacetime is flat but conical, with deficit angle 8πGμ. Subsequently it was shown that even the exact spacetime is flat but conical. Recently E. Copeland, M. Hindmarsh, and N. Turok have derived the stress tensor 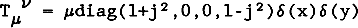for a straight current-carrying string, where j is proportional to the current. We have used this stress tensor, together with the stress tensor of the external electromagnetic field caused by the current, to find the external spacetime of a conducting string in linear approximation. In cylindrical coordinates, the metric may be written where h00 = 4GI2ln2r/r0 + 8Gμj2lnr/r0 = h33 + 8GI2lnr/r0, and a = 1–4Gμ–2GI2. Here r0 is the string radius and I is the electric current, related to j as described in reference 4. The linear approximation is valid as long as h0 0 and h3 3 are small compared with unity, which restricts the range of r. The (r, φ) subspace is flat but conical, with deficit angle 8πG(μ + I2/2). The spacetime reduces to that found by Vilenkin if j and I go to zero. Efforts are currently underway to find an exact exterior solution, which would apply for arbitrary values of r.

The space surrounding a long straight cosmic string is flat but conical. The conical topology implies that such a string focuses light rays or particles passing by opposite sides of the string, which can have important astrophysical effects. The flatness, however, implies that the string has no gravitational influence on matter at rest with respect to the string. The flatness is a consequence of the fact that the tension along a cosmic string is equal to its linear mass density μ. There may be physical effects, however, which destroy the equality of tension and mass density, so that straight strings might after all affect matter at rest. One such effect we and others have calculated is the vacuum fluctuations of fields near the strings induced by the conical topology. Such fluctuation s are physically observable but normally small, as in the Casimir effect between parallel plates. We find the vacuum expectation value of the stress - energy tensor of a conformally coupled scalar field around a cosmic string to be in cylindrical coordinates (t, r, θ, z). The equality of Ttt and Tzz means that the effective tension and mass density of the vacuum fluctuations are equal, so that at least in a semiclassical approximation a string dressed by such fields still has no gravitational influence on matter at rest, even though it has a substantial mass density.

We consider homogeneous, isotropic universe with arbitrary curvature (k=0, ±1), filled with dust, radiation, ˄-term and a set of noninteracting strings (i.e. scaling as ρs ∼ R−2, hence yielding string dominated universe). For such model we find analytic solution of the Friedman equation using Weierstrass functions. We realize that addition of (rather unrealistic component) stable domain walls (ρw ∼ R−1) to the model, does not essentially complicate the calculations.

We have used an N-body code to simulate the collapse of a wake left by a loop of cosmic string in a cold dark matter universe (Ω = 1, h = 0.5). Initial perturbations were introduced via the Zeldovich approximation in a high-resolution sphere of radius 1.5 Mpc (comoving) filled with a lattice of ∼ 104 points masses of 1.38 × 108M⊙. The top plot shows, at z = 24, a wake of a loop of Ms = 3 × 108M⊙ which has moved up and left through the center of the sphere with zeq velocity v = 0.01c directed at an 18° angle out of the plane of the figure. The collapsed object (center) is quite prolate. The outer shell of more massive particles is now also visible. The spherically averaged rotation profile of the “halo” is shown in the bottom diagram. The almost flat portion of the rotation curve corresponds to the size of the wake. There is also evidence for a soft core of ∼ 10 kpc in radius (our dynamical softening was 3 kpc). While the adopted initial velocity and mass are appropriate for loops with galaxy - galaxy separations, a one loop - one object correspondence is not a good approximation on the galactic scale. Moreover, baryons settling in the core of the halo would have steepened the profile and made it incompatible with observations. Halos seeded by several loops may have rotation profiles more compatible with those observed. The results of our simulations may be 10 applied - after an appropriate rescaling - to Abell clusters. There one loop typically seeds a single cluster. String-seeded Abell clusters should be therefore significantly prolate.

Large-scale streaming can be caused by a single “strange attractor” located at a distance D ∼ 45h−1 Mpc from our galaxy (Faber, these proceedings). These observations can be interpreted in terms of a wake of a large loop of cosmic string laid down at z ≤ 1000. The velocity field induced by a stationary loop modeled as a thin spherical shell has a radial dependence v ∼ r−1, consistent with the observed. Zeldovich spectrum of density perturbations would result in v ∼ r−3. The stationary loop model can account for the observed amplitude of the peculiar velocity (v ≃ 500km/s at D ≃ 45h−1 Mpc) only if the dimensionless string tension Gμ/c2 is large, ≳ 7 × 10−6. When peculiar velocities of the loops are taken into account, the estimate of μ can be lowered: The loop is now able to spread its influence. The flow induced by the loop with the physical radius Rs = 36h−1 kpc moving initially with vs = 0.1c and laid down at z=500 is shown in the top portion of the diagram below. The distances are in h−1 Mpc's. The initial comoving loop size and location are indicated by a circle and the present-day location by a dot. Bottom part of the figure shows the line-of-sight peculiar velocities with respect to the microwave background seen by the observer located at the point marked with the square. The loop induces v = 480km/s at ∼ 35h−1 Mpc from the nonlinear part of the diagram - presumed location of the “strange attractor” - providing that its mass is Ms = 9 × μRs, with Gμ/c2 = 5 × 10−6. A loop with Rs ≃ 300h−1 kpc deposited later, at z = 125, would result in v = 520km/s on a scale of 45h−1 Mpc.

If voids like those seen in the low z galaxy distribution existed in the H I distribution at z ≈ 2, then high quality QSO spectra, with many Ly-α forest lines per unit z, could be used to discern the voids from the usual random fluctuations in observed number density of lines (≡ n). Several such spectra have been obtained, and these show evidence for gaps in the Ly-α distribution on scales of 20 to 50 h−1Mpc (comoving coordinates, with h = H0/66.7 km s−1Mpc−1, assuming q0 = 0.1). These results are summarized in the table below. All QSO spectra with a line-of-sight n of Ly-α lines n > 80 per unit z and total number of lines N > 40 known to the author are included (except that of PKS 2000-330 [c.f. Carswell and Rees 1987], which is broken into five separate segments of Ly-α forest by gaps in the data and a broad absorption line). Excluded are portions of these spectra where n falls more than 25% below the mean due to instrumental bias. For each of these the distribution of gaps between nearest-neighbor Ly-α redshifts is computed as a function of gap size. If the distribution of redshifts were Poisson, the distribution of gaps should be a decreasing exponential function of gap size. For the two best spectra, large deviations from an exponential are found in the range of 20 to 50 h−1Mpc (in the other four cases, it should be noted that a large number of gaps of such sizes are still expected from Poisson fluctuations). The probability that such deviations are statistically consistent with an exponential distribution is shown in the fifth column of the table.

We have investigated the redshift distribution of the Ly-α absorption lines in a quite reasonable homogeneous sample compiled by Murdoch et al. (1986).

OSO absorption spectra may offer a clue to the distribution of intervening matter clouds at z = 0 … 3. We tackle 1) the question of the occurrence of metal absorption lines of different ionisation levels in the same redshift systems by investigating the radiation transport through an inhomogeneous temperature profile; 2) the clustering properties of the lines of the Lyα forest by a correlation analysis of 9 published high-resolution QSO-spectra with the result of a certain contribution of metal absorption lines as well as marginally significant positive correlation regions ξ ≈ 0.3.

Using three deep samples of quasars, we have found strong evidence for quasar clustering and its evolution.

We present the results of long slit spectroscopy and deep imaging of the quasar PKS 0812+02 and its environment, obtained with the ESO 3.6m telescope using the ESO Faint Object Spectrograph and Camera (EFOSC) and the Boller and Chivens spectrograph.

This paper summarizes recent results from a study of quasar clustering based on a survey of U.K. Schmidt objective prism plates using the Automatic Plate Measuring facility at Cambridge. New procedures have been developed to detect quasars, eliminate contamination and measure accurate redshifts from the (see Drinkwater, M.J. 1987. Mon. Not. R. astr. soc., submitted). The redshift identifications are 90% reliable with an error (rms) of Δz = ±0.019 for quasars to a limiting magnitude of Bj = 19.5. Some 600 quasars from 6 U.K. Schmidt fields in the redshift range 1.8 < z < 2.6 have been analysed for weak clustering using power spectrum analysis (Webster, A. 1976. Mon. Not. R. astr. soc.175, 61). Plots of the statistic Q′ for 2-D and 3-D (4 fields only) projections of the data are given in the figure below. The ±1σ expectation values of Q′ calaulated for random simulations of the data (including non-uniform redshift selection and plate sensitivity) are indicated by vertical bars.

In recent years much attention has been focussed on the environments of low redshift QSOs. In particular, Yee and Green (1987) have found that the average environment of radio-loud QSOs at z ≃ 0.6, as measured by the QSO-galaxy spatial covarience function, is over three times richer than that of radio-loud QSOs at z ≃ 0.4. This strongly indicates that there has been a steep evolution in the numbers of QSOs in rich clusters over a period of 109 years. This observation is therefore inconsistent with pure luminosity evolution models, which preserves QSO number with epoch, currently employed by a number of authors (see e.g. Boyle et al. 1987) to explain the observed redshift dependence of the QSO luminosity function. However, since over 90% of QSOs are radio-quiet, the main test concerning the validity of pure luminosity evolution is to look for similar evolutionary effects in the preferred environments of optically-selected QSOs.

Quasars at redshifts < 1.0 provide good markers for discovering galaxies and galaxy clusters at moderately high redshifts. Recent results from a comprehensive program of imaging surveys of galaxies in quasar fields are summarized.

Two independent fields of 14′ × 14′ south east of M82 and 7′ × 7′ in SA 57 were completely spectroscopicaly searched for quasars using the Multi Slit Field Spectrograph at the 6-meter telescope of the Academy of Sciences of the USSR (Dodonov, 1982).

We are working on an all-sky sample of radio-selected elliptical galaxies to provide a powerful probe of clustering & streaming velocities on 10–100 Mpc scales. Our eventual sample will have the limits (i) S>0.5 Jy at 1.4 GHz; (ii) 0.01<z<0.1; (iii) |b| >15°; about 400 galaxies satisfy these criteria. We are pursuing an optical programme to obtain (i) B & I CCD frames for all galaxies; (ii) spectra for the galaxies without accurate redshifts; this is now about 30% complete. Accurate optical luminosity indicators exist for radio galaxies, without needing to measure velocity dispersions (using the correlations with optical core radius and radio central-component luminosity: Hoessel 1980: Ap. J. 241, 493; Fabbiano et al. 1984: Ap. J. 277, 115). We therefore expect to provide an accurate test of the Rubin-Ford effect, and to extend such studies to higher redshift. We also have a preliminary result for the 3D two-point correlation function of radio galaxies (see Figure). This strong clustering signal is seen only from galaxies in the decade of radio power below the Fanaroff-Riley division. These objects are known a priori to lie in cluster environments of average Abell richness 0 (Longair & Seldner 1979: MNRAS 189, 433). This result therefore provides confirmation of a trend of clustering with richness independent of optical selection effects in choosing a cluster sample.

Because of their large separation bright radio galaxies characterize the structure of the universe on the scales of clusters and superclusters. In order to calculate the spatial correlation function we choose radio galaxies in radio surveys, for which the redshift values have been measured. One is from Bright Radio Sources at 178 MHz (Liang, Riley and Longair 1983), and another is from All-Sky Catalogue of Bright Extragalactic Radio Sources at 2.7 GHz (Wall and Peacock 1985).

Observational objections against an important role of evolution in morphological differentiation of disk - galaxies (Burnstein 1979, Dressier 1980b, Kent 1985) are summarized together with the results of their det ailed analysis (Salvador-Solé et al. 1987a and 1987b):

1. i) Morphological segregation is independent on cluster characteristics.

The general luminosity function of galaxies is believed to be well represented by the Schechter function with exponent α ≃ −1 and L* ≃ 1010L⊙, but with a wide range of variation from one sample to another. This function decreases exponentially at high luminosities and has a power law behaviour increasing towards low luminosities with no present evidence of turn over or cut-off.

We have investigated the consequences of galaxies in pancakes releasing large quantities of energy, in the spirit of explosion models.