Reviews
Andrzej Grzegorczyk. Zarys logiki matematycznej. Biblioteka matematyczna, vol. 20. Państwowe Wydawnictwo Naukowe, Warsaw1961, 477 pp. - Andrzej Grzegorczyk. Zarys logiki matematycznej. Second, revised and enlarged, edition of the preceding and Polish original of An outline of mathematical logic. Biblioteka matematyczna, vol. 20. Państwowe Wydawnictwo Naukowe, Warsaw1969, 505 pp. - Andrzej Grzegorczyk. Zarys logiki matematycznej. Third revised edition. Biblioteka matematyczna, vol. 20. Państwowe Wydawnictwo Naukowe, Warsaw1973, 510 pp.
-
- Published online by Cambridge University Press:
- 12 March 2014, p. 222
-
- Article
- Export citation
Research Article
Church's thesis without tears
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 797-803
-
- Article
- Export citation
Reviews
Joseph G. Rosenstein. Linear orderings. Pure and applied mathematics, no. 98. Academic Press, New York and London1982, xvii + 487 pp.
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 1207-1209
-
- Article
- Export citation
H. Jerome Keisler. The stability function of a theory. The journal of symbolic logic, vol. 43 (1978), pp. 481–486.
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 496-497
-
- Article
- Export citation
Research Article
The inverse of a regressive object
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 804-815
-
- Article
- Export citation
Reviews
Saharon Shelah. On uniqueness of prime models. The journal of symbolic logic, vol. 44 (1979), pp. 215–220.
-
- Published online by Cambridge University Press:
- 12 March 2014, p. 497
-
- Article
- Export citation
Other
Notices
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 223-224
-
- Article
- Export citation
Meeting Report
Meeting of the Association for Symbolic Logic, Marseilles, 1981 (the Herbrand Symposium)
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 1210-1232
-
- Article
- Export citation
Research Article
Interpreting second-order logic in the monadic theory of order1
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 816-828
-
- Article
- Export citation
Meeting Report
Meeting of the Association for Symbolic Logic, Madison, 1982
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 1233-1239
-
- Article
- Export citation
Front matter
JSL volume 48 issue 1 Cover and Front matter
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. f1-f2
-
- Article
-
- You have access
- Export citation
Reviews
Peter G. Hinman. Recursion-theoretic hierarchies. Perspectives in mathematical logic. Springer-Verlag, Berlin, Heidelberg, and New York, 1978, XII + 480 pp.
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 497-498
-
- Article
- Export citation
Michael R. ΠGarey and David S. Johnson. Computers and intractability. A guide to the theory of NP-completeness. W. H. Freeman and Company, San Francisco1979, x + 338 pp.
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 498-500
-
- Article
- Export citation
Back matter
JSL volume 48 issue 1 Cover and Back matter
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. b1-b2
-
- Article
-
- You have access
- Export citation
Research Article
Kleene index sets and functional m-degrees
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 829-840
-
- Article
- Export citation
Other
Association for Symbolic Logic
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 1240-1272
-
- Article
- Export citation
Reviews
Anil Gupta. The logic of common nouns. An investigation in quantified modal logic. Yale University Press, New Haven and London1980, xi + 142 pp.
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 500-501
-
- Article
- Export citation
Other
Index of Reviews
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 1273-1283
-
- Article
- Export citation
Research Article
On the strength of the Sikorski extension theorem for Boolean algebras
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 841-846
-
- Article
- Export citation
Other
Notices
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 1285-1286
-
- Article
- Export citation

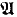 ∈
∈