Research Article
Higher gap morasses, Ia: Gap-two morasses and condensation
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 753-787
-
- Article
- Export citation
Definability and definable groups in simple theories
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 788-796
-
- Article
- Export citation
Σ2 Induction and infinite injury priority argument, Part I: Maximal sets and the jump operator
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 797-814
-
- Article
- Export citation
Automorphisms moving all non-algebraic points and an application to NF
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 815-830
-
- Article
- Export citation
Multiplicative conjunction and an algebraic meaning of contraction and weakening
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 831-859
-
- Article
- Export citation
Forcing on bounded arithmetic II
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 860-868
-
- Article
- Export citation
Completeness of the propositions-as-types interpretation of intuitionistic logic into illative combinatory logic
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 869-890
-
- Article
- Export citation
On automorphism groups of countable structures
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 891-896
-
- Article
- Export citation
Variations on a theme by Weiermann
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 897-925
-
- Article
- Export citation
A note on Lascar strong types in simple theories
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 926-936
-
- Article
- Export citation
The fine structure of real mice
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 937-994
-
- Article
- Export citation
Small fields
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 995-1002
-
- Article
- Export citation
Nowhere precipitousness of some ideals
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 1003-1006
-
- Article
- Export citation
Proof systems for probabilistic uncertain reasoning
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 1007-1039
-
- Article
- Export citation
Ideals over ω and cardinal invariants of the continuum
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 1040-1054
-
- Article
- Export citation
On the existence of strong chains in ℘(ω1)/Fin
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 1055-1062
-
- Article
- Export citation
Completions of PA: Models and enumerations of representable sets
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 1063-1082
-
- Article
- Export citation
Kolmogorov complexity and symmetric relational structures
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 1083-1094
-
- Article
- Export citation
Witnessing functions in bounded arithmetic and search problems
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 1095-1115
-
- Article
- Export citation
Chains of end elementary extensions of models of set theory
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 1116-1136
-
- Article
- Export citation

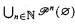 .
.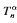 denote a finitely branching, primitive recursive tree of ordinals, i.e., an ordinal as a label is attached to each node in the tree so that the labelling is compatible with the tree ordering. Then the tree
denote a finitely branching, primitive recursive tree of ordinals, i.e., an ordinal as a label is attached to each node in the tree so that the labelling is compatible with the tree ordering. Then the tree 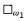 is used to construct a c.c.c forcing that adds a strong chain and Chang's Conjecture to prove that there is no strong chain.
is used to construct a c.c.c forcing that adds a strong chain and Chang's Conjecture to prove that there is no strong chain. -definable with small
-definable with small 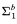 and
and  -definable in the theories
-definable in the theories  and
and 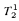 .
.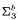 -definable in the theory
-definable in the theory  .
.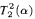 is not
is not 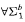 -conservative over the theory
-conservative over the theory 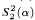 .
.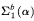 -principle separating the two theories:
-principle separating the two theories: