Research Article
Scales in K(ℝ) at the end of a weak gap
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 369-390
-
- Article
- Export citation
On the consistency strength of the inner model hypothesis
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 391-400
-
- Article
- Export citation
Reals n-generic relative to some perfect tree
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 401-411
-
- Article
- Export citation
Finite state automata and monadic definability of singular cardinals
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 412-438
-
- Article
- Export citation
On metric types that are definable in an o-minimal structure
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 439-447
-
- Article
- Export citation
Special groups whose isometry relation is a finite union of cosets
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 448-473
-
- Article
- Export citation
Coding complete theories in Galois groups
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 474-491
-
- Article
- Export citation
Some pathological examples of precipitous ideals
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 492-511
-
- Article
- Export citation
Internal consistency and global co-stationarity of the ground model
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 512-521
-
- Article
- Export citation
Hierarchies of forcing axioms II
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 522-542
-
- Article
- Export citation
On the structure of the Medvedev lattice
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 543-558
-
- Article
- Export citation
Randomness, lowness and degrees
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 559-577
-
- Article
- Export citation
The polynomial and linear hierarchies in models where the weak pigeonhole principle fails
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 578-592
-
- Article
- Export citation
Complex tilings
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 593-613
-
- Article
- Export citation
Changing the heights of automorphism towers by forcing with Souslin trees over L
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 614-633
-
- Article
- Export citation
How enumeration reductibility yields extended Harrington non-splitting
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 634-655
-
- Article
- Export citation
Generic complexity of undecidable problems
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 656-673
-
- Article
- Export citation
The PCF conjecture and large cardinals
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 674-688
-
- Article
- Export citation
Transfer and a supremum principle for ERNA
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 689-710
-
- Article
- Export citation
Cardinal invariants and the collapse of the continuum by Sacks forcing
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 711-728
-
- Article
- Export citation

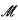
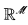 -
-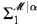
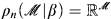 ; then we have that
; then we have that 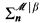 has the Scale Property
has the Scale Property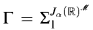 be the pointclass at the beginning of the weak gap referred to in 0.1. In section 1, we use Woodin's ideas to construct a Γ-
be the pointclass at the beginning of the weak gap referred to in 0.1. In section 1, we use Woodin's ideas to construct a Γ-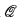 having ω Woodin cardinals cofinal in its ordinals, together with an iteration strategy Σ which
having ω Woodin cardinals cofinal in its ordinals, together with an iteration strategy Σ which 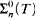 sets
sets 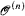 , is not 2-generic relative to any perfect tree and for every ordinal α below the least λ such that sup
, is not 2-generic relative to any perfect tree and for every ordinal α below the least λ such that sup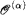 is not 5-generic relative to any perfect tree. Finally, we demonstrate some necessary conditions for reals to be 1-generic relative to some perfect tree.
is not 5-generic relative to any perfect tree. Finally, we demonstrate some necessary conditions for reals to be 1-generic relative to some perfect tree.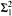
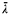 < λ so that
< λ so that 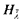 , ψ) is a
, ψ) is a 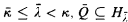 , and π:
, and π: 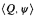 is a
is a 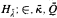 ) into (
) into (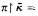 id.
id.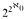 . the size of the lattice itself. We also prove that it is consistent with ZFC that the lattice has chains of size
. the size of the lattice itself. We also prove that it is consistent with ZFC that the lattice has chains of size 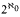 degrees (so that, in particular, there exist
degrees (so that, in particular, there exist  exists in which NP is not in the second level of the linear hierarchy; and that a model of
exists in which NP is not in the second level of the linear hierarchy; and that a model of  definable relations) implies that the strict version of PH does not collapse to a finite level.
definable relations) implies that the strict version of PH does not collapse to a finite level.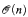 Kolmogorov complexity of its (
Kolmogorov complexity of its ( and we obtain a cardinal invariant
and we obtain a cardinal invariant 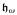 such that
such that 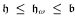 . Applying the Baumgartner-Dordal theorem on preservation of eventually narrow sequences we obtain the consistency of
. Applying the Baumgartner-Dordal theorem on preservation of eventually narrow sequences we obtain the consistency of 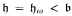 . We define two relations
. We define two relations 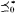 and
and 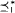 on the set (
on the set (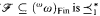 -unbounded, well-ordered by
-unbounded, well-ordered by 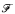 follows from Martin's axiom. This is an analogue of the results of [3], [9, 10] for increasing functions.
follows from Martin's axiom. This is an analogue of the results of [3], [9, 10] for increasing functions.