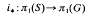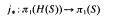Research Article
Inner Bogoliubov automorphisms of the minimal C* Weyl algebra
-
- Published online by Cambridge University Press:
- 18 May 2009, pp. 263-270
-
- Article
-
- You have access
- Export citation
The weak cotype 2 and the Orlicz property of the Lorentz sequence space d(a, 1)
-
- Published online by Cambridge University Press:
- 18 May 2009, pp. 271-276
-
- Article
-
- You have access
- Export citation
Some properties of non-commutative regular graded rings
-
- Published online by Cambridge University Press:
- 18 May 2009, pp. 277-300
-
- Article
-
- You have access
- Export citation
Subalgebras, direct products and associated lattices of MV-algebras
-
- Published online by Cambridge University Press:
- 18 May 2009, pp. 301-307
-
- Article
-
- You have access
- Export citation
An integral formula for compact hypersurfaces in a Euclidean space and its applications
-
- Published online by Cambridge University Press:
- 18 May 2009, pp. 309-311
-
- Article
-
- You have access
- Export citation
Normal closures of powers of Dehn twists in mapping class groups
-
- Published online by Cambridge University Press:
- 18 May 2009, pp. 314-317
-
- Article
-
- You have access
- Export citation
Generalized injectivity and chain conditions
-
- Published online by Cambridge University Press:
- 18 May 2009, pp. 319-326
-
- Article
-
- You have access
- Export citation
A note on p-groups with power automorphisms
-
- Published online by Cambridge University Press:
- 18 May 2009, pp. 327-332
-
- Article
-
- You have access
- Export citation
One sided invertibility and localisation
-
- Published online by Cambridge University Press:
- 18 May 2009, pp. 333-339
-
- Article
-
- You have access
- Export citation
A remarkable identity found in Ramanujan's third notebook
-
- Published online by Cambridge University Press:
- 18 May 2009, pp. 341-345
-
- Article
-
- You have access
- Export citation
On a result of Johnson about Schur multipliers
-
- Published online by Cambridge University Press:
- 18 May 2009, p. 347
-
- Article
-
- You have access
- Export citation
Rings characterized by their weakly-injective modules
-
- Published online by Cambridge University Press:
- 18 May 2009, pp. 349-353
-
- Article
-
- You have access
- Export citation
On the Gauss map of ruled surfaces
-
- Published online by Cambridge University Press:
- 18 May 2009, pp. 355-359
-
- Article
-
- You have access
- Export citation
New characterizations of approximately Gorenstein rings
-
- Published online by Cambridge University Press:
- 18 May 2009, pp. 361-363
-
- Article
-
- You have access
- Export citation
On affine completeness of distributive p-algebras
-
- Published online by Cambridge University Press:
- 18 May 2009, pp. 365-368
-
- Article
-
- You have access
- Export citation
On non-zero values of the central homological dimension of C*-algebras
-
- Published online by Cambridge University Press:
- 18 May 2009, pp. 369-378
-
- Article
-
- You have access
- Export citation
On the fundamental group of a Lie semigroup
-
- Published online by Cambridge University Press:
- 18 May 2009, pp. 379-394
-
- Article
-
- You have access
- Export citation
Front matter
GMJ volume 34 issue 3 Cover and Front matter
-
- Published online by Cambridge University Press:
- 18 May 2009, pp. f1-f8
-
- Article
-
- You have access
- Export citation
Back matter
GMJ volume 34 issue 3 Cover and Back matter
-
- Published online by Cambridge University Press:
- 18 May 2009, pp. b1-b2
-
- Article
-
- You have access
- Export citation

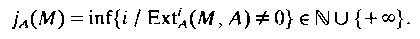
 , where
, where