Research Article
Spaces in Which Special Sets are z-Embedded
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 673-690
-
- Article
-
- You have access
- Export citation
Weak Separation Lattices of Graphs
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 691-724
-
- Article
-
- You have access
- Export citation
Treillis de Séparation des Graphes
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 725-752
-
- Article
-
- You have access
- Export citation
Fourier-Young Coefficients of a Function of Wiener's Class Vp
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 753-759
-
- Article
-
- You have access
- Export citation
Multiplication Ideals, Multiplication Rings, and the Ring R(X)
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 760-768
-
- Article
-
- You have access
- Export citation
Value Distribution of Biaxially Symmetric Harmonic Polynomials
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 769-773
-
- Article
-
- You have access
- Export citation
Stable Extensions and Fields with the Global Density Property
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 774-787
-
- Article
-
- You have access
- Export citation
Local Minimal Overrings
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 788-792
-
- Article
-
- You have access
- Export citation
A General Integral Inequality for the Derivative of an Equimeasurable Rearrangement
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 793-804
-
- Article
-
- You have access
- Export citation
Transitivity Properties of Fuchsian Groups
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 805-814
-
- Article
-
- You have access
- Export citation
Localizations of Hi and Di Rings
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 815-819
-
- Article
-
- You have access
- Export citation
The Ordering of Spec R
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 820-835
-
- Article
-
- You have access
- Export citation
Transformations With Discrete Spectrum are Stacking Transformations
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 836-839
-
- Article
-
- You have access
- Export citation
Nonlinear Oscillation of Fourth Order Differential Equations
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 840-852
-
- Article
-
- You have access
- Export citation
Permutation Problems and Special Functions
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 853-874
-
- Article
-
- You have access
- Export citation
A Minimal Regular Space that is Not Strongly Minimal Regular
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 875-878
-
- Article
-
- You have access
- Export citation
A Combinatorial Interpretation Of The Wreath Product Of Schur Functions
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 879-884
-
- Article
-
- You have access
- Export citation
Holomorphic Functionals on Open Riemann Surfaces
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 885-888
-
- Article
-
- You have access
- Export citation
Purity And Copurity in Systems of Linear Transformations
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 889-896
-
- Article
-
- You have access
- Export citation

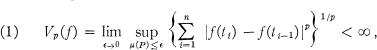
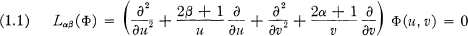


 respectively the separable closure and the algebraic closure of M. If F is a variety which is defined over
respectively the separable closure and the algebraic closure of M. If F is a variety which is defined over 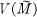 is dense in
is dense in 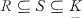 , is called an
, is called an 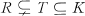 and there are no domains properly between
and there are no domains properly between 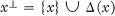 of transformations which can be constructed by the stacking method using single stacks. In particular there is no simple characterization of the class
of transformations which can be constructed by the stacking method using single stacks. In particular there is no simple characterization of the class  .
.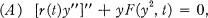
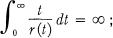
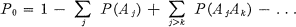
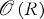 denote the space of functions holomorphic on an open Riemann surface
denote the space of functions holomorphic on an open Riemann surface  *. The result is not new, for it is implicitly contained in the more general results of Tillmann [
*. The result is not new, for it is implicitly contained in the more general results of Tillmann [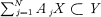 determines a subsystem
determines a subsystem