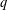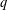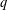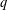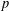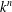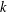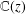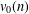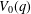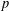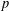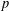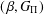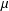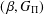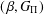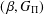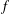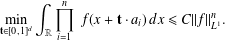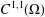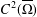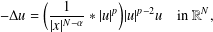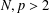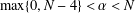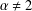Research Article
A TRUNCATED IDENTITY OF EULER AND RELATED
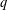 $q$-CONGRUENCES
$q$-CONGRUENCES
- Part of:
-
- Published online by Cambridge University Press:
- 08 April 2020, pp. 353-359
-
- Article
- Export citation
PROOF OF TWO CONJECTURES ON SUPERCONGRUENCES INVOLVING CENTRAL BINOMIAL COEFFICIENTS
- Part of:
-
- Published online by Cambridge University Press:
- 13 February 2020, pp. 360-364
-
- Article
- Export citation
ON SEMIGROUP ORBITS OF POLYNOMIALS AND MULTIPLICATIVE ORDERS
- Part of:
-
- Published online by Cambridge University Press:
- 20 February 2020, pp. 365-373
-
- Article
- Export citation
APPLICATIONS OF SYSTEMS OF QUADRATIC FORMS TO GENERALISED QUADRATIC FORMS
- Part of:
-
- Published online by Cambridge University Press:
- 13 February 2020, pp. 374-386
-
- Article
- Export citation
VANISHING COEFFICIENTS IN QUOTIENTS OF THETA FUNCTIONS OF MODULUS FIVE
- Part of:
-
- Published online by Cambridge University Press:
- 27 March 2020, pp. 387-398
-
- Article
- Export citation
DEGREE-ONE MAHLER FUNCTIONS: ASYMPTOTICS, APPLICATIONS AND SPECULATIONS
- Part of:
-
- Published online by Cambridge University Press:
- 05 February 2020, pp. 399-409
-
- Article
- Export citation
OVERPARTITIONS RELATED TO THE MOCK THETA FUNCTION
 $V_{0}(q)$
$V_{0}(q)$
- Part of:
-
- Published online by Cambridge University Press:
- 16 January 2020, pp. 410-417
-
- Article
- Export citation
POWER PARTITIONS AND SEMI-
 $m$-FIBONACCI PARTITIONS
$m$-FIBONACCI PARTITIONS
- Part of:
-
- Published online by Cambridge University Press:
- 20 February 2020, pp. 418-429
-
- Article
- Export citation
FINITE GROUPS WITH COMPLEMENTED 2-MINIMAL
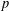 $p$-SUBGROUPS
$p$-SUBGROUPS
- Part of:
-
- Published online by Cambridge University Press:
- 11 March 2020, pp. 430-438
-
- Article
- Export citation
ON
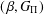 $(\,\unicode[STIX]{x1D6FD},G_{\unicode[STIX]{x1D6F1}})$-UNFAVOURABLE SPACES
$(\,\unicode[STIX]{x1D6FD},G_{\unicode[STIX]{x1D6F1}})$-UNFAVOURABLE SPACES
- Part of:
-
- Published online by Cambridge University Press:
- 16 March 2020, pp. 439-450
-
- Article
- Export citation
EXTENSIONS OF AUTOCORRELATION INEQUALITIES WITH APPLICATIONS TO ADDITIVE COMBINATORICS
- Part of:
-
- Published online by Cambridge University Press:
- 08 April 2020, pp. 451-461
-
- Article
- Export citation
DISTINCT SOLUTIONS TO GENERATED JACOBIAN EQUATIONS CANNOT INTERSECT
- Part of:
-
- Published online by Cambridge University Press:
- 20 February 2020, pp. 462-470
-
- Article
- Export citation
STABLE SOLUTIONS TO THE STATIC CHOQUARD EQUATION
- Part of:
-
- Published online by Cambridge University Press:
- 10 June 2020, pp. 471-478
-
- Article
- Export citation
NOTE ON FOURIER–STIELTJES COEFFICIENTS OF COIN-TOSSING MEASURES
- Part of:
-
- Published online by Cambridge University Press:
- 20 April 2020, pp. 479-489
-
- Article
- Export citation
SOLUTION BRANCHES OF NONLINEAR EIGENVALUE PROBLEMS ON RESTRICTED DOMAINS
- Part of:
-
- Published online by Cambridge University Press:
- 13 March 2020, pp. 490-497
-
- Article
- Export citation
DEFORMING A STARSHAPED CURVE INTO A CIRCLE BY AN AREA-PRESERVING FLOW
- Part of:
-
- Published online by Cambridge University Press:
- 23 April 2020, pp. 498-505
-
- Article
- Export citation
SEQUENTIAL COLLISION-FREE OPTIMAL MOTION PLANNING ALGORITHMS IN PUNCTURED EUCLIDEAN SPACES
- Part of:
-
- Published online by Cambridge University Press:
- 13 March 2020, pp. 506-516
-
- Article
- Export citation
AN IMPROVED LOWER BOUND FOR THE CRITICAL PARAMETER OF STAVSKAYA’S PROCESS
- Part of:
-
- Published online by Cambridge University Press:
- 13 May 2020, pp. 517-524
-
- Article
- Export citation
Front Cover (OFC, IFC) and matter
BAZ volume 102 Issue 3 Cover and Front matter
-
- Published online by Cambridge University Press:
- 19 November 2020, pp. f1-f2
-
- Article
-
- You have access
- Export citation
Back Cover (OBC, IBC) and matter
BAZ volume 102 Issue 3 Cover and Back matter
-
- Published online by Cambridge University Press:
- 19 November 2020, pp. b1-b6
-
- Article
-
- You have access
- Export citation