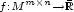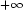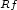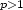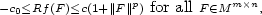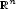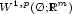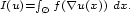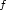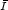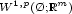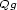In this paper, we consider a Borel
measurable function on
the space of
$\scriptstyle m\times n$ matrices $\scriptstyle f:
M^{m\times n}\rightarrow \bar{\mathbb{R}}$
matrices $\scriptstyle f:
M^{m\times n}\rightarrow \bar{\mathbb{R}}$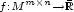 taking the value
$ \scriptstyle +\infty$
taking the value
$ \scriptstyle +\infty$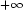 , such that its rank-one-convex
envelope
$\scriptstyle Rf$
, such that its rank-one-convex
envelope
$\scriptstyle Rf$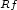 is finite and satisfies for some fixed
$\scriptstyle p>1$
is finite and satisfies for some fixed
$\scriptstyle p>1$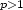 :
$$\scriptstyle -c_0\leq Rf(F)\leq c(1+\Vert F\Vert^p)\
\hbox{for all}\ F\in
M^{m\times n},$$
:
$$\scriptstyle -c_0\leq Rf(F)\leq c(1+\Vert F\Vert^p)\
\hbox{for all}\ F\in
M^{m\times n},$$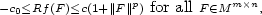 where
$\scriptstyle c,c_0>0$
where
$\scriptstyle c,c_0>0$ . Let $\scriptstyle\O$
. Let $\scriptstyle\O$ be a given
regular bounded
open domain of
$\scriptstyle \mathbb{R}^n$
be a given
regular bounded
open domain of
$\scriptstyle \mathbb{R}^n$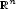 . We define on $\scriptstyle
W^{1,p}(\O;\mathbb{R}^m)$
. We define on $\scriptstyle
W^{1,p}(\O;\mathbb{R}^m)$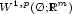 the functional
$$\scriptstyle I(u)=\int_{\O}f(\nabla u(x))\ dx.$$
the functional
$$\scriptstyle I(u)=\int_{\O}f(\nabla u(x))\ dx.$$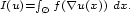 Then, under some technical restrictions on
$\scriptstyle f$
Then, under some technical restrictions on
$\scriptstyle f$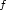 , we show that the relaxed functional
$\scriptstyle\bar I$
, we show that the relaxed functional
$\scriptstyle\bar I$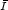 for the weak topology
of
$\scriptstyle W^{1,p}(\O;\mathbb{R}^m)$
for the weak topology
of
$\scriptstyle W^{1,p}(\O;\mathbb{R}^m)$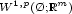 has the integral
representation:
$$\scriptstyle \bar I(u)=\int_{\O}Q[Rf](\nabla u(x))\
dx,$$
has the integral
representation:
$$\scriptstyle \bar I(u)=\int_{\O}Q[Rf](\nabla u(x))\
dx,$$ where for a given function $\scriptstyle g$
where for a given function $\scriptstyle g$ ,
$\scriptstyle Qg$
,
$\scriptstyle Qg$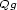 denotes its
quasiconvex envelope.
denotes its
quasiconvex envelope.