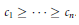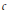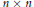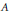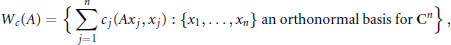Skip to main content Accessibility help

We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.

Close cookie message
×
  1. Home
  2. Search

Refine search

Refine search

Access:
Content type:
Publication date:
Apply   From and To filters
Subject: Show more
Journals Show more
Publishers: Show more
Societies Show more
Series: Show more
Collections: Show more

Actions for selected content:

Select all | Deselect all
  • View selected items
  • Export citations
  • Download PDF (zip)
  • Save to Kindle
  • Save to Dropbox
  • Save to Google Drive

Save Search

You can save your searches here and later view and run them again in "My saved searches".

Please provide a title, maximum of 40 characters.
Cancel
×

5 results

  • 1 - Linear Operators and Matrices

  • from Part I - Numerical Linear Algebra
  • Abner J. Salgado, University of Tennessee, Knoxville, Steven M. Wise, University of Tennessee, Knoxville
  • Book:
    Classical Numerical Analysis
    Published online:
    29 September 2022
    Print publication:
    20 October 2022, pp 3-19
    • Chapter
      • You have access
    • PDF
    • Export citation

  • Summary

    We present several facts about the natural transformations between vector spaces, and their representations via matrices. We introduce induced matrix norms, and the spectral decomposition of nondefective matrices

  • 1 - Semigroups and Generators

  • David Applebaum, University of Sheffield
  • Book:
    Semigroups of Linear Operators
    Published online:
    27 July 2019
    Print publication:
    15 August 2019, pp 9-30
    • Chapter
      • You have access
    • PDF
    • Export citation

  • Summary

    We define semigroups of linear operators in Banach spaces and introduce their generators (which may be unbounded operators) and their resolvents. A number of examples are given, which will be referred back to throughout the volume.

  • Value Distribution and Linear Operators

  • R. Halburd, R. Korhonen
  • Journal:
    Proceedings of the Edinburgh Mathematical Society / Volume 57 / Issue 2 / June 2014
    Published online by Cambridge University Press:
    22 November 2013, pp. 493-504
    • Article
      • You have access
    • PDF
    • Export citation

  • Nevanlinna's second main theorem is a far-reaching generalization of Picard's theorem concerning the value distribution of an arbitrary meromorphic function f. The theorem takes the form of an inequality containing a ramification term in which the zeros and poles of the derivative f′ appear. We show that a similar result holds for special subfields of meromorphic functions where the derivative is replaced by a more general linear operator, such as higher-order differential operators and differential-difference operators. We subsequently derive generalizations of Picard's theorem and the defect relations.

  • Linear Operators Preserving Generalized Numerical Ranges and Radii on Certain Triangular Algebras of Matrices

  • Wai-Shun Cheung, Chi-Kwong Li
  • Journal:
    Canadian Mathematical Bulletin / Volume 44 / Issue 3 / 01 September 2001
    Published online by Cambridge University Press:
    20 November 2018, pp. 270-281
    Print publication:
    01 September 2001
    • Article
      • You have access
    • PDF
    • Export citation

  • Let $c\,=\,\left( {{c}_{1}},\ldots ,{{c}_{n}} \right)$ be such that ${{c}_{1}}\,\ge \,\cdots \,\ge \,{{c}_{n}}$. The $c$-numerical range of an $n\,\times \,n$ matrix $A$ is defined by

    $${{W}_{c}}\left( A \right)\,=\,\left\{ \sum\limits_{j=1}^{n}{{{c}_{j}}\left( A{{x}_{j}},\,{{x}_{j}} \right)\,:\,\left\{ {{x}_{1}},\ldots ,{{x}_{n}} \right\}\,\text{an}\,\text{orthonormal basis for }{{\mathbf{C}}^{n}}} \right\}\,,$$

    and the $c$-numerical radius of $A$ is defined by ${{r}_{c}}\left( A \right)\,=\,\max \left\{ \left| z \right|\,:\,z\,\in \,{{W}_{c}}\left( A \right) \right\}$. We determine the structure of those linear operators $\phi$ on algebras of block triangular matrices, satisfying

    $${{W}_{c}}\left( \phi \left( A \right) \right)={{W}_{c}}\left( A \right)\text{for}\,\,\text{all}\,\,A\,\text{or}\,\,{{r}_{c}}\left( \phi \left( A \right) \right)={{r}_{c}}\left( A \right)\text{for}\,\,\text{all}\,A.$$