We prove a range of new sum-product type growth estimates over a general field 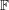 $\mathbb{F}$, in particular the special case
$\mathbb{F}$, in particular the special case 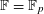 $\mathbb{F}=\mathbb{F}_{p}$. They are unified by the theme of “breaking the
$\mathbb{F}=\mathbb{F}_{p}$. They are unified by the theme of “breaking the 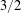 $3/2$ threshold”, epitomizing the previous state of the art. This concerns two questions pivotal for the sum-product theory, which are lower bounds for the number of distinct cross-ratios determined by a finite subset of
$3/2$ threshold”, epitomizing the previous state of the art. This concerns two questions pivotal for the sum-product theory, which are lower bounds for the number of distinct cross-ratios determined by a finite subset of  $\mathbb{F}$, as well as the number of values of the symplectic form determined by a finite subset of
$\mathbb{F}$, as well as the number of values of the symplectic form determined by a finite subset of 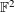 $\mathbb{F}^{2}$. We establish the estimate
$\mathbb{F}^{2}$. We establish the estimate 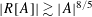 $|R[A]|\gtrsim |A|^{8/5}$ for cardinality of the set
$|R[A]|\gtrsim |A|^{8/5}$ for cardinality of the set 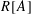 $R[A]$ of distinct cross-ratios, defined by triples of elements of a set
$R[A]$ of distinct cross-ratios, defined by triples of elements of a set 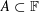 $A\subset \mathbb{F}$ (sufficiently small if
$A\subset \mathbb{F}$ (sufficiently small if 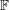 $\mathbb{F}$ has positive characteristic, similarly for the rest of the estimates), pinned at infinity. The cross-ratio bound enables us to break the threshold in the second question: for a non-collinear point set
$\mathbb{F}$ has positive characteristic, similarly for the rest of the estimates), pinned at infinity. The cross-ratio bound enables us to break the threshold in the second question: for a non-collinear point set 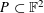 $P\subset \mathbb{F}^{2}$, the number of distinct values of the symplectic form
$P\subset \mathbb{F}^{2}$, the number of distinct values of the symplectic form  $\unicode[STIX]{x1D714}$ on pairs of distinct points
$\unicode[STIX]{x1D714}$ on pairs of distinct points 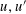 $u,u^{\prime }$ of
$u,u^{\prime }$ of 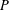 $P$ is
$P$ is  $|\unicode[STIX]{x1D714}(P)|\gtrsim |P|^{2/3+c}$, with an explicit
$|\unicode[STIX]{x1D714}(P)|\gtrsim |P|^{2/3+c}$, with an explicit  $c$. Symmetries of the cross-ratio underlie its local growth properties under both addition and multiplication, yielding an onset of growth of products of difference sets, which is another main result herein. Our proofs make use of specially suited applications of new incidence bounds over
$c$. Symmetries of the cross-ratio underlie its local growth properties under both addition and multiplication, yielding an onset of growth of products of difference sets, which is another main result herein. Our proofs make use of specially suited applications of new incidence bounds over 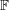 $\mathbb{F}$, which apply to higher moments of representation functions. The technical thrust of the paper uses additive combinatorics to relate and adapt these higher moment bounds to growth estimates. A particular instance of this is breaking the threshold in the few sums, many products question over any
$\mathbb{F}$, which apply to higher moments of representation functions. The technical thrust of the paper uses additive combinatorics to relate and adapt these higher moment bounds to growth estimates. A particular instance of this is breaking the threshold in the few sums, many products question over any 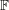 $\mathbb{F}$, by showing that if
$\mathbb{F}$, by showing that if 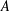 $A$ is sufficiently small and has additive doubling constant
$A$ is sufficiently small and has additive doubling constant 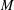 $M$, then
$M$, then  $|AA|\gtrsim M^{-2}|A|^{14/9}$. This result has a second moment version, which allows for new upper bounds for the number of collinear point triples in the set
$|AA|\gtrsim M^{-2}|A|^{14/9}$. This result has a second moment version, which allows for new upper bounds for the number of collinear point triples in the set  $A\times A\subset \mathbb{F}^{2}$, the quantity often arising in applications of geometric incidence estimates.
$A\times A\subset \mathbb{F}^{2}$, the quantity often arising in applications of geometric incidence estimates.
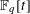 $\mathbb{F}_{q}[t]$
$\mathbb{F}_{q}[t]$
 $A(X\oplus X)$ INTO
$A(X\oplus X)$ INTO 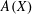 $A(X)$
$A(X)$
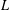 $L$-FUNCTIONS
$L$-FUNCTIONS