Hostname: page-component-cd9895bd7-gxg78 Total loading time: 0 Render date: 2024-12-27T22:49:12.822Z Has data issue: false hasContentIssue false
  • English
  • Français

ON THE DISTRIBUTION OF THE RATIONAL POINTS ON CYCLIC COVERS IN THE ABSENCE OF ROOTS OF UNITY

Part of: Arithmetic algebraic geometry Algebraic number theory: global fields

Published online by Cambridge University Press:  17 April 2019

Lior Bary-Soroker  and
Patrick Meisner
Lior Bary-Soroker
Affiliation:
School of Mathematical Science, Tel Aviv University, Ramat Aviv, Tel Aviv, 6997801, Israel email [email protected]
Patrick Meisner
Affiliation:
School of Mathematical Science, Tel Aviv University, Ramat Aviv, Tel Aviv, 6997801, Israel email [email protected]
Get access

Abstract

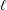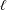In this paper we study the number of rational points on curves in an ensemble of abelian covers of the projective line: let $\ell$ be a prime, $q$ a prime power and consider the ensemble ${\mathcal{H}}_{g,\ell }$ of $\ell$-cyclic covers of $\mathbb{P}_{\mathbb{F}_{q}}^{1}$ of genus 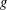$g$. We assume that $q\not \equiv 0,1~\text{mod}~\ell$. If 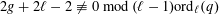$2g+2\ell -2\not \equiv 0~\text{mod}~(\ell -1)\operatorname{ord}_{\ell }(q)$, then 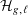${\mathcal{H}}_{g,\ell }$ is empty. Otherwise, the number of rational points on a random curve in ${\mathcal{H}}_{g,\ell }$ distributes as 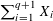$\sum _{i=1}^{q+1}X_{i}$ as $g\rightarrow \infty$, where 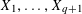$X_{1},\ldots ,X_{q+1}$ are independent and identically distributed random variables taking the values 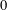$0$ and 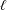$\ell$ with probabilities $(\ell -1)/\ell$ and 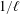$1/\ell$, respectively. The novelty of our result is that it works in the absence of a primitive 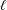$\ell$th root of unity, the presence of which was crucial in previous studies.

MSC classification

Primary: 11G20: Curves over finite and local fields 11R20: Other abelian and metabelian extensions 11R58: Arithmetic theory of algebraic function fields
Type
Research Article
Information
Mathematika , Volume 65 , Issue 3 , 2019 , pp. 719 - 742
Copyright
Copyright © University College London 2019 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Bae, S. and Jung, H., The statistics of the number of rational points in the hyperelliptic ensemble over a finite field. Israel J. Math. 214(1) 2016, 421–442; MR 3540620.Google Scholar
Bucur, A., David, C., Feigon, B., Kaplan, N., Lalín, M., Ozman, E. and Wood, M. M., The distribution of F q -points on cyclic ℓ-covers of genus g . Int. Math. Res. Not. IMRN 2016(14) 2016, 4297–4340; MR 3556420.Google Scholar
Bucur, A., David, C., Feigon, B. and Lalín, M., Fluctuations in the number of points on smooth plane curves over finite fields. J. Number Theory 130(11) 2010, 2528–2541; MR 2678860.Google Scholar
Bucur, A., David, C., Feigon, B. and Lalín, M., Statistics for traces of cyclic trigonal curves over finite fields. Int. Math. Res. Not. IMRN 2010(5) 2010, 932–967; MR 2595014.Google Scholar
Bucur, A., David, C., Feigon, B. and Lalín, M., Biased statistics for traces of cyclic p-fold covers over finite fields. In WIN—Women in Numbers (Fields Institute Communications 60 ), American Mathematical Society (Providence, RI, 2011), 121–143; MR 2777802.Google Scholar
Bucur, A., David, C., Feigon, B. and Lalín, M., Statistics for ordinary Artin–Schreier covers and other p-rank strata. Trans. Amer. Math. Soc. 368(4) 2016, 2371–2413; MR 3449243.Google Scholar
Bucur, A., David, C., Feigon, B., Lalín, M. and Sinha, K., Distribution of zeta zeroes of Artin–Schreier covers. Math. Res. Lett. 19(6) 2012, 1329–1356; MR 3091611.Google Scholar
Cassels, J. W. S. and Fröhlich, A. (eds), Algebraic Number Theory, Academic Press/Thompson Book (London/Washington, DC, 1967).Google Scholar
Cheong, G., Wood, M. M. and Zaman, A., The distribution of points on superelliptic curves over finite fields. Proc. Amer. Math. Soc. 143(4) 2015, 1365–1375; MR 3314052.Google Scholar
Dummit, D. S. and Foote, R. M., Abstract Algebra, Vol. 1984, Wiley (Hoboken, NJ, 2004).Google Scholar
Hess, F. and Massierer, M., Tame class field theory for global function fields. J. Number Theory 162 2016, 86–115.Google Scholar
Kurlberg, P. and Rudnick, Z., The fluctuations in the number of points on a hyperelliptic curve over a finite field. J. Number Theory 129(3) 2009, 580–587; MR 2488590.Google Scholar
Lorenzo, E., Meleleo, G. and Milione, P., Statistics for biquadratic covers of the projective line over finite fields. J. Number Theory 173 2017, 448–477, with an appendix by Alina Bucur;MR 3581928.Google Scholar
Meisner, P., Distribution of points on Abelian covers over finite fields. Int. J. Number Theory 14(5) 2018, 1375–1401.Google Scholar
Meisner, P., Distribution of points on cyclic curves over finite fields. J. Number Theory 177 2017, 528–561; MR 3629255.Google Scholar
Tóth, L., Multiplicative arithmetic functions of several variables: a survey. In Mathematics without Boundaries, Springer (New York, 2014), 483–514; MR 3330713.Google Scholar
Xiong, M., Distribution of zeta zeroes for abelian covers of algebraic curves over a finite field. J. Number Theory 147 2015, 789–823; MR 3276354.Google Scholar