1 Introduction
Shock waves can occur in a fluid whenever the speed of a flow, or of an obstacle moving through it, exceeds the local propagation speed of waves within that fluid. Shocks were first described by Mach & Salcher (Reference Mach and Salcher1887) in the context of a compressible flow of air, where the relevant wave propagation speed is the speed of sound. The ratio of flow speed to acoustic wave speed is known accordingly as the Mach number.
Shock waves of a different sort are found in shallow free-surface flows, where they are called bores or hydraulic jumps. Such a hydraulic jump occurs when thin rapid flow of water down the face of a weir meets the river below and suddenly increases in depth. The relevant wave speed in this flow is the speed of surface gravity waves, and the ratio of flow speed to this wave speed is denoted by the Froude number.
While physically very different, these compression and free-surface shocks arise from the same underlying conservation of mass and momentum across the shock, and can be described by similar systems of hyperbolic conservation laws, namely the compressible Euler equations and the shallow-water equations, respectively.
In granular flows, the speed of acoustic waves is considerably less than that in a homogeneous fluid or solid, and is comparable to the surface gravity wave speed. For example, in the highlighted paper, Khan et al. (Reference Khan, Verma, Hankare, Kumar and Kumar2020) estimate a speed of sound through flowing glass beads of 0.22 m s-1, at least three orders of magnitude slower than the speed of sound in air or glass. Consequently, a free-surface granular avalanche may readily exhibit both compression shocks and free-surface shocks, perhaps simultaneously (Eglit, Kulibaba & Naaim Reference Eglit, Kulibaba and Naaim2007). Both types of shock have been observed in the flow of granular snow avalanches around obstacles, and the design of structures to mitigate the effects of such avalanches (Hákonardóttir et al. Reference Hákonardóttir, Hogg, Jóhannesson and Tómasson2003) has been a significant motivation for the study of free-surface granular shocks (e.g. Gray, Tai & Noelle Reference Gray, Tai and Noelle2003).
The interactions between shocks in a granular flow have received comparatively little study. As in fluids, when two granular compression or free-surface shocks collide, they reflect off one another, sometimes forming a third, stronger shock, known as a Mach stem (Rericha Reference Rericha2004; Vreman et al. Reference Vreman, Al-Tarazi, Kuipers, van Sint Annaland and Bokhove2007; Johnson & Gray Reference Johnson and Gray2011). The highlighted paper by Khan et al. reveals how granular rheology can make these shock interactions significantly more complex.
2 Overview
Khan et al. (Reference Khan, Verma, Hankare, Kumar and Kumar2020) study granular shocks experimentally, using a gravity-driven flow of grains through a thin glass channel, 900 mm long by 310 mm wide, 5 mm deep, and inclined at  $\unicode[STIX]{x1D719}\approx 33^{\circ }{-}80^{\circ }$ to the horizontal. During an experiment, ∼7 kg of 0.125 mm diameter glass beads are released uniformly across the chute width, forming a thin accelerating sheet of granular flow, about 2.5 mm deep and reaching speeds of 1–3 m s-1. At the midpoint of the chute, two triangular obstacles alongside one another, with vertices pointing towards the oncoming flow, obstruct and divert the flowing grains, creating shocks in the granular flow (figure 1a).
$\unicode[STIX]{x1D719}\approx 33^{\circ }{-}80^{\circ }$ to the horizontal. During an experiment, ∼7 kg of 0.125 mm diameter glass beads are released uniformly across the chute width, forming a thin accelerating sheet of granular flow, about 2.5 mm deep and reaching speeds of 1–3 m s-1. At the midpoint of the chute, two triangular obstacles alongside one another, with vertices pointing towards the oncoming flow, obstruct and divert the flowing grains, creating shocks in the granular flow (figure 1a).
Figure 1. Adapted from Khan et al. (Reference Khan, Verma, Hankare, Kumar and Kumar2020). (a) A rapid granular flow (direction indicated by arrows) impinging onto two triangular obstacles. Oblique granular shocks separate undisturbed flow from denser and thicker flow (which appears darker). (b) Shocks from blunter obstacles are detached (i.e. they do not contact the obstacle apex) and collide to form a very dense granular streak. (c–f) The changing morphology of granular shocks and dense streak as the chute inclination angle  $\unicode[STIX]{x1D719}$ is increased from 33° to 80°.
$\unicode[STIX]{x1D719}$ is increased from 33° to 80°.
Across these shocks, the flow rapidly becomes both denser and thicker, thus combining aspects of both classical free-surface and compression shocks. Depending on the shape and spacing of the obstacles, and inclination angle of the chute, which determines the speed of the incoming flow, Khan et al. demonstrate that a wide variety of steady and unsteady shock structures can form.
When the obstacles are sufficiently widely spaced, the flow around each can be treated separately. As in supersonic compressible fluid flows, the flow depends qualitatively on the angle at the apex of each obstacle. When this angle is small, oblique shocks form at this apex (figure 1a), whereas for larger angles the shock is detached from the obstacle, forming some distance upstream of the apex (figure 1b).
When the chute inclination angle  $\unicode[STIX]{x1D719}$ is small (figure 1c), a region of slowly moving densely packed grains collects around the apex of each obstacle (Amarouchene, Boudet & Kellay Reference Amarouchene, Boudet and Kellay2001). The focus of Khan et al.’s work, however, is the flow in between two relatively closely spaced obstacles, where the shocks and associated thicker stream of grains diverted from each obstacle collide. The collision of these two streams forms a region of very dense, near-incompressible granular flow (with grain volume fraction close to the maximum ≈0.6) that is in frictional contact with both the lower and upper surfaces of the chute. The energy loss associated with the inelastic collision of grains in this region promotes the formation of a persistent dense cluster, within an otherwise energetic granular flow (Kudrolli, Wolpert & Gollub Reference Kudrolli, Wolpert and Gollub1997). Grains at the top of this dense region rest on those by those below, forming a granular pile (which is likely further supported by friction with the chute walls, as in Taberlet et al. (Reference Taberlet, Richard, Valance, Losert, Pasini, Jenkins and Delannay2003)) but grains at the very bottom of the dense region fall under gravity to form a dense granular streak. As the inclination angle of the chute is increased (figure 1c–f), this shock interaction region becomes the densest and slowest-moving part of the flow. The presence of such a dense, liquid-like region, suspended away from the obstacle by dilute gas-like flow, is reminiscent of the so-called granular Leidenfrost effect (Eshuis et al. Reference Eshuis, van der Weele, van der Meer and Lohse2005), in which a dense granular flow is suspended above a vibrating boundary by energetic grains in a dilute gas-like state.
$\unicode[STIX]{x1D719}$ is small (figure 1c), a region of slowly moving densely packed grains collects around the apex of each obstacle (Amarouchene, Boudet & Kellay Reference Amarouchene, Boudet and Kellay2001). The focus of Khan et al.’s work, however, is the flow in between two relatively closely spaced obstacles, where the shocks and associated thicker stream of grains diverted from each obstacle collide. The collision of these two streams forms a region of very dense, near-incompressible granular flow (with grain volume fraction close to the maximum ≈0.6) that is in frictional contact with both the lower and upper surfaces of the chute. The energy loss associated with the inelastic collision of grains in this region promotes the formation of a persistent dense cluster, within an otherwise energetic granular flow (Kudrolli, Wolpert & Gollub Reference Kudrolli, Wolpert and Gollub1997). Grains at the top of this dense region rest on those by those below, forming a granular pile (which is likely further supported by friction with the chute walls, as in Taberlet et al. (Reference Taberlet, Richard, Valance, Losert, Pasini, Jenkins and Delannay2003)) but grains at the very bottom of the dense region fall under gravity to form a dense granular streak. As the inclination angle of the chute is increased (figure 1c–f), this shock interaction region becomes the densest and slowest-moving part of the flow. The presence of such a dense, liquid-like region, suspended away from the obstacle by dilute gas-like flow, is reminiscent of the so-called granular Leidenfrost effect (Eshuis et al. Reference Eshuis, van der Weele, van der Meer and Lohse2005), in which a dense granular flow is suspended above a vibrating boundary by energetic grains in a dilute gas-like state.
As the inclination angle  $\unicode[STIX]{x1D719}$ increases, the upstream edge of this dense region of grains changes in shape from pointed (figure 1c,d) to a blunt, flat-topped shape (figure 1e,f). For parameters close to the transition between these two regimes (figure 1e), Khan et al. (Reference Khan, Verma, Hankare, Kumar and Kumar2020) observe that the dense collision region oscillates from side to side, bouncing between the two obstacles with a frequency of approximately 3 Hz. This instability leaves oscillations in the dense streak of grains downstream.
$\unicode[STIX]{x1D719}$ increases, the upstream edge of this dense region of grains changes in shape from pointed (figure 1c,d) to a blunt, flat-topped shape (figure 1e,f). For parameters close to the transition between these two regimes (figure 1e), Khan et al. (Reference Khan, Verma, Hankare, Kumar and Kumar2020) observe that the dense collision region oscillates from side to side, bouncing between the two obstacles with a frequency of approximately 3 Hz. This instability leaves oscillations in the dense streak of grains downstream.
3 Future
The granular flows observed by Khan et al. (Reference Khan, Verma, Hankare, Kumar and Kumar2020) present a significant challenge for theoretical continuum models for granular flow, exhibiting both dilute and dense regions of flow, a strong influence of boundaries, and flow features potentially comparable in size to the mean free path of grains. Some insight into the role of granular rheology in these flows can be gained by comparing the experimental results with solutions of possibly the simplest analogous shock-forming system, the non-dimensional shallow-water equations (Tan Reference Tan1992). These hyperbolic equations express the conservation of mass,  $\unicode[STIX]{x2202}_{t}h+\unicode[STIX]{x1D735}\boldsymbol{\cdot }(h\bar{\boldsymbol{u}})=0$, and the conservation of momentum,
$\unicode[STIX]{x2202}_{t}h+\unicode[STIX]{x1D735}\boldsymbol{\cdot }(h\bar{\boldsymbol{u}})=0$, and the conservation of momentum,  $\unicode[STIX]{x2202}_{t}(h\bar{\boldsymbol{u}})+\unicode[STIX]{x1D735}\boldsymbol{\cdot }(h\bar{\boldsymbol{u}}\bar{\boldsymbol{u}}+\unicode[STIX]{x1D644}h^{2}/2)=0$, of a thin layer of inviscid, incompressible fluid, of thickness
$\unicode[STIX]{x2202}_{t}(h\bar{\boldsymbol{u}})+\unicode[STIX]{x1D735}\boldsymbol{\cdot }(h\bar{\boldsymbol{u}}\bar{\boldsymbol{u}}+\unicode[STIX]{x1D644}h^{2}/2)=0$, of a thin layer of inviscid, incompressible fluid, of thickness  $h$ and depth-averaged (two-dimensional) velocity
$h$ and depth-averaged (two-dimensional) velocity  $\bar{\boldsymbol{u}}$. Granular physics is entirely neglected in these equations, yet numerical solutions of flows past triangular obstacles (figure 2) show some qualitatively similar shock interactions to those observed by Khan et al., illustrating the fundamental importance of mass and momentum conservation in determining these structures. But the simple shallow-water model fails, of course, to capture much of the complexity of Khan et al.’s flows, including large density variations, oscillatory shocks and the ‘indent’ in the detached shock near the apex of each obstacle at high inclinations (figure 1f), which must be attributed to specifics of granular compression and shear rheology. A unified theoretical description of these observations, and more generally of granular flows with both gas-like and liquid-like regions, remains an important challenge for the granular modelling community.
$\bar{\boldsymbol{u}}$. Granular physics is entirely neglected in these equations, yet numerical solutions of flows past triangular obstacles (figure 2) show some qualitatively similar shock interactions to those observed by Khan et al., illustrating the fundamental importance of mass and momentum conservation in determining these structures. But the simple shallow-water model fails, of course, to capture much of the complexity of Khan et al.’s flows, including large density variations, oscillatory shocks and the ‘indent’ in the detached shock near the apex of each obstacle at high inclinations (figure 1f), which must be attributed to specifics of granular compression and shear rheology. A unified theoretical description of these observations, and more generally of granular flows with both gas-like and liquid-like regions, remains an important challenge for the granular modelling community.
Figure 2. Steady numerical solutions of the shallow-water equations for uniform flow past triangular obstacles, showing flow thickness (shading) and streamlines. Discontinuities in thickness indicate the formation of shocks. The shock structures depend strongly on the inflow Froude number  $Fr=|\bar{\boldsymbol{u}}|/\sqrt{h}$, and are reminiscent of several regimes observed by Khan et al. (Reference Khan, Verma, Hankare, Kumar and Kumar2020).
$Fr=|\bar{\boldsymbol{u}}|/\sqrt{h}$, and are reminiscent of several regimes observed by Khan et al. (Reference Khan, Verma, Hankare, Kumar and Kumar2020).
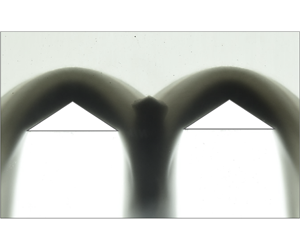

 $\unicode[STIX]{x1D719}$ is increased from 33° to 80°.
$\unicode[STIX]{x1D719}$ is increased from 33° to 80°.
 $Fr=|\bar{\boldsymbol{u}}|/\sqrt{h}$, and are reminiscent of several regimes observed by Khan et al. (2020).
$Fr=|\bar{\boldsymbol{u}}|/\sqrt{h}$, and are reminiscent of several regimes observed by Khan et al. (2020).
1 Introduction
Shock waves can occur in a fluid whenever the speed of a flow, or of an obstacle moving through it, exceeds the local propagation speed of waves within that fluid. Shocks were first described by Mach & Salcher (Reference Mach and Salcher1887) in the context of a compressible flow of air, where the relevant wave propagation speed is the speed of sound. The ratio of flow speed to acoustic wave speed is known accordingly as the Mach number.
Shock waves of a different sort are found in shallow free-surface flows, where they are called bores or hydraulic jumps. Such a hydraulic jump occurs when thin rapid flow of water down the face of a weir meets the river below and suddenly increases in depth. The relevant wave speed in this flow is the speed of surface gravity waves, and the ratio of flow speed to this wave speed is denoted by the Froude number.
While physically very different, these compression and free-surface shocks arise from the same underlying conservation of mass and momentum across the shock, and can be described by similar systems of hyperbolic conservation laws, namely the compressible Euler equations and the shallow-water equations, respectively.
In granular flows, the speed of acoustic waves is considerably less than that in a homogeneous fluid or solid, and is comparable to the surface gravity wave speed. For example, in the highlighted paper, Khan et al. (Reference Khan, Verma, Hankare, Kumar and Kumar2020) estimate a speed of sound through flowing glass beads of 0.22 m s-1, at least three orders of magnitude slower than the speed of sound in air or glass. Consequently, a free-surface granular avalanche may readily exhibit both compression shocks and free-surface shocks, perhaps simultaneously (Eglit, Kulibaba & Naaim Reference Eglit, Kulibaba and Naaim2007). Both types of shock have been observed in the flow of granular snow avalanches around obstacles, and the design of structures to mitigate the effects of such avalanches (Hákonardóttir et al. Reference Hákonardóttir, Hogg, Jóhannesson and Tómasson2003) has been a significant motivation for the study of free-surface granular shocks (e.g. Gray, Tai & Noelle Reference Gray, Tai and Noelle2003).
The interactions between shocks in a granular flow have received comparatively little study. As in fluids, when two granular compression or free-surface shocks collide, they reflect off one another, sometimes forming a third, stronger shock, known as a Mach stem (Rericha Reference Rericha2004; Vreman et al. Reference Vreman, Al-Tarazi, Kuipers, van Sint Annaland and Bokhove2007; Johnson & Gray Reference Johnson and Gray2011). The highlighted paper by Khan et al. reveals how granular rheology can make these shock interactions significantly more complex.
2 Overview
Khan et al. (Reference Khan, Verma, Hankare, Kumar and Kumar2020) study granular shocks experimentally, using a gravity-driven flow of grains through a thin glass channel, 900 mm long by 310 mm wide, 5 mm deep, and inclined at $\unicode[STIX]{x1D719}\approx 33^{\circ }{-}80^{\circ }$ to the horizontal. During an experiment, ∼7 kg of 0.125 mm diameter glass beads are released uniformly across the chute width, forming a thin accelerating sheet of granular flow, about 2.5 mm deep and reaching speeds of 1–3 m s-1. At the midpoint of the chute, two triangular obstacles alongside one another, with vertices pointing towards the oncoming flow, obstruct and divert the flowing grains, creating shocks in the granular flow (figure 1a).
$\unicode[STIX]{x1D719}\approx 33^{\circ }{-}80^{\circ }$ to the horizontal. During an experiment, ∼7 kg of 0.125 mm diameter glass beads are released uniformly across the chute width, forming a thin accelerating sheet of granular flow, about 2.5 mm deep and reaching speeds of 1–3 m s-1. At the midpoint of the chute, two triangular obstacles alongside one another, with vertices pointing towards the oncoming flow, obstruct and divert the flowing grains, creating shocks in the granular flow (figure 1a).
Figure 1. Adapted from Khan et al. (Reference Khan, Verma, Hankare, Kumar and Kumar2020). (a) A rapid granular flow (direction indicated by arrows) impinging onto two triangular obstacles. Oblique granular shocks separate undisturbed flow from denser and thicker flow (which appears darker). (b) Shocks from blunter obstacles are detached (i.e. they do not contact the obstacle apex) and collide to form a very dense granular streak. (c–f) The changing morphology of granular shocks and dense streak as the chute inclination angle $\unicode[STIX]{x1D719}$ is increased from 33° to 80°.
$\unicode[STIX]{x1D719}$ is increased from 33° to 80°.
Across these shocks, the flow rapidly becomes both denser and thicker, thus combining aspects of both classical free-surface and compression shocks. Depending on the shape and spacing of the obstacles, and inclination angle of the chute, which determines the speed of the incoming flow, Khan et al. demonstrate that a wide variety of steady and unsteady shock structures can form.
When the obstacles are sufficiently widely spaced, the flow around each can be treated separately. As in supersonic compressible fluid flows, the flow depends qualitatively on the angle at the apex of each obstacle. When this angle is small, oblique shocks form at this apex (figure 1a), whereas for larger angles the shock is detached from the obstacle, forming some distance upstream of the apex (figure 1b).
When the chute inclination angle $\unicode[STIX]{x1D719}$ is small (figure 1c), a region of slowly moving densely packed grains collects around the apex of each obstacle (Amarouchene, Boudet & Kellay Reference Amarouchene, Boudet and Kellay2001). The focus of Khan et al.’s work, however, is the flow in between two relatively closely spaced obstacles, where the shocks and associated thicker stream of grains diverted from each obstacle collide. The collision of these two streams forms a region of very dense, near-incompressible granular flow (with grain volume fraction close to the maximum ≈0.6) that is in frictional contact with both the lower and upper surfaces of the chute. The energy loss associated with the inelastic collision of grains in this region promotes the formation of a persistent dense cluster, within an otherwise energetic granular flow (Kudrolli, Wolpert & Gollub Reference Kudrolli, Wolpert and Gollub1997). Grains at the top of this dense region rest on those by those below, forming a granular pile (which is likely further supported by friction with the chute walls, as in Taberlet et al. (Reference Taberlet, Richard, Valance, Losert, Pasini, Jenkins and Delannay2003)) but grains at the very bottom of the dense region fall under gravity to form a dense granular streak. As the inclination angle of the chute is increased (figure 1c–f), this shock interaction region becomes the densest and slowest-moving part of the flow. The presence of such a dense, liquid-like region, suspended away from the obstacle by dilute gas-like flow, is reminiscent of the so-called granular Leidenfrost effect (Eshuis et al. Reference Eshuis, van der Weele, van der Meer and Lohse2005), in which a dense granular flow is suspended above a vibrating boundary by energetic grains in a dilute gas-like state.
$\unicode[STIX]{x1D719}$ is small (figure 1c), a region of slowly moving densely packed grains collects around the apex of each obstacle (Amarouchene, Boudet & Kellay Reference Amarouchene, Boudet and Kellay2001). The focus of Khan et al.’s work, however, is the flow in between two relatively closely spaced obstacles, where the shocks and associated thicker stream of grains diverted from each obstacle collide. The collision of these two streams forms a region of very dense, near-incompressible granular flow (with grain volume fraction close to the maximum ≈0.6) that is in frictional contact with both the lower and upper surfaces of the chute. The energy loss associated with the inelastic collision of grains in this region promotes the formation of a persistent dense cluster, within an otherwise energetic granular flow (Kudrolli, Wolpert & Gollub Reference Kudrolli, Wolpert and Gollub1997). Grains at the top of this dense region rest on those by those below, forming a granular pile (which is likely further supported by friction with the chute walls, as in Taberlet et al. (Reference Taberlet, Richard, Valance, Losert, Pasini, Jenkins and Delannay2003)) but grains at the very bottom of the dense region fall under gravity to form a dense granular streak. As the inclination angle of the chute is increased (figure 1c–f), this shock interaction region becomes the densest and slowest-moving part of the flow. The presence of such a dense, liquid-like region, suspended away from the obstacle by dilute gas-like flow, is reminiscent of the so-called granular Leidenfrost effect (Eshuis et al. Reference Eshuis, van der Weele, van der Meer and Lohse2005), in which a dense granular flow is suspended above a vibrating boundary by energetic grains in a dilute gas-like state.
As the inclination angle $\unicode[STIX]{x1D719}$ increases, the upstream edge of this dense region of grains changes in shape from pointed (figure 1c,d) to a blunt, flat-topped shape (figure 1e,f). For parameters close to the transition between these two regimes (figure 1e), Khan et al. (Reference Khan, Verma, Hankare, Kumar and Kumar2020) observe that the dense collision region oscillates from side to side, bouncing between the two obstacles with a frequency of approximately 3 Hz. This instability leaves oscillations in the dense streak of grains downstream.
$\unicode[STIX]{x1D719}$ increases, the upstream edge of this dense region of grains changes in shape from pointed (figure 1c,d) to a blunt, flat-topped shape (figure 1e,f). For parameters close to the transition between these two regimes (figure 1e), Khan et al. (Reference Khan, Verma, Hankare, Kumar and Kumar2020) observe that the dense collision region oscillates from side to side, bouncing between the two obstacles with a frequency of approximately 3 Hz. This instability leaves oscillations in the dense streak of grains downstream.
3 Future
The granular flows observed by Khan et al. (Reference Khan, Verma, Hankare, Kumar and Kumar2020) present a significant challenge for theoretical continuum models for granular flow, exhibiting both dilute and dense regions of flow, a strong influence of boundaries, and flow features potentially comparable in size to the mean free path of grains. Some insight into the role of granular rheology in these flows can be gained by comparing the experimental results with solutions of possibly the simplest analogous shock-forming system, the non-dimensional shallow-water equations (Tan Reference Tan1992). These hyperbolic equations express the conservation of mass, $\unicode[STIX]{x2202}_{t}h+\unicode[STIX]{x1D735}\boldsymbol{\cdot }(h\bar{\boldsymbol{u}})=0$, and the conservation of momentum,
$\unicode[STIX]{x2202}_{t}h+\unicode[STIX]{x1D735}\boldsymbol{\cdot }(h\bar{\boldsymbol{u}})=0$, and the conservation of momentum,  $\unicode[STIX]{x2202}_{t}(h\bar{\boldsymbol{u}})+\unicode[STIX]{x1D735}\boldsymbol{\cdot }(h\bar{\boldsymbol{u}}\bar{\boldsymbol{u}}+\unicode[STIX]{x1D644}h^{2}/2)=0$, of a thin layer of inviscid, incompressible fluid, of thickness
$\unicode[STIX]{x2202}_{t}(h\bar{\boldsymbol{u}})+\unicode[STIX]{x1D735}\boldsymbol{\cdot }(h\bar{\boldsymbol{u}}\bar{\boldsymbol{u}}+\unicode[STIX]{x1D644}h^{2}/2)=0$, of a thin layer of inviscid, incompressible fluid, of thickness  $h$ and depth-averaged (two-dimensional) velocity
$h$ and depth-averaged (two-dimensional) velocity  $\bar{\boldsymbol{u}}$. Granular physics is entirely neglected in these equations, yet numerical solutions of flows past triangular obstacles (figure 2) show some qualitatively similar shock interactions to those observed by Khan et al., illustrating the fundamental importance of mass and momentum conservation in determining these structures. But the simple shallow-water model fails, of course, to capture much of the complexity of Khan et al.’s flows, including large density variations, oscillatory shocks and the ‘indent’ in the detached shock near the apex of each obstacle at high inclinations (figure 1f), which must be attributed to specifics of granular compression and shear rheology. A unified theoretical description of these observations, and more generally of granular flows with both gas-like and liquid-like regions, remains an important challenge for the granular modelling community.
$\bar{\boldsymbol{u}}$. Granular physics is entirely neglected in these equations, yet numerical solutions of flows past triangular obstacles (figure 2) show some qualitatively similar shock interactions to those observed by Khan et al., illustrating the fundamental importance of mass and momentum conservation in determining these structures. But the simple shallow-water model fails, of course, to capture much of the complexity of Khan et al.’s flows, including large density variations, oscillatory shocks and the ‘indent’ in the detached shock near the apex of each obstacle at high inclinations (figure 1f), which must be attributed to specifics of granular compression and shear rheology. A unified theoretical description of these observations, and more generally of granular flows with both gas-like and liquid-like regions, remains an important challenge for the granular modelling community.
Figure 2. Steady numerical solutions of the shallow-water equations for uniform flow past triangular obstacles, showing flow thickness (shading) and streamlines. Discontinuities in thickness indicate the formation of shocks. The shock structures depend strongly on the inflow Froude number $Fr=|\bar{\boldsymbol{u}}|/\sqrt{h}$, and are reminiscent of several regimes observed by Khan et al. (Reference Khan, Verma, Hankare, Kumar and Kumar2020).
$Fr=|\bar{\boldsymbol{u}}|/\sqrt{h}$, and are reminiscent of several regimes observed by Khan et al. (Reference Khan, Verma, Hankare, Kumar and Kumar2020).
Declaration of interests
The author reports no conflict of interest.