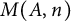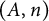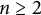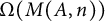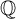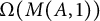On homotopy nilpotency of loop spaces of Moore spaces

Abstract

Let $M(A,n)$ be the Moore space of type $(A,n)$ for an Abelian group A and $n\ge 2$ . We show that the loop space $\Omega (M(A,n))$ is homotopy nilpotent if and only if A is a subgroup of the additive group $\mathbb {Q}$ of the field of rationals. Homotopy nilpotency of loop spaces $\Omega (M(A,1))$ is discussed as well.

1 Introduction

In group theory, if we consider only nilpotent groups, the nilpotency class is the one which measures a distance from commutativity. Already Whitehead [21] had the insight that the (J.H.C.) Whitehead products satisfy identities which reflect commutator identities for groups. Berstein and Ganea [3] adapted the nilpotency to H-spaces as follows. Let X be an H-space, $\varphi _{X,1}=\text {id}_X$ and $\varphi _{X,2} : X^2\to X$ the commutator map. Put $\varphi _{X,n+1} = \varphi _{X,1}\circ (\text {id}_X \times \varphi _{X,n})$ for $(n+1)$ -fold commutator map of X with $n\ge 2$ . An H-space X is called homotopy nilpotent of class n if $\varphi _{X,n+1}\simeq \ast $ , is null homotopic but $\varphi _{X,n}$ is not [3]. In this case, we write $\text {nil}\,X = n$ .

Then, Berstein and Ganea [3] introduced a concept of the homotopy nilpotency of a pointed space by means of its loop space. In particular, the m-iterated Samelson products vanish in the loop space $\Omega (X)$ , or equivalently, the m-iterated Whitehead products vanish in X provided $m>\text {nil}\,\Omega (X)$ . We refer to [22, Chapter X] for details on Samelson and Whitehead products.

The homotopy nilpotency classes $\text {nil}\,X$ of associative H-spaces X has been extensively studied as well as their homotopy commutativity. Work of Hopkins [12] drew renewed attention to such problems by relating this classical nilpotency notion with the nilpotence theorem of Devinatz et al. [5]. In particular, Hopkins [12] made substantial progress by giving cohomological criteria for homotopy associative finite H-spaces to be homotopy nilpotent. For example, he showed that if a homotopy associative finite H-space has no torsion in the integral homology, then it is homotopy nilpotent. Later, Rao [16] showed that the converse of the above criterion is true in the case of groups $\text {Spin}(n)$ and $SO(n)$ . Eventually, Yagita [23] proved that, when G is a compact, simply connected Lie group, its p-localization $G_{(p)}$ is homotopy nilpotent if and only if G has no torsion in the integral homology. Finally, Rao [17] showed that a connected compact Lie group is homotopy nilpotent if and only if it has no torsion in homology.

Although many results on the homotopy nilpotency have been obtained, the homotopy nilpotency classes have been determined in very few cases. It is well-known that for the loop space $\Omega (\mathbb {S}^m)$ of the m-sphere $\mathbb {S}^m$ , we have $\text {nil}\,\Omega (\mathbb {S}^m)=1$ if and only if $m=1,3,7$ and

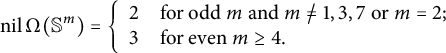$$ \begin{align*} \mathrm{nil}\,\Omega(\mathbb{S}^m) = \left\{\begin{array}{ll} 2&\text{for odd } m \text{ and } m\not=1,3,7 \text{ or } m = 2;\\ 3&\text{for even } m\ge 4. \end{array}\right. \end{align*} $$

Write $\mathbb {K}P^m$ for the projective m-space for $\mathbb {K}=\mathbb {R},\,\mathbb {C}$ , the field of reals or complex numbers and $\mathbb {H}$ , the skew $\mathbb {R}$ -algebra of quaternions. Then, the homotopy nilpotency of $\Omega (\mathbb {K}P^m)$ has been first studied by Ganea [8], Snaith [19], and then their p-localization $\Omega ((\mathbb {K}P^m)_{(p)})$ by Meier [13]. The homotopy nilpotency of the loop spaces of Grassmann and Stiefel manifolds, and their p-localization have been extensively studied in [9].

Let $\mathbb {S}^{2m-1}_{(p)}$ be the p-localization of the sphere $\mathbb {S}^{2m-1}$ at a prime p. The main result of the paper [10] is the explicit determination of the homotopy nilpotence class of a wide range of homotopy associative multiplications on localized spheres $\mathbb {S}^{2m-1}_{(p)}$ for $p>3$ .

The paper grew out of our desire to develop techniques in the study of the homotopy nilpotency classes $\text {nil}\,\Omega (M(A,n))$ for Moore spaces $M(A,n)$ with $n\ge 1$ . In Section 1, we set stages for developments to come. This introductory section is devoted to a general discussion and establishes notations on the homotopy nilpotency of H-spaces used in the rest of the paper.

Section 2, takes up the systematic study of the homotopy nilpotency of $\Omega (M(A,n))$ for $n\ge 1$ . First, given a space X, we consider the iterated Samelson product $s_k : X^{\wedge k}\longrightarrow \Omega \Sigma (X)$ to show in Proposition 3.2 that the space $\Omega \Sigma (X)$ is not homotopy nilpotent provided the homology $\tilde {H}_{\ast }(X, \mathbb {F})$ has at least two primitive generators, where $\mathbb {F}$ is a field. Then, we state the main result

Theorem 3.8 If $M(A,n)$ is a Moore space with $n\ge 2$ then

$$ \begin{align*} \mathrm{nil}\,\Omega(M(A, n)) < \infty \end{align*} $$

if and only if A is a torsion-free group with rank $r(A) = 1$ or equivalently, A is a subgroup of $\mathbb {Q}$ .

Unfortunately, Moore spaces of type $(A,1)$ are not determined uniquely (up to homotopy) by an Abelian group A. At the end, we present constructions and analyse the homotopy nilpotency of some loop spaces $\Omega (M(A,1))$ .

2 Prerequisites

All spaces and maps in this note are assumed to be connected and based with the homotopy type of $CW$ -complexes unless we assume otherwise. We also do not distinguish notationally between a continuous map and its homotopy class. We write $\Omega (X)$ (resp. $\Sigma (X)$ ) for the loop (resp. suspension) space on a space X and $[X,Y]$ for the set of homotopy classes of maps $X\to Y$ .

Given a space X, we use the customary notations $X\vee X$ and $X\wedge X$ for the wedge and the smash square of X, respectively.

Recall that an H-space is a pair $(X,\mu )$ , where X is a space and $\mu : X \times X \to X$ is a map such that the diagram

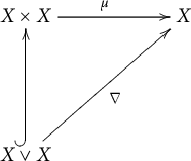
commutes up to homotopy, where $\nabla : X\vee X\to X$ is the folding map. We call $\mu $ a multiplication or an H-structure for X. Two examples of H-spaces come in mind: topological groups and the loop spaces $\Omega (X)$ . In the sequel, we identify an H-space $(X,\mu )$ with the space X.

An H-space X is called a group-like space if X satisfies all the axioms of groups up to homotopy. Recall that a homotopy associative H– $CW$ -complex always has a homotopy inverse. More precisely, according to [24, 1.3.2. Corollary] (see also [1, Proposition 8.4.4]), we have

Proposition 2.1 If X is a homotopy associative H– $CW$ -complex then X is a group-like space.

From now on, we assume that any H-space X is group-like.

Given spaces $X_1,\ldots ,X_n$ , we use the customary notations $X_1\times \cdots \times X_n$ for their Cartesian and $T_m(X_1,\ldots ,X_n)$ for the subspace of $X_1\times \cdots \times X_n$ consisting of those points with at least m coordinates at base points with $m=0,1,\ldots ,n$ . Then, $T_0(X_1,\ldots ,X_n)= X_1\times \cdots \times X_n$ , $T_1(X_1,\ldots ,X_n)$ is the so called the fat wedge of spaces $X_1,\ldots ,X_n$ and $T_{n-1}(X_1,\ldots ,X_n)=X_1\vee \cdots \vee X_n$ , the wedge products of spaces $X_1,\ldots ,X_n$ . We write $j_m(X_1,\ldots ,X_n) : T_m(X_1,\ldots ,X_n)\to X_1\times \cdots \times X_n$ for the inclusion map with $m=0,1,\ldots ,n$ and $X_1\wedge \cdots \wedge X_n= X_1\times \cdots \times X_n/T_1(X_1,\ldots ,X_n)$ for the smash product of spaces $X_1,\ldots ,X_n$ .

Let $f_m: (X_m,\star _m)\to (Y_m,\ast _m)$ be continuous maps of pointed topological spaces for $m=1,\ldots ,n$ . The map $f_1\times \cdots \times f_n : (X_1\times \cdots \times X_n, (\star _1,\ldots ,\star _n))\to (Y_1\times \cdots \times Y_n,(\ast _1,\ldots ,\ast _n))$ sends the point $(x_1,\ldots ,x_n)$ into $(f_1(x_1),\ldots , f_n(x_n))$ for $(x_1,\ldots ,x_n)\in X_1\times \cdots \times X_n$ and restricts to maps $T_m(f_1,\ldots ,f_n) : T_m(X_1,\ldots , X_n)\to T_m(Y_1,\ldots ,Y_n)$ with $m=0,1,\ldots ,n$ . If $X_m=X$ and $f_m=f$ for $m=1,\ldots ,n$ then we write $X^n=X_1\times \cdots \times X_n$ , $X^{\wedge n}=X_1\wedge \cdots \wedge X_n$ , $f^n=f_1\times \cdots \times f_n$ and $f^{\wedge n}=f_1\wedge \cdots \wedge f_n$ . The identity map of a space X involved is consistently denoted by $\iota _X$ .

Given an H-group X, the functor $[ -, X]$ takes its values in the category of groups. One may then ask when those functors take their values in various subcategories of groups. For example, X is homotopy commutative if and only if $[Y, X]$ is Abelian for all Y.

Given an H-space X, we write $\varphi _{X,1}=\iota _X$ , $\varphi _{X,2}: X^2\to X$ for the basic commutator map and $\varphi _{X,n+1}=\varphi _{X,2}\circ (\varphi _{X,n}\times \iota _X)$ for $n\ge 2$ .

2.1 The nilpotency class

The nilpotency class $\mathrm{nil}\, (X,\mu )$ of an H-space $(X,\mu )$ is the least integer $n\ge 0$ for which the map $\varphi _{X,n+1}\simeq \ast $ is nullhomotopic and we call the homotopy associative H-space X homotopy nilpotent. If no such integer exists, we put $\mathrm{nil}\,(X,\mu )=\infty $ . In the sequel, we simply write $\mathrm{nil}\, X$ for the nilpotency class of an H-space X. Thus, $\mathrm{nil}\, X=0$ if and only if X is contractible and, as is easily seen, $\mathrm{nil}\, X\le 1$ if and only if X is homotopy commutative.

The set $\pi _0(X)$ of all path-components of an H-space X is known to be a group. The following result is easy to prove:

Lemma 2.2 If X is an H-space and the path component of the base-point $\star \in X$ is contractible then $\mathrm{nil} \pi _0(X) = \mathrm{nil}\,X.$

The definition of the nilpotency classes may be extended to maps. The nilpotency class $\mathrm{nil}\, f$ of an H-map $f: X_1\to X_2$ is the least integer $n\ge 0$ for which the map $f\circ \varphi _{X,n+1} : X_1^{n+1}\to X_2$ is nullhomotopic; if no such integer exists, we put $\mathrm{nil}\, f=\infty $ .

In the sequel, we need

Lemma 2.3 If X is an H-space then the composite map

$$ \begin{align*} T_1(X,\ldots,X)\stackrel{j_1(X,\ldots,X)}{\longrightarrow}X^n\stackrel{\varphi_{X,n}}{\longrightarrow}X \end{align*} $$

is nullhomotopic.

Since the space $X^{\wedge n}$ , the nth smash power of X is the homotopy cofiber of the map $j_1(X,\ldots ,X) : T_1(X,\ldots ,X)\to X^n$ , the result above implies an existence of a map $\bar {\varphi }_{X,n} : X^{\wedge n}\to X$ for $n\ge 1$ with $\bar {\varphi }_{X,1}=\varphi _{X,1}$ .

It is well known that the quotient map $X^n \to X^{\wedge n}$ has a right homotopy inverse after suspending for $n\ge 1$ , and the fact that X is an H-space means that the suspension map $[Y,X] \to [\Sigma Y,\Sigma X]$ is a monomorphism for any space Y. Thus, we may state

Proposition 2.4 Let X be an H-space. Then $\varphi _{X,n}\simeq \ast $ if and only if $\bar {\varphi }_{X,n}\simeq \ast $ for $n\ge 1$ .

Then, [3, 2.7. Theorem] and Proposition 2.4 lead to

Theorem 2.5 If X is an H-space then

$$ \begin{align*} \mathrm{nil}\,X=\sup_m\mathrm{nil}[X^m,X]= \sup_m\mathrm{nil}[X^{\wedge m},X]=\sup_Y\mathrm{nil}[Y,X], \end{align*} $$

where m ranges over all integers and Y over all topological spaces.

Furthermore, in view of [24, Lemma 2.6.1], we may state

Corollary 2.6 A connected H-space X is homotopy nilpotent if and only if the functor $[- , X]$ on the category of all spaces is nilpotent group valued.

Proof Certainly, the homotopy nilpotency of a connected associative H-space X implies that the functor $[- , X]$ on the category of all pointed spaces is nilpotent group valued.

Now, suppose that the functor $[-, X]$ is nilpotent groups valued and $\mathrm{nil}\,[\prod _1^{\infty } X, X] < n$ . Then, for the projection map $\prod _1^{\infty } X\to X^n$ on the first n factors, the composite map

$$ \begin{align*} \prod_1^{\infty} X \to X^n\stackrel{\varphi_{X,n}}{\longrightarrow} X \end{align*} $$

is null-homotopic. Since, the projection $\prod _1^{\infty } X\to X^n$ has a retraction, we deduce that the map $\varphi _{X,n} : X^n\to X$ is also null-homotopic and the proof is complete.▪

Next, notice that for a map $f : X\to Y$ of H-spaces, we have the commutative (up to homotopy) diagram

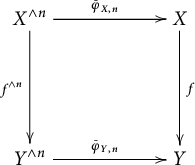
with $n\ge 1$ .

This yields

Remark 2.7 If $X'$ is an H-subspace of an H-space X and $r: X\to X'$ its a homotopy H-retract then one can easily derive from the above that

$$ \begin{align*} \mathrm{nil}\,X'\le\mathrm{nil}\,X. \end{align*} $$

2.2 Homotopy nilpotency of spaces

With any based space X, we associate the integer $\mathrm{nil}\,\Omega (X)$ called the nilpotency class of X. Evidently, $\mathrm{nil}\,\pi _1(X)\le \mathrm{nil}\, \Omega (X)$ . We give an extension of this result involving Whitehead products, generally denoted by $[\alpha _1,\alpha _2]\in \pi _{m_1+m_2-1}(X)$ if $\alpha _i\in \pi _{m_i}(X)$ for $m_i\ge 1$ with $i=1,2$ .

We define $(n+1)$ -fold Whitehead products $[\alpha _1,\ldots ,\alpha _{n+1}]$ as $[[\alpha _1,\ldots ,\alpha _n],\alpha _{n+1}]$ if $\alpha _i\in \pi _{m_i}(X)$ for $m_i\ge 1$ with $i=1,\ldots ,n+1$ agreeing that, for $n=0$ , $[\alpha ]=\alpha $ .

Recall that $\text {W-length}\,X$ , the Whitehead length of a space X is the least integer $n\ge 0$ such that $[\alpha _1,\ldots ,\alpha _{n+1}]=0$ for all $\alpha _i\in \pi _{m_i}(X)$ , $m_i\ge 1$ ; if no such integer exists, we put $\text {W-length}\,X=\infty $ .

Then, according to [3, 4.6. Theorem], we by have:

Theorem 2.8 $\text {W-length}\,X\le \mathrm{nil}\,\Omega (X).$

Example 2.9 (1) It is well-known that

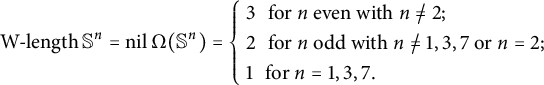$$ \begin{align*} \text{W-length}\,\mathbb{S}^n=\mathrm{nil}\,\Omega(\mathbb{S}^n)=\begin{cases} ~3\, ~\text{ for } n \text{ even with } n\not=2;\\ ~2\, ~\text{ for } n \text{ odd with } n\not=1,3,7 \text{ or } n=2;\\ ~1\, ~\text{ for } n=1,3,7. \end{cases} \end{align*} $$

(2) For the wedge $\mathbb {S}^m\vee \mathbb {S}^n$ of two spheres with $m,n\ge 2$ , there is an iterated nontrivial Whitehead product of any length. Therefore, by Theorem 2.8, we conclude that

(2.1) $$ \begin{align} \mathrm{nil}\,\Omega(\mathbb{S}^m\vee \mathbb{S}^n)=\infty. \end{align} $$

The concept of a nilpotent space is due to Dror [6]. Recall that a pointed path-connected space X is said to be nilpotent if its fundamental group $\pi _1(X)$ acts nilpotently on the higher homotopy groups $\pi _n(X)$ for $n\ge 1$ . Since, the action of $\pi _1(X)$ on $\pi _n(X)$ for $n\ge 1$ may be written in terms of Whitehead products, the nilpotency of a space X is a lower bound of its homotopy nilpotency $\mathrm{nil}\,\Omega (X)$ . Therefore, by Theorem 2.8, the space X is nilpotent if $\Omega (X)$ is homotopy nilpotent. But, by Example 2.9(2), not every space $\Omega (X)$ is homotopy nilpotent if X is nilpotent or even simply connected.

Dror [6] has also published a far-reaching generalization of a classical theorem of J.H.C. Whitehead useful in the next section.

Theorem 2.11 If $f: X\to Y$ is a map of connected, pointed, $CW$ -complexes which induces an isomorphism on integral homology, and if X and Y are nilpotent spaces, then f is a homotopy equivalence.

3 Moore spaces

We take up the systematic study of the homotopy nilpotency of Moore spaces $M(A,n)$ for $n\ge 1$ . In Eckmann–Hilton duality, Moore spaces play the role of dual objects of Eilenberg–MacLane $CW$ -complexes.

Let A be an Abelian group and n any integer $\ge 1$ . A $CW$ -complex X (if one such exists) satisfying $\pi _j(X) = 0$ for $j < n$ , $\pi _n(X)\approx A$ and $H_i(X)=0$ for $i> n$ is known as a Moore space of type $(A, n)$ , or simply an $M(A, n)$ space. By [15], it is known that a Moore space $M(A,n)$ with $n\ge 2$ exists and, in view of [11, Example 4.34], the homotopy type of a Moore space $M(A,n)$ is uniquely determined by A and $n\ge 2$ . This implies that every Moore space $M(A,n)$ with $n\ge 3$ , is the suspension $\Sigma M(A,n-1)$ . Furthermore, in [2, Section 2], it was shown that also $M(A,2)$ is a suspension $\Sigma L(A)$ for some $CW$ -complex $L(A)$ .

By means of [20, Proposition 1.1], there exists an $M(A,1)$ space if and only if the homology $H_2(A,\mathbb {Z})=0$ for the ring $\mathbb {Z}$ of integers. Recall also that, by [14, Theorem 3], $H_2(A,\mathbb {Z})=0$ if and only if $A\otimes A$ coincides with its subgroup generated by the diagonal, $\{a\otimes a;\,a\in A \}$ .

Remark 3.1 If $X_1,X_2$ are Moore spaces of type $(A,1)$ then, like for Moore spaces of type $(A,n)$ with $n \ge 2$ , there is an integral homotopy isomorphism $f : X_1\to X_2$ . If the spaces $X_1,X_2$ are nilpotent then Theorem 2.11 implies that $f : X_1 \to X_2$ is a homotopy equivalence.

However, the homotopy type of a Moore space $M(A,1)$ is not uniquely determined by A. Hatcher [11, Example 4.35] constructed the space $X=(\mathbb {S}^1\vee \mathbb {S}^n)\cup e^{n+1}$ such that the inclusion $\mathbb {S}^1 \hookrightarrow X$ induces an isomorphism on all homology groups and on $\pi _k$ for $k < n$ , but not on $\pi _n$ . More precisely, from [11, Example 4.27] we have $\pi _n(\mathbb {S}^1\vee \mathbb {S}^n)\approx \mathbb {Z}[t,t^{-1}]/(2t-1)$ . Then, X is obtained from $\mathbb {S}^1\vee \mathbb {S}^n$ by attaching a cell $e^{n+1}$ via a map $\mathbb {S}^n\to \mathbb {S}^1\vee \mathbb {S}^n$ corresponding to $2t-1\in \mathbb {Z}[t,t^{-1}]$ .

3.1 Moore spaces of type $\mathbf {(A,n)}$ with $\mathbf {n}\ge \mathbf {2}$

To examine the homotopy nilpotency of $M(A,n)$ with $\ge 2$ , we need to fix some notations and recall a definition. Given a pointed space X, write $i_1,i_2 : X\to X\times X$ for the canonical embedding maps and $\Delta : X\to X\times X$ for the diagonal map. If $H_m(X,A)$ is the mth homology group of X with coefficient in an Abelian group A then an element $\alpha \in H_m(X,A)$ is said to be primitive if $\Delta _{\ast }(\alpha )=i_{1\ast }(\alpha )+ i_{2\ast }(\alpha )$ for the induced homomorphisms $i_{1 \ast },i_{2 \ast }, \Delta _{\ast } : H_m(X,A)\to H_m(X\times X,A)$ .

We show that the space $\Omega \Sigma (X)$ is not homotopy nilpotent provided the homology $H_{\ast }(X, \mathbb {F})$ has at least two primitive generators, where $\mathbb {F}$ is a field.

Proposition 3.2 If $\tilde {H}_{\ast }(X, \mathbb {F})$ has at least two primitive generators, where $\mathbb {F}$ is a field then $\Omega \Sigma (X)$ is not homotopy nilpotent.

Proof To see this, take homology $H_{\ast }(X,\mathbb {F})$ with $\mathbb {F}$ -coefficients and recall that the Bott–Samelson Theorem [4] states that $H_{\ast }(\Omega \Sigma X,\mathbb {F})\approx T(\tilde {H}_{\ast }(X,\mathbb {F}))$ , the tensor algebra on $\tilde {H}_{\ast }(X,\mathbb {F})$ . This may be rewritten as $UL\big <\tilde {H}_{\ast }(X,\mathbb {F})\big>$ , where $L\big <\tilde {H}_{\ast }(X,\mathbb {F})\big>$ is the free Lie algebra generated by $\tilde {H}_{\ast }(X,\mathbb {F})$ and $UL\big <\tilde {H}_{\ast }(X,\mathbb {F})\big>$ is its universal enveloping algebra. Further, the suspension $E : X \to \Omega \Sigma X$ induces the inclusion of the generating set in homology. Now, consider the iterated Samelson product

$$ \begin{align*} s_k : X^{\wedge k}\longrightarrow \Omega\Sigma (X), \end{align*} $$

where $s_1=E$ , $s_{k+1}=\big < E,s_k\big>$ , the Samelson product of $s_k$ and E for $n\ge 2$ . For any $x\in \tilde {H}_{\ast }(X)$ we have $E_{\ast }(x) = x$ .

The effect of the Samelson product map on homology classes of loop spaces is presented e.g., in [22, Chapter X, Section 6] (see also [11, Chapter 3]). If $x_1,\ldots ,x_k\in \tilde {H}_{\ast }(X,\mathbb {F})$ are primitive then the class $x_1\otimes \cdots \otimes x_k\in \tilde {H}_{\ast } (X,\mathbb {F})^{\otimes k}\approx \tilde {H}_{\ast }(X^{\wedge k},\mathbb {F})$ is sent by $(s_k)_{\ast }$ to the iterated bracket $[x_1, [x_2,\ldots [x_{k-1},x_k]]\ldots ]$ . In particular, if $x,y\in \tilde {H}_{\ast }(X,\mathbb {F})$ are distinct primitive generators then the class

$$ \begin{align*} [x, [x,\ldots[x, y]]\ldots]\in UL\tilde{H}_{\ast}(X,\mathbb{F})\approx \tilde{H}_{\ast}(\Omega\Sigma (X),\mathbb{F}) \end{align*} $$

is in the image of $(s_k)_{\ast }$ . Hence, $s_k$ cannot be null homotopic.

Notice that for the map $s_k : X^{\wedge k}\longrightarrow \Omega \Sigma (X)$ defined above, there is by the factorization

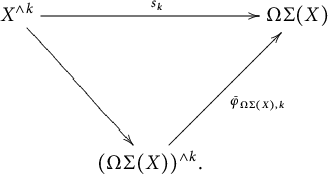
Consequently, the map $\bar {\varphi }_{\Omega \Sigma X,k}$ is not null homotopic provided the map $s_k$ is so and the proof is complete.▪

To apply Proposition 3.2 for computations of $\mathrm{nil}\,\Omega M(A,n)$ , we need

Lemma 3.3 If $n\ge 2$ then

$$ \begin{align*} \mathrm{nil}\,\Omega(M(\mathbb{Z}_{p^{m}},n))=\infty \end{align*} $$

for $m=1,2,\ldots \infty $ and $n\ge 2$ .

Proof Given an Abelian group A, write $X_n(A)=M(A,n)$ with $n\ge 2$ or $X_1(A)=L(A)$ . Then, by the Universal Coefficient Theorem, we have

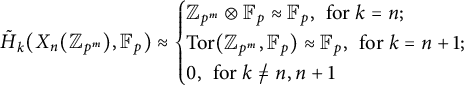$$ \begin{align*} \tilde{H}_k(X_n(\mathbb{Z}_{p^m}),\mathbb{F}_p)\approx \begin{cases}\mathbb{Z}_{p^m}\otimes \mathbb{F}_p\approx\mathbb{F}_p ,\,\text{ for } k = n;\\ \text{Tor}(\mathbb{Z}_{p^m}, \mathbb{F}_p)\approx\mathbb{F}_p,\,\text{ for } k = n +1;\\ 0,\,\text{ for } k\not=n,n+1\end{cases} \end{align*} $$

for $m,n\ge 1$ .

Since the Moore space $M(\mathbb {Z}_{p^m},n)\simeq \Sigma X_{n-1}(\mathbb {Z}_{p^m})$ for $n\ge 2$ , we can consider the iterated Samelson product

$$ \begin{align*} s_n : (X_{n-1}(\mathbb{Z}_{p^m}))^{\wedge k}\longrightarrow \Omega M(\mathbb{Z}_{p^m},n)\simeq \Omega\Sigma (X_{n-1}(\mathbb{Z}_{p^m})). \end{align*} $$

Thus, Proposition 3.2 implies that

(3.1) $$ \begin{align} \mathrm{nil}\,\Omega(M(\mathbb{Z}_{p^m},n))=\infty \end{align} $$

for $m\ge 1$ and $n\ge 2$ .

Because $\mathbb {Z}_{p^{\infty }}\otimes \mathbb {F}_p=0$ , we have

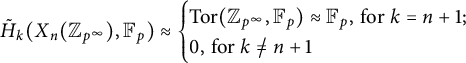$$ \begin{align*} \tilde{H}_k(X_n(\mathbb{Z}_{p^{\infty}}),\mathbb{F}_p)\approx \begin{cases} \text{Tor}(\mathbb{Z}_{p^{\infty}}, \mathbb{F}_p)\approx\mathbb{F}_p,\,\text{for } k=n+1;\\ 0,\,\text{for } k\not=n+1 \end{cases} \end{align*} $$

for $n\ge 1$ . Thus, the argument above collapses.

Therefore, we process as follows. Given $n\ge 2$ and a prime p, consider the mapping telescope T determined by the sequence of maps

$$ \begin{align*} \mathbb{S}^n\stackrel{p}\to\mathbb{S}^n\stackrel{p}\to\mathbb{S}^n\stackrel{p}\to\cdots. \end{align*} $$

Recall that T is the union of the mapping cylinders $M_k$ with the copies of $\mathbb {S}^n$ in $M_k$ and $M_{k-1}$ identified for all k. Thus, T is the quotient space of the disjoint union $\bigsqcup _{k=1}^{\infty } \mathbb {S}^n\times [k,k+1]$ in which each point $(x_k,k+1)\in \mathbb {S}^n\times [k,k+1]$ is identified with $(p(x_k),k+1)\in \mathbb{S}^n\times [k+1,k+2]$ . In the mapping telescope T, let $T_m$ be the union of the first m mapping cylinders. This deformation retracts onto $\mathbb {S}^n$ by deformation retracting each mapping cylinder onto its right end in turn. Since the maps $p: \mathbb {S}^n\to \mathbb {S}^n$ are cellular, each mapping cylinder is a $CW$ -complex and the telescope T is the increasing union of the subcomplexes $T_m\simeq \mathbb {S}^n$ .

If we attach a cell $e^{n+1}$ to the first $\mathbb {S}^n$ in T via the identity map of $\mathbb {S}^n$ , we obtain a space X which is the increasing union of its subspaces $X_m=T_m\cup e^{n+1}$ being $M(\mathbb {Z}_{p^m},n)$ ’s. Since, $H_n(X,\mathbb {Z})\approx \text {colim}_mH_n(X_m,\mathbb {Z})=\text {colim}_m\mathbb {Z}_{p^m}=\mathbb {Z}_{p^{\infty }}$ and $H_k(X,\mathbb {Z})=0$ for $k\not =n$ , we derive that X is the Moore space of type $(\mathbb {Z}_{p^{\infty }},n)$ .

Furthermore, $\Omega (X)=\text {colim}_m\Omega (X_m)$ implies a homotopy equivalence

$$ \begin{align*} \Omega(M(\mathbb{Z}_{p^{\infty}},n))\simeq\text{colim}_m\Omega(M(\mathbb{Z}_{p^m},n)). \end{align*} $$

Thus, the nontrivial maps

$$ \begin{align*} \bar{\varphi}_{\Omega(M(\mathbb{Z}_{p^m},n)),k} : \Omega(M(\mathbb{Z}_{p^m},n))^{\wedge k}\longrightarrow \Omega(M(\mathbb{Z}_{p^m},n)) \end{align*} $$

for $k,m\ge 1$ determined by (3.1) yield the nontrivial the maps

$$ \begin{align*} \bar{\varphi}_{\Omega(M(\mathbb{Z}_{p^{\infty}},n)),k} : \Omega(M(\mathbb{Z}_{p^{\infty}},n))^{\wedge k}\longrightarrow \Omega(M(\mathbb{Z}_{p^{\infty}},n)) \end{align*} $$

for $k\ge 1$ and $n\ge 2$ . Consequently,

(3.2) $$ \begin{align} \mathrm{nil}\,\Omega(M(\mathbb{Z}_{p^{\infty}},n))=\infty \end{align} $$

for $n\ge 2$ and this concludes the proof.▪

Now, recall that by [7, Corollary 27.4], an Abelian group A with elements of finite order contains a direct summand $\mathbb {Z}_{p^m}$ for some prime p and $m=1,2,\ldots $ or $\infty $ . Then, such an Abelian group $A\approx \mathbb {Z}_{p^m}\oplus B$ for some Abelian group B and $m=1,\ldots ,\infty $ .

Hence, $M(A,n)=M(\mathbb {Z}_{p^m},n)\vee M(B,n)$ and so Remark 2.7, and Lemma 3.3 imply that

$$ \begin{align*} \mathrm{nil}\,\Omega(M(A,n))=\infty \end{align*} $$

for $n\ge 2$ .

Given an Abelian group A, we have $A\otimes \mathbb {Q}=\bigoplus _1^{{\tiny r}(A)}\mathbb {Q}$ for the rank $r(A)$ of A and the additive group $\mathbb {Q}$ of the field $\mathbb {Q}$ of rationals. Therefore,

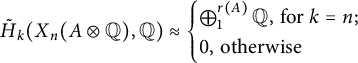$$ \begin{align*} \tilde{H}_k(X_n(A\otimes\mathbb{Q}),\mathbb{Q})\approx \begin{cases}\bigoplus_1^{r(A)}\mathbb{Q},\,\text{for } k = n;\\ 0,\,\text{otherwise} \end{cases}\end{align*} $$

for $n\ge 1$ .

Next, by for a nilpotent space X and a set of primes I, write $X_{(I)}$ for the I-localization of X. Then, by [13, Proposition 3.5], we have

Proposition 3.6 Let X be a nilpotent space. If $\mathrm{nil}\, \Omega (X) < n$ then $\mathrm{nil}\,\Omega (X_{(I)}) < n$ for every set of primes I.

Since the Moore space $M(A,n)\simeq \Sigma X_{n-1}(A)$ for $n\ge 2$ , we can consider the iterated Samelson product

$$ \begin{align*} s_n : (X_{n-1}(A))^{\wedge k}\longrightarrow \Omega M(A,n)\simeq \Omega\Sigma (X_{n-1}(A)). \end{align*} $$

Furthermore, if $r(A)\ge 2$ then Proposition 3.2 yields $\mathrm{nil}\,\Omega (M(A\otimes \mathbb {Q},n))=\infty $ . Since $M(A\otimes \mathbb {Q},n)=M(A,n)_{(0)}$ , the rationalization of $M(A,n)$ , Proposition 3.6 leads to $\mathrm{nil}\,\Omega (M(A,n))=\infty $ . Thus, in view of Proposition 3.2 and Lemma 3.3, we may state

Corollary 3.7 If A is an Abelian group with elements of finite order or $r(A)\ge 2$ then

$$ \begin{align*} \mathrm{nil}\, \Omega(M(A,n)) = \infty \end{align*} $$

for $n\ge 2$ .

If $r(A)=1$ and A is a torsion-free Abelian group then by [7, Chapter IV, Section 24], we know that A is a subgroup of $\mathbb {Q}$ . Notice that $M(\mathbb {Q},n)=\mathbb {S}^n_{(0)}=K(\mathbb {Q},n)$ , the Eilenberg–MacLane of type $(\mathbb {Q},n)$ provided n is odd. Therefore, $M(\mathbb {Q},n)$ is a homotopy commutative and associative H-space and $\mathrm{nil}\,M(\mathbb {Q},n)=\mathrm{nil}\,\Omega (M(\mathbb {Q},n))=~1$ .

Given any subgroup $A< \mathbb {Q}$ , we have a sequence $\mathbb {Z}\stackrel {n_0}\to \mathbb {Z}\stackrel {n_1}\to \mathbb {Z}\stackrel {n_2}\to \cdots $ and $A=\text {colim}_{n_k}\mathbb {Z}$ . Next, for $n\ge 2$ , the mapping telescope T of the associated sequence of maps

$$ \begin{align*} \mathbb{S}^n\stackrel{n_0}\to\mathbb{S}^n\stackrel{n_1}\to\mathbb{S}^n\stackrel{n_2}\to\cdots \end{align*} $$

is the union of the mapping cylinders $M_{n_k}$ with the copies of $\mathbb {S}^n$ in $M_{n_k}$ and $M_{n_{k-1}}$ identified for all k. In the mapping telescope T, let $T_m$ be the union of the first m mapping cylinders. This deformation retracts onto $\mathbb {S}^n$ by deformation retracting each mapping cylinder onto its right end in turn. Since the maps $n_k: \mathbb {S}^n\to \mathbb {S}^n$ are cellular, each mapping cylinder is a $CW$ -complex and the telescope T is the increasing union of the subcomplexes $T_m\simeq \mathbb {S}^n=M(\mathbb {Z},n)$ . Next, by $\tilde {H}_n(T,\mathbb {Z})\approx A=\text {colim}_m\mathbb {Z}$ and $\tilde {H}_k(T,\mathbb {Z})=0$ for $k\not =n$ , we derive that $T=M(A,n)=\text {colim}_mT_m$ . Then, $\Omega (M(A,n))= \text {colim}_m\Omega (T_m)$ and $\mathrm{nil}\,\Omega (T_m))=\mathrm{nil}\,\Omega (\mathbb {S}^n)\le 3$ imply that $\mathrm{nil}\,\Omega (M(A,n))\le 3$ provided $n\ge 2$ and we get the main result

Theorem 3.8 If $M(A,n)$ is a Moore space with $n\ge 2$ then

$$ \begin{align*} \mathrm{nil}\,\Omega(M(A, n)) < \infty \end{align*} $$

if and only if A is a torsion-free group with rank $r(A) = 1$ or equivalently, A is a subgroup of $\mathbb {Q}$ .

3.2 Moore spaces of type $\mathbf {(A,1)}$

We present constructions and analyse homotopy nilpotency of some Moore spaces of type $(A,1)$ .

(1) The space $X=(\mathbb {S}^1\vee \mathbb {S}^n)\cup e^{n+1}$ constructed by Hatcher [11, Example 4.35] is a Moore space of type $(\mathbb {Z},1)$ for the infinite cyclic group $\mathbb {Z}\big < t\big>$ . Since $\pi _1(X)\approx \mathbb {Z}\big < t\big>$ and $\pi _n(\mathbb {S}^1\vee \mathbb {S}^n)\approx \mathbb {Z}[t,t^{-1}]/(2t-1)$ , we get that $(2t-1)\alpha =0$ implies $2t\alpha =\alpha $ for $\alpha \in \mathbb {Z}[t,t^{-1}]/(2t-1)$ . Hence, the action of $\pi _1(X)$ on $\pi _n(X)$ is nontrivial.

Note that the map $\mathbb {Z}[t,t^{-1}]\to \mathbb {Q}$ given by $t\mapsto 1/2$ yields a ring isomorphism

$$ \begin{align*} \mathbb{Z}[t,t^{-1}]/(2t-1)\stackrel{\approx}{\longrightarrow}\mathbb{Z}[1/2] \end{align*} $$

for the subring $\mathbb {Z}[1/2]\subseteq \mathbb {Q}$ consisting of rationals with denominator a power of $2$ . Then, $\pi _1(X)\approx \mathbb {Z}\big < t\big > $ acts on $\pi _n(X)\approx \mathbb {Z}[1/2]$ by $t\alpha =(1/2)\alpha $ for $\alpha \in \mathbb {Z}[1/2]$ . Consequently, the Whitehead product $[t,\alpha ]=(-1/2)\alpha $ and the $(n+1)$ -fold Whitehead product $[\alpha ,t,t\ldots ,t]=(-1/2)^n\alpha $ is non-trivial for $n\ge 1$ provided $\alpha \not =0$ . Consequently, in view of Theorem 2.8,

$$ \begin{align*} \mathrm{nil}\,\Omega(X)= \infty. \end{align*} $$

(2) The space $\mathbb {R}P^2_m=\mathbb {S}^1\cup _me^2$ is a Moore space of type $(\mathbb {Z}_m,1)$ for the cyclic group $\mathbb {Z}_m$ of order m. Then, $\pi _1(\mathbb {R}P^2_m)=\mathbb {Z}_m\big < t\big > $ , where t is represented by the canonical map $i : \mathbb {S}^1\to \mathbb {R}P^2_m$ . Next, by [18], the group $\pi _2(\mathbb {R}P^2_m)$ can be identified with the ideal of the group ring $\mathbb {Z}[\mathbb {Z}_m]$ generated by $\alpha =1-t$ , so that as an Abelian group $\pi _2(\mathbb {R}P^2_m)$ is free of rank $m-1$ and, as $\pi _1$ -module, $\pi _2(\mathbb {R}P^2_m)$ has a single generator $\alpha $ , subject solely to the relation $(1+t+\cdots +t^{m-1})\alpha =0$ . Then, the Whitehead product $[\alpha ,t]=t\alpha -\alpha =2\alpha -t^2\alpha -\cdots -t^{m-1}\alpha $ . Thus, we derive that the $(n+1)$ -fold Whitehead product $[\alpha ,t,t\ldots ,t]$ is non-trivial for $n\ge 1$ . Consequently, in view of Theorem 2.8, we derive that

$$ \begin{align*} \mathrm{nil}\,\Omega(\mathbb{R}P^2_m)=\infty \end{align*} $$

for $m\ge 2$ .

(3) If $r(A)=1$ and A is a torsion-free Abelian group then $A< \mathbb {Q}$ , we have a sequence of maps $\mathbb {Z}\stackrel {n_0}\to \mathbb {Z}^n\stackrel {n_1}\to \mathbb {Z}\stackrel {n_2}\to \cdots $ and $A=\text {colim}_{k_i}\mathbb {Z}$ . Next, the mapping telescope T of the associated sequence of maps

$$ \begin{align*} \mathbb{S}^1\stackrel{n_0}\to\mathbb{S}^1\stackrel{n_1}\to\mathbb{S}^1\stackrel{n_2}\to\cdots \end{align*} $$

is the union of the mapping cylinders $M_{n_k}$ with the copies of $\mathbb {S}^1$ in $M_{n_k}$ and $M_{n_{k-1}}$ identified for all k. In the mapping telescope T, let $T_m$ be the union of the first m mapping cylinders. This deformation retracts onto $\mathbb {S}^1$ by deformation retracting each mapping cylinder onto its right end in turn. Since the maps $n_k: \mathbb {S}^1\to \mathbb {S}^n$ are cellular, each mapping cylinder is a $CW$ -complex and the telescope T is the increasing union of the subcomplexes $T_m\simeq \mathbb {S}^1$ .

Next, by $\tilde {H}_1(T,\mathbb {Z})\approx \text {colim}_mH_1(T_m)=\text {colim}_{k_i}\mathbb {Z}=A$ and $\tilde {H}_k(T,\mathbb {Z})=0$ for $k\not =1$ , we derive that $T=\text {colim}_mT_m$ is a Moore space of type $(A,1)$ . Furthermore, $\pi _k(T)=\text {colim}_m\pi _k(T_m)$ implies that $\pi _1(T)=\text {colim}_m\mathbb {Z}=A$ and $\pi _k(T)=0$ for $k\not = 1$ . Consequently, $T=\text {colim}_mT_m$ , as the Eilenberg-MacLane space $K(A,1)$ , is a homotopy commutative and an associative H-space. Finally, we get that

$$ \begin{align*} \mathrm{nil}\,T= 1. \end{align*} $$

(4) At the end, given a prime p, consider the telescope T determined by the sequence of maps

$$ \begin{align*} \mathbb{S}^1\stackrel{p}\to\mathbb{S}^1\stackrel{p}\to\mathbb{S}^1\stackrel{p}\to\cdots. \end{align*} $$

If we attach a cell $e^2$ to the first $\mathbb {S}^1$ in T via the identity map of $\mathbb {S}^1$ , we obtain a space X which is a Moore space of type $(\mathbb {Z}_{p^{\infty }},1)$ since X is the increasing union of its subspaces $X_m=T_m\cup e^2$ , which are $\mathbb {R}P^2_{p^m}$ ’s. Then, (2) leads to

$$ \begin{align*} \mathrm{nil}\,\Omega(X)= \infty. \end{align*} $$