Article contents
XIV.—The Minimum System of Two Quadratic Forms in n Variables
Published online by Cambridge University Press: 15 September 2014
Extract
The following pages deal with the simultaneous system of two general quadratic forms in n homogeneous variables. It is a special case of Gordan's Theorem which proves such systems to be finite, for the general projective group of linear transformations. While several works have dealt with the cases when n = 2, 3, or 4, nothing seems to have been written on the general case except a memoir in the year 1908. We continue, and simplify, the results there obtained, and now establish that
(1) All rational integral concomitants of two quadratic forms in n variables and any number of sets of linear variables may be expressed as rational integral functions of (3n + 1) concomitants, and forms derivable by polarisation.
(2) These (3n + 1) forms, called the H system, constitute a strictly irreducible system.
This system is exhibited in §7 as
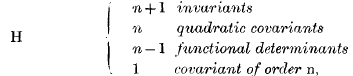
together with polars.
The work is divided into three chapters: I §§ 1–4 is introductory notation, II §§ 5–17 provides a proof of these theorems, while III §§18–21 gives the non-symbolic and canonical forms of the results.
- Type
- Proceedings
- Information
- Copyright
- Copyright © Royal Society of Edinburgh 1926
References
page 149 note * Hilbert, , Math. Ann., xxxvi (1890), p. 473 CrossRefGoogle Scholar; Grace and Young, Algebra of Invariants.
page 149 note † Hilbert, , Math. Ann., xxxvi (1890), p. 161 Google Scholar, also pp. 274–286.
page 149 note ‡ Turnbull, , Proc. London Math. Sec. (2), xviii (1919), pp. 69–91 Google Scholar.
page 149 note § Turnbull, , Trans. Camb. Phil. Soc., xxi (1909), pp. 197–240 Google Scholar.
page 150 note * Clebsch, , Göttinger Nachrichten, xvii (1872); Weitzenböck, Invariantentheorie, pp. 148–159, Groningen (1923)Google Scholar.
page 151 note * Clebsch, , Crelle, lix (1861)Google Scholar; Weitzenböck, Wiener Berichte, cxxii (1913); Grace and Young, Algebra of Invariants.
page 157 note * This is permissible as the suffix is simply a distinguishing mark and does not denote currency.
page 159 note * This method is only safe after proving that a canonical form exists which involves the same number of constants. Otherwise it may lead to a downright error. We here assume the well-known result that the two general quadratic forms can be expressed simultaneously each as the sum of n squares.
page 159 note † Gilham, , Proc. London Math. Soc. (2), xx (1921), p. 326 Google Scholar.
- 2
- Cited by


