Hostname: page-component-cd9895bd7-dk4vv Total loading time: 0 Render date: 2024-12-29T08:13:49.500Z Has data issue: false hasContentIssue false
  • English
  • Français

The cyclicity of the period annulus of a reversible quadratic system

Part of: Qualitative theory Local and nonlocal bifurcation theory

Published online by Cambridge University Press:  10 February 2021

Changjian Liu ,
Chengzhi Li  and
Jaume Llibre
Changjian Liu
Affiliation:
School of Mathematics (Zhuhai), Sun Yat-sen University, Zhuhai519086, China ([email protected])
Chengzhi Li
Affiliation:
School of Mathematical Sciences, Peking University, Beijing100871, China ([email protected])
Jaume Llibre
Affiliation:
Departament de Matemàtiques, Universitat Autònoma de Barcelona, 08193 Bellaterra, Barcelona, Catalonia, Spain ([email protected])
Get access Rights & Permissions [Opens in a new window]

Abstract

We prove that perturbing the periodic annulus of the reversible quadratic polynomial differential system 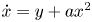$\dot x=y+ax^2$, $\dot y=-x$ with a ≠ 0 inside the class of all quadratic polynomial differential systems we can obtain at most two limit cycles, including their multiplicities. Since the first integral of the unperturbed system contains an exponential function, the traditional methods cannot be applied, except in Figuerasa, Tucker and Villadelprat (2013, J. Diff. Equ., 254, 3647–3663) a computer-assisted method was used. In this paper, we provide a method for studying the problem. This is also the first purely mathematical proof of the conjecture formulated by Dumortier and Roussarie (2009, Discrete Contin. Dyn. Syst., 2, 723–781) for q ⩽ 2. The method may be used in other problems.

Keywords

Perturbation of quadratic reversible centreAbelian integrallimit cycle

MSC classification

Primary: 34C07: Theory of limit cycles of polynomial and analytic vector fields (existence, uniqueness, bounds, Hilbert's 16th problem and ramifications)
Secondary: 34C08: Connections with real algebraic geometry (fewnomials, desingularization, zeros of Abelian integrals, etc.) 37G15: Bifurcations of limit cycles and periodic orbits
Type
Research Article
Information
Proceedings of the Royal Society of Edinburgh Section A: Mathematics , Volume 152 , Issue 2 , April 2022 , pp. 281 - 290
Check for updates
Copyright
Copyright © The Author(s), 2021. Published by Cambridge University Press on behalf of The Royal Society of Edinburgh

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Arnold, V. I.. Loss of stability of self-oscillations close to resonance and versal deformations of equivariant vector fields. Funct. Anal. Appl. 11 (1977), 85–92.CrossRefGoogle Scholar
Arnold, V. I.. Ten problems. Adv. Soviet Math. 1 (1990), 1–8.Google Scholar
Christopher, C. and Li, C.. Limit Cycles in Differential Equations (Boston: Birkhäuser, 2007).Google Scholar
Dumortier, F., Llibre, J. and Artés, J. C.. Qualitative Theory of Planar Differential Systems (Spring-Verlag: Universitext, 2006).Google Scholar
Dumortier, F. and Roussarie, R.. Birth of canard cycles. Discrete Contin. Dyn. Syst. 2 (2009), 723–781.Google Scholar
Figuerasa, J., Tucker, W. and Villadelprat, J.. Computer-assisted techniques for the verification of the Chebyshev property of Abelian integrals. J. Diff. Equ. 254 (2013), 3647–3663.Google Scholar
Iliev, I. D.. Perturbation of quadratic centers. Bull. Sci. Math. 122 (1998), 107–161.CrossRefGoogle Scholar