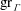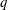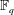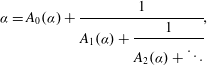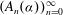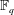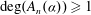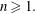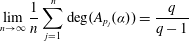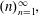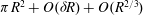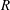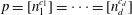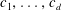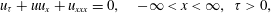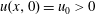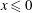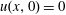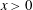Research Article
EXPLICIT BOUNDS ON AUTOMORPHIC AND CANONICAL GREEN FUNCTIONS OF FUCHSIAN GROUPS
- Part of:
-
- Published online by Cambridge University Press:
- 14 May 2014, pp. 257-306
-
- Article
- Export citation
ON THE METRIC THEORY OF CONTINUED FRACTIONS IN POSITIVE CHARACTERISTIC
- Part of:
-
- Published online by Cambridge University Press:
- 27 May 2014, pp. 307-320
-
- Article
-
- You have access
- Export citation
THE TILED CIRCLE PROBLEM
- Part of:
-
- Published online by Cambridge University Press:
- 31 March 2014, pp. 321-346
-
- Article
- Export citation
THE INTERSECTION OF PIATETSKI-SHAPIRO SEQUENCES
- Part of:
-
- Published online by Cambridge University Press:
- 01 April 2014, pp. 347-362
-
- Article
- Export citation
MULTIPLE EXPONENTIAL AND CHARACTER SUMS WITH MONOMIALS
- Part of:
-
- Published online by Cambridge University Press:
- 27 May 2014, pp. 363-373
-
- Article
- Export citation
AN AFFINE FOURIER RESTRICTION THEOREM FOR CONICAL SURFACES
- Part of:
-
- Published online by Cambridge University Press:
- 13 December 2013, pp. 374-390
-
- Article
- Export citation
THE LARGE-TIME DEVELOPMENT OF THE SOLUTION TO AN INITIAL-VALUE PROBLEM FOR THE KORTEWEG–DE VRIES EQUATION. II. INITIAL DATA HAS A DISCONTINUOUS COMPRESSIVE STEP
- Part of:
-
- Published online by Cambridge University Press:
- 14 May 2014, pp. 391-414
-
- Article
- Export citation
THE FINITE SECTION METHOD FOR DISSIPATIVE OPERATORS
- Part of:
-
- Published online by Cambridge University Press:
- 14 May 2014, pp. 415-443
-
- Article
- Export citation
CONTRACTIVE FAMILIES ON COMPACT SPACES
- Part of:
-
- Published online by Cambridge University Press:
- 14 May 2014, pp. 444-462
-
- Article
- Export citation
THE ERDŐS–SZEKERES PROBLEM FOR NON-CROSSING CONVEX SETS
- Part of:
-
- Published online by Cambridge University Press:
- 14 May 2014, pp. 463-484
-
- Article
- Export citation
A LIMITATION OF THE ESTIMATION OF INTRINSIC VOLUMES VIA PIXEL CONFIGURATION COUNTS
- Part of:
-
- Published online by Cambridge University Press:
- 28 March 2014, pp. 485-511
-
- Article
- Export citation
Front Cover (OFC, IFC) and matter
MTK volume 60 Issue 2 Cover and Front matter
-
- Published online by Cambridge University Press:
- 10 July 2014, pp. f1-f2
-
- Article
-
- You have access
- Export citation
Back Cover (OBC, IBC) and matter
MTK volume 60 Issue 2 Cover and Back matter
-
- Published online by Cambridge University Press:
- 10 July 2014, pp. b1-b3
-
- Article
-
- You have access
- Export citation