Hostname: page-component-cd9895bd7-7cvxr Total loading time: 0 Render date: 2024-12-27T07:04:20.286Z Has data issue: false hasContentIssue false
  • English
  • Français

CONTRACTIVE FAMILIES ON COMPACT SPACES

Part of: Nonlinear operators and their properties Spaces with richer structures

Published online by Cambridge University Press:  14 May 2014

Luka Milićević
Luka Milićević*
Affiliation:
Trinity College, Cambridge CB2 1TQ,U.K. email [email protected]
Get access

Abstract

A family 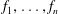$\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}f_1,\ldots,f_n$ of operators on a complete metric space 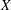$X$ is called contractive if there exists $\lambda < 1$ such that for any 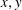$x,y$ in 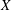$X$ we have 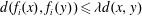$d(f_i(x),f_i(y)) \leq \lambda d(x,y)$ for some 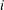$i$. Stein conjectured that for any contractive family there is some composition of the operators $f_i$ that has a fixed point. Austin gave a counterexample to this, and asked whether Stein’s conjecture is true if we restrict to compact spaces. Our aim in this paper is to show that, even for compact spaces, Stein’s conjecture is false.

MSC classification

Secondary: 47H09: Contraction-type mappings, nonexpansive mappings, $A$-proper mappings, etc. 54E45: Compact (locally compact) metric spaces
Type
Research Article
Information
Mathematika , Volume 60 , Issue 2 , July 2014 , pp. 444 - 462
Copyright
Copyright © University College London 2014 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Austin, T., On contractive families and a fixed-point question of Stein. Mathematika 52(1–2) 2005, 115–129.Google Scholar
Banach, S., Sur les opérations dans les ensembles abstraits et leur application aux équations intégrales. Fund. Math. 3 1922, 133–181.Google Scholar
Merryfield, J., Rothschild, B. and Stein, J. D. Jr, An application of Ramsey’s theorem to the Banach contraction mapping principle. Proc. Amer. Math. Soc. 130(4) 2002, 927–933.CrossRefGoogle Scholar
Merryfield, J. and Stein, J. D. Jr, A generalization of the Banach contraction mapping theorem. J. Math. Anal. Appl. 273(1) 2002, 112–120.CrossRefGoogle Scholar
Stein, J. D. Jr, A systematic generalization procedure for fixed-point theorems. Rocky Mountain J. Math. 30(2) 2000, 735–754.Google Scholar