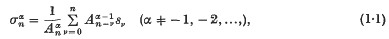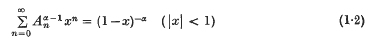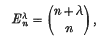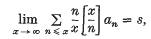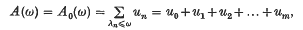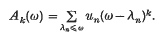Research Article
On a conjecture of Hughes
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 647-652
-
- Article
- Export citation
Asymptotic formula in a generalized Waring's problem
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 87-98
-
- Article
- Export citation
Finite approximations to infinite non-negative matrices
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 983-992
-
- Article
- Export citation
On operators which depend on some parameter
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 359-366
-
- Article
- Export citation
An extreme point theorem for inverses in a Banach algebra with identity
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 993-994
-
- Article
- Export citation
Abstract Köthe Spaces. I
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 653-660
-
- Article
- Export citation
Corrigendum
Singular measures with absolutely continuous convolution squares.†(Corrigendum)
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 367-368
-
- Article
-
- You have access
- Export citation
Research Article
Summability fields which span the bounded sequences
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 99-106
-
- Article
- Export citation
An intersection theorem of Erdős and Rado
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 995-996
-
- Article
- Export citation
On absolute summability factors of infinite series and their application to Fourier series
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 107-118
-
- Article
- Export citation
A note on ideals of homological dimension one in local domains
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 661-662
-
- Article
- Export citation
On quadrics through five real points
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 369-388
-
- Article
- Export citation
Janko's group as a collineation group in PG(6,11)
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 663-677
-
- Article
- Export citation
Strong summability of infinite series on a scale of Abel type summability methods
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 119-127
-
- Article
- Export citation
On topological sequence spaces
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 997-1019
-
- Article
- Export citation
On the pseudoprimes of the form ax + b
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 389-392
-
- Article
- Export citation
On the infinitesimal generator of the Poisson operator II. The n-dimensional case
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 1021-1025
-
- Article
- Export citation
A criterion for the planarity of the total graph of a graph*
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 679-681
-
- Article
- Export citation
Summability by Dirichlet convolutions
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 393-400
-
- Article
- Export citation
On the convergence factor of a Fourier series
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 129-132
-
- Article
- Export citation

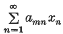 and
and 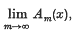 if that limit exists. We shall denote by
if that limit exists. We shall denote by 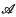 the set of bounded sequences which are summed by
the set of bounded sequences which are summed by  then we say that
then we say that 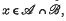 then
then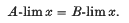
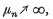 we say that
we say that 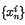 is summed by
is summed by 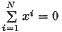 implies that
implies that 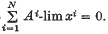 The set of sequences,
The set of sequences, 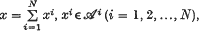 is denoted by
is denoted by 
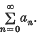 .
.