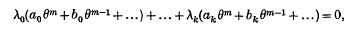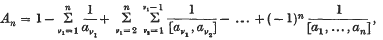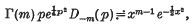Research Article
Quelques Remarques à Propos D'Une Note De G. H. Hardy: The Resultant of two Fourier Kernels (1)
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 413-418
-
- Article
- Export citation
A symmetrical configuration of n + 1 rational normal curves in [2n]
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 293-300
-
- Article
- Export citation
On a theorem of Paley and Wiener
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 1-5
-
- Article
- Export citation
The envelope of the subspaces of the polyhedra of an involution on a rational curve
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 183-187
-
- Article
- Export citation
Projective characters and invariants of algebraic varieties
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 188-198
-
- Article
- Export citation
On the easier Waring problem for powers of primes. I
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 6-12
-
- Article
- Export citation
Note on a Theorem of Myrberg
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 419-424
-
- Article
- Export citation
The postulation of a multiple surface
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 301-305
-
- Article
- Export citation
Regular canonical surfaces of genus three and four
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 306-310
-
- Article
- Export citation
Intersections of Loci on an Algebraic V4
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 425-437
-
- Article
- Export citation
On the extension of the Pflastersatz (Part II)
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 13-20
-
- Article
- Export citation
On certain surfaces which have unpostulated singularities
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 199-206
-
- Article
- Export citation
Note on the Invariants of the Canonical System of an Algebraic Variety
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 438-443
-
- Article
- Export citation
On an inequality in the elementary theory of numbers
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 207-209
-
- Article
- Export citation
On certain Cremona transformations in circle-space connected with the Miquel-Clifford configuration
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 21-25
-
- Article
- Export citation
On the canonical systems of certain algebraic surfaces
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 311-314
-
- Article
- Export citation
On the theorem of Riemann-Roch
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 26-34
-
- Article
- Export citation
Abstract definitions for the symmetry groups of the regular polytopes, in terms of two generators. Part II: the rotation groups
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 315-324
-
- Article
- Export citation
An infinite integral involving Bessel functions and parabolic cylinder functions
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 210-211
-
- Article
- Export citation
On the Smoothing of Observed Data
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 444-450
-
- Article
- Export citation