Research Article
A note on system normalizers of a finite soluble group
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 339-346
-
- Article
- Export citation
Minimal verbal subgroups
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 347-350
-
- Article
- Export citation
On the Mathieu group of degree twelve
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 351-364
-
- Article
- Export citation
A periodicity theorem in homological algebra
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 365-377
-
- Article
- Export citation
Spectral synthesis on spheres
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 379-387
-
- Article
- Export citation
Inclusion sets of regular summability matrices. III
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 389-394
-
- Article
- Export citation
On projective spaces over two skew fields
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 395-398
-
- Article
- Export citation
Singular measures with absolutely continuous convolution squares
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 399-420
-
- Article
- Export citation
On the consistency and relative strength of regular summability methods
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 421-428
-
- Article
- Export citation
On the least non-residue of a quartic polynomial
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 429-431
-
- Article
- Export citation
Binary integral quadratic forms over R(t)
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 433-440
-
- Article
- Export citation
The expansion of Lamé functions into series of associated Legendre functions of the second kind
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 441-452
-
- Article
- Export citation
Evaluation of an integral containing Bessel functions
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 453-457
-
- Article
- Export citation
Some formulae for Jacobi polynomials
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 459-462
-
- Article
- Export citation
On the Feynman integral for classical waves
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 463-465
-
- Article
- Export citation
An inversion formula for the Varma transform
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 467-471
-
- Article
- Export citation
Certain product theorems for bilateral hypergeometric series
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 473-475
-
- Article
- Export citation
On integral operators with kernels involving Bessel functions
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 477-484
-
- Article
- Export citation
Identifiability of countable mixtures of discrete probability distributions using methods of infinite matrices
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 485-494
-
- Article
- Export citation
Rotating fluid masses in general relativity. II
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 495-501
-
- Article
- Export citation

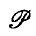 of soluble groups with self-normalizing system normalizers introduced by Carter in (1), while Theorem 2 shows the same is true for
of soluble groups with self-normalizing system normalizers introduced by Carter in (1), while Theorem 2 shows the same is true for 
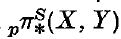 is built up from the
is built up from the 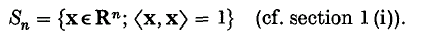
 will denote the set of bounded sequences which are summed by
will denote the set of bounded sequences which are summed by  consists of every bounded sequence then we shall say that the matrices
consists of every bounded sequence then we shall say that the matrices 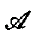 then we denote the value to which
then we denote the value to which 
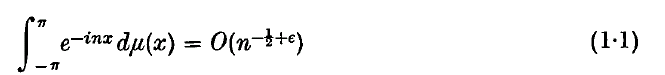
 For general locally compact Abelian groups, no exact analogue of (1 · 1) seems possible, as the character group may admit no natural order. However, it makes good sense to ask if μ* μ is absolutely continuous and has
For general locally compact Abelian groups, no exact analogue of (1 · 1) seems possible, as the character group may admit no natural order. However, it makes good sense to ask if μ* μ is absolutely continuous and has  for all real
for all real 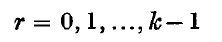


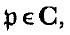 , the lattices
, the lattices 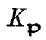 and
and 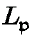 are in the same class. We mean to count the number of classes of lattices in a given genus over a binary space: we shall find that it is either 1 or
are in the same class. We mean to count the number of classes of lattices in a given genus over a binary space: we shall find that it is either 1 or 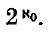 .
.
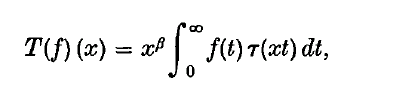
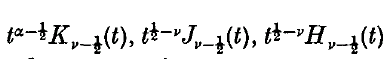 and
and 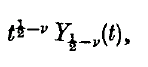 and in the last three cases the integrals converge in norm.
and in the last three cases the integrals converge in norm.