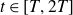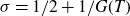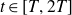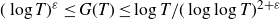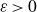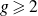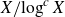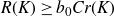Research Article
Centralisers of linear growth automorphisms of free groups
- Part of:
-
- Published online by Cambridge University Press:
- 20 September 2024, pp. 219-240
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
A study of the Ganea conjecture for topological complexity by using weak topological complexity
- Part of:
-
- Published online by Cambridge University Press:
- 21 October 2024, pp. 241-261
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Homotopy classification of 4-manifolds with finite abelian 2-generator fundamental groups
- Part of:
-
- Published online by Cambridge University Press:
- 08 November 2024, pp. 263-283
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Graded extensions of Verma modules
- Part of:
-
- Published online by Cambridge University Press:
- 08 November 2024, pp. 285-311
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Discrepancy bounds for the distribution of L-functions near the critical line
- Part of:
-
- Published online by Cambridge University Press:
- 21 October 2024, pp. 313-332
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Special homogeneous surfaces
- Part of:
-
- Published online by Cambridge University Press:
- 22 October 2024, pp. 333-362
-
- Article
-
- You have access
- HTML
- Export citation
Most integers are not a sum of two palindromes
- Part of:
-
- Published online by Cambridge University Press:
- 22 October 2024, pp. 363-366
-
- Article
-
- You have access
- HTML
- Export citation
The ropelength conjecture of alternating knots
-
- Published online by Cambridge University Press:
- 25 November 2024, pp. 367-369
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Front Cover (OFC, IFC) and matter
PSP volume 177 issue 2 Cover and Front matter
-
- Published online by Cambridge University Press:
- 05 December 2024, pp. f1-f2
-
- Article
-
- You have access
- Export citation
Back Cover (OBC, IBC) and matter
PSP volume 177 issue 2 Cover and Back matter
-
- Published online by Cambridge University Press:
- 05 December 2024, pp. b1-b2
-
- Article
-
- You have access
- Export citation