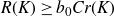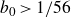The ropelength conjecture of alternating knots

Abstract

A long standing conjecture states that the ropelength of any alternating knot is at least proportional to its crossing number. In this paper we prove that this conjecture is true. That is, there exists a constant $b_0 \gt 0$ such that $R(K)\ge b_0Cr(K)$ for any alternating knot K, where R(K) is the ropelength of K and Cr(K) is the crossing number of K. In this paper, we prove that this conjecture is true and establish that $b_0 \gt 1/56$.

1. Introduction

The ropelength of a knot K is defined as the minimum length among all knots in the ambient isotopic class of K with unit thickness [ 3, 8, 11 ]. A fundamental question in geometric knot theory asks how the ropelength R(K) of a knot K is related to Cr(K), the crossing number of K [ 1–4, 6 ]. In the particular case that K is an alternating knot, a long standing conjecture, well known at least to researchers who study the ropelength problem, states that the ropelength of K is at least proportional to Cr(K). We prove that this conjecture is true. More specifically, we prove that there exists a constant $b_0 \gt 1/56$ such that $R(K) \gt b_0Cr(K)$ for any alternating knot K.

2. Reverse parallel links of alternating knots

Let K be a knot in $\textbf{S}^3$ . The regular neighbourhood of K on any orientable surface M embedded in $\textbf{S}^3$ on which K lies is an annulus. Let A be such an annulus. The link formed by the boundaries $K^\prime$ , $K^{\prime\prime}$ of A is called a reverse parallel link of K if $K^\prime$ , $K^{\prime\prime}$ are assigned opposite orientations. The linking number f between $K^\prime$ and $K^{\prime\prime}$ when they are assigned parallel orientations is called the framing of K [ 12, 13 ]. The framing f is independent of the orientation of K and the ambient isotopy class of A in $S^3$ depends only on K and the framing. Furthermore, the reverse parallel links of K are characterised by their framing numbers. That is, two reverse parallel links of K are ambient isotopic if and only if they have the same linking number. We shall denote a reverse parallel link of K with framing f by $\mathbb{K}_f$ following [ 9 ].

Let K be an alternating knot with a reduced diagram D. Let c(D) be the number of crossings in D and w(D) the writhe of D. It is known that $c(D)=Cr(K)$ is the crossing number of K. Also, all crossings of D are positive with respect to one checkerboard shading of D and are all negative with respect to the other checkerboard shading of D. Let $v_+(D)$ be the number of regions in the shading with respect to which all crossings are positive, and $v_-(D)$ be the number of regions in the complementary shading with respect to which all crossings are negative, then $v_+(D)+v_-(D)=c(D)+2$ [ 10 ]. The following results provide the key to solving the ropelength conjecture for alternating knots.

Theorem 2·1. [ 9 ] Let K be an alternating knot and D a reduced diagram of K. Let c(D), w(D), $v_+(D)$ and $v_-(D)$ be as defined above, then the braid index of $\mathbb{K}_f$ , denoted by $\mathbf{b}(\mathbb{K}_f)$ , is given by the following formula:

\begin{equation}\mathbf{b}(\mathbb{K}_f)=\left\{\begin{array}{ll}c(D)+2+a(D)-f, &\ {\rm if}\ f \lt a(D),\\[5pt] c(D)+2, &\ {\rm if}\ a(D)\le f \le b(D),\\[5pt] c(D)+2-b(D)+f, &\ {\rm if}\ f \gt b(D),\\ \end{array}\right.\end{equation}

where $a(D)=-v_-(D)+w(D)$ and $b(D)=v_+(D)+w(D)$ . In particular, $\mathbf{b}(\mathbb{K}_f)\ge c(D)+2$ for any f.

Theorem 2·2. [ 5 ] Let L be an oriented link and $\mathbf{b}(L)$ be its braid index. Then $\ell(L_c)\ge \mathbf{b}(L)$ where $\ell(L_c)$ is the minimum length among all links in the ambient isotopic class of L that are embedded in the cubic lattice of $\mathbb{R}^3$ .

3. The ropelengths of alternating knots

Theorem 3·1. There exists a constant $b_0 \gt 1/56$ such that for any alternating knot K, $R(K)\ge b_0 Cr(K)$ .

Proof. Let $K_c$ be a knot on the cubic lattice that is ambient isotopic to K and has the minimum length among all lattice knots in the ambient isotopic class of K. Let $\ell(K_c)$ be the length of $K_c$ , then we have $R(K) \gt ({1}/{14})\ell(K_c)$ as a consequence from the proof of [ 7 , lemma 1].

Now, the set $\{\textbf{x}+t(({1}/{2}, {1}/{2}, {1}/{2}): \textbf{x}\in K_c,\ 0\le t\le 1\}$ is an embedding of the annulus $\{1\le x^2+y^2\le 2:\ x, y\in \mathbb{R}\}$ into $\mathbb{R}^3$ with $K_c$ and $K_c^\prime=(({1}/{2}, {1}/{2}, {1}/{2}))+K_c$ as its boundary curves. Assigning $K_c$ and $K_c^\prime$ opposite orientations yields a reverse parallel link $L_c$ of K. Let $\mathbb{K}_c$ and $\mathbb{K}^\prime_c$ be the lattice knots obtained from $K_c$ and $K_c^\prime$ by scaling up with a scaling factor of 2, and let $\mathbb{L}_c$ be the link formed by them. Notice that $\ell(\mathbb{L}_c)=4\ell(K_c)$ . By Theorem 2·1, we have $\mathbf{b}(\mathbb{L}_c)\ge Cr(K)+2$ . Then by Theorem 2·2, we have $\ell(K_c)\ge (Cr(K)+2)/4$ . Therefore $R(K) \gt ({1}/{14})\ell(K_c)\ge (Cr(K)+2)/56$ .

Remark 3·2. The construction in the proof of [ 7 , lemma 1] can in fact be improved to yield a better bound $R(K) \gt ({1}/{12})\ell(K_c)$ . This would allow us to improve the estimation of the constant $b_0$ to be at least $1/48$ . It is certainly possible to further improve $b_0$ by not using the lattice knots as a mid step.

Remark 3·3. Theorem 2·1 only applies to alternating knots, therefore the ropelength conjecture remains open in general for alternating links with two or more components.