Research Article
Short intervals containing numbers without large prime factors
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 1-5
-
- Article
- Export citation
The syzygies of m-full ideals
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 7-13
-
- Article
- Export citation
Some inequalities concerning immanants
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 15-30
-
- Article
- Export citation
Kähler groups and rigidity phenomena
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 31-44
-
- Article
- Export citation
The Pompeiu problem for groups
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 45-58
-
- Article
- Export citation
Hopf algebras and regular isotopy invariants for link diagrams
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 59-77
-
- Article
- Export citation
On groups associated to a knot
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 79-82
-
- Article
- Export citation
Jones polynomial invariants for knots and satellites
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 83-103
-
- Article
- Export citation
Minimal atlases on 3-manifolds
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 105-115
-
- Article
- Export citation
A torus reduction theorem for regular coverings of 3-manifolds by homology 3-spheres
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 117-124
-
- Article
- Export citation
On parallelepipeds of minimal volume containing a convex symmetric body in ℝn
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 125-148
-
- Article
- Export citation
Essentially unbounded chains in compact sets
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 149-160
-
- Article
- Export citation
On the reciprocal Dunford-Pettis property in projective tensor products
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 161-166
-
- Article
- Export citation
Bicompleteness of the fine quasi-uniformity
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 167-186
-
- Article
- Export citation
Lindelöf locales and ℕ-compactness
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 187-191
-
- Article
- Export citation
The number of infinite substructures
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 193-209
-
- Article
- Export citation
The horizon problem for random surfaces
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 211-219
-
- Article
- Export citation
A stochastic calculus approach to the shape distribution induced by a complex normal model
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 221-228
-
- Article
- Export citation
Front matter
PSP volume 109 issue 1 Cover and Front matter
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. f1-f2
-
- Article
-
- You have access
- Export citation
Back matter
PSP volume 109 issue 1 Cover and Back matter
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. b1-b2
-
- Article
-
- You have access
- Export citation

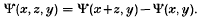
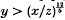 in [
in [ of fundamental groups of non-singular complex projective varieties is an interesting, but as yet imperfectly understood, class of finitely presented groups. Membership of
of fundamental groups of non-singular complex projective varieties is an interesting, but as yet imperfectly understood, class of finitely presented groups. Membership of 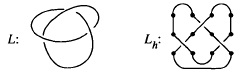
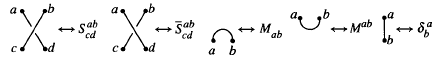
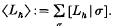
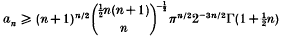
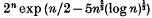
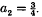 . We show that
. We show that 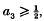 , and that a local minimum of the function
, and that a local minimum of the function  ℕ which are ⊆ *-unbounded in their topological closures.
ℕ which are ⊆ *-unbounded in their topological closures.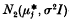 -random points (
-random points (