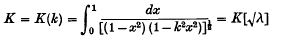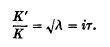Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Zucker, I. J.
1979.
The Summation of Series of Hyperbolic Functions.
SIAM Journal on Mathematical Analysis,
Vol. 10,
Issue. 1,
p.
192.
Glasser, M.L.
and
Zucker, I.J.
1980.
Theoretical Chemistry.
p.
67.
Zucker, I. J.
and
Robertson, M. M.
1984.
Further aspects of the evaluation of σ(m. n≠0, 0) (am2 + bnm + cn2)−s.
Mathematical Proceedings of the Cambridge Philosophical Society,
Vol. 95,
Issue. 1,
p.
5.
Joyce, G. S.
and
Zucker, I. J.
1991.
Special values of the hypergeometric series.
Mathematical Proceedings of the Cambridge Philosophical Society,
Vol. 109,
Issue. 2,
p.
257.
Berndt, Bruce C.
and
Chan, Heng Huat
1995.
Ramanujan's explicit values for the classical theta‐function.
Mathematika,
Vol. 42,
Issue. 2,
p.
278.
Berndt, Bruce C.
2001.
Special Functions 2000: Current Perspective and Future Directions.
p.
61.
Dowker, J.S.
and
Kirsten, Klaus
2002.
Elliptic functions and temperature inversion symmetry on spheres.
Nuclear Physics B,
Vol. 638,
Issue. 3,
p.
405.
Yi, Jinhee
2004.
Theta-function identities and the explicit formulas for theta-function and their applications.
Journal of Mathematical Analysis and Applications,
Vol. 292,
Issue. 2,
p.
381.
VIDUNAS, Raimundas
2005.
EXPRESSIONS FOR VALUES OF THE GAMMA FUNCTION.
Kyushu Journal of Mathematics,
Vol. 59,
Issue. 2,
p.
267.
Levin, Aaron
2005.
A New Class of Infinite Products Generalizing Viète's Product Formula for π.
The Ramanujan Journal,
Vol. 10,
Issue. 3,
p.
305.
Dowker, J. S.
and
Kirsten, Klaus
2008.
Elliptic aspects of statistical mechanics on spheres.
Journal of Mathematical Physics,
Vol. 49,
Issue. 11,
Zucker, I. J.
2011.
70+ Years of the Watson Integrals.
Journal of Statistical Physics,
Vol. 145,
Issue. 3,
p.
591.
Chamberland, Marc
and
Straub, Armin
2013.
On gamma quotients and infinite products.
Advances in Applied Mathematics,
Vol. 51,
Issue. 5,
p.
546.
Mingari Scarpello, Giovanni
and
Ritelli, Daniele
2014.
Legendre hyperelliptic integrals, π new formulae and Lauricella functions through the elliptic singular moduli.
Journal of Number Theory,
Vol. 135,
Issue. ,
p.
334.
Rogers, M.
Wan, J. G.
and
Zucker, I. J.
2015.
Moments of elliptic integrals and critical $$L$$ L -values.
The Ramanujan Journal,
Vol. 37,
Issue. 1,
p.
113.
Joyce, G S
2016.
On the Laurent series for the Epstein zeta function.
Journal of Physics A: Mathematical and Theoretical,
Vol. 49,
Issue. 40,
p.
405204.
Chen, Parry Y.
Smith, Michael J. A.
and
McPhedran, Ross C.
2018.
Evaluation and regularization of phase-modulated Eisenstein series and application to double Schlömilch-type sums.
Journal of Mathematical Physics,
Vol. 59,
Issue. 7,
Rebák, Örs
2023.
The three missing terms in Ramanujan’s septic theta function identity.
The Ramanujan Journal,
Vol. 60,
Issue. 4,
p.
885.
Berndt, Bruce C.
and
Rebák, Örs
2025.
Cubic and quintic analogues of Ramanujan’s septic theta function identity.
The Ramanujan Journal,
Vol. 66,
Issue. 4,