Hostname: page-component-cd9895bd7-8ctnn Total loading time: 0 Render date: 2024-12-28T11:11:45.505Z Has data issue: false hasContentIssue false
  • English
  • Français

Bounds for the solution of a non-linear parabolic initial-boundary value problem

Published online by Cambridge University Press:  24 October 2008

M. R. Carter
M. R. Carter
Affiliation:
Massey University, Palmerston North, New Zealand
Get access Rights & Permissions [Opens in a new window]

Extract

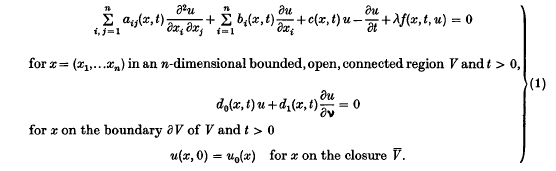
A number of papers have appeared over the past decade or so which study questions of the existence and stability of positive steady-state solutions for parabolic initial-boundary value problems of the general form

Type
Research Article
Information
Mathematical Proceedings of the Cambridge Philosophical Society , Volume 82 , Issue 1 , July 1977 , pp. 131 - 145
Copyright
Copyright © Cambridge Philosophical Society 1977

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

(1)Amann, H.Multiple positive fixed points of asymptotically linear maps. J. Functional Analysis 17 (1974), 174–213.CrossRefGoogle Scholar
(2)Amann, H.Supersolutions, monotone iterations and stability. J. Differential Equations 21 (1976), 363–377.CrossRefGoogle Scholar
(3)Amann, H.Fixed point equations and nonlinear eigenvalue problems in ordered Banach spaces. Siam Review 18 (1976), 620–709.CrossRefGoogle Scholar
(4)Boddington, T., Gray, P. and Harvey, D. I.Thermal theory of spontaneous ignition: criticality in bodies of arbitrary shape. Philos. Trans. R. Soc. London A 270 (1971), 467–506.Google Scholar
(5)Browder, F. E.Linear parabolic differential equations of arbitrary order; general boundary-value problems for elliptic equations. Proc. Nat. Acad. Sci. U.S.A. 39 (1953), 185–190. Also errata p. 298.CrossRefGoogle ScholarPubMed
(6)Carter, M. R. A non-linear boundary value problem arising in the theory of thermal explosions. Ph.D. thesis, Massey University, Palmerston North, New Zealand, 1976.Google Scholar
(7)Chan, C. Y.Positive solutions for nonlinear parabolic second initial boundary value problems. Quart. Appl. Math. 31 (1974), 443–454.CrossRefGoogle Scholar
(8)Cohen, D. S.Multiple stable solutions of non-linear boundary value problems arising in chemical reactor theory. Siam J. Appl. Math. 20 (1971), 1–13.CrossRefGoogle Scholar
(9)Hunjaev, S. I.Boundary problems for certain quasi-linear elliptic equations. Soviet Math. Dokl. 5 (1964), 188–192.Google Scholar
(10)Keener, J. P. and Keller, H. B.Positive solutions of convex non-linear eigenvalue problems. J. Differential Equations 16 (1974), 103–125.CrossRefGoogle Scholar
(11)Keller, H. B. and Cohen, D. S.Some positone problems suggested by non-linear heat generation. J. Math. Mech. 16 (1967), 1361–1376.Google Scholar
(12)Krein, M. G. and Rutman, M. A.Linear operators leaving invariant a cone in a Banach space. Amer. Math. Soc. Transi., Ser. 1, 10 (1962), 199–325.Google Scholar
(13)McNabb, A.Comparison and existence theorems for multicomponent diffusion systems. J. Math. Anal. Appl. 3 (1961), 133–144.CrossRefGoogle Scholar
(14)Parter, S. V.Solutions of a differential equation arising in chemical reactor processes. Siam J. Appl. Math. 26 (1974), 687–716.CrossRefGoogle Scholar
(15)Sattinger, D. H.Monotone methods in non-linear elliptic and parabolic boundary value problems. Indiana Univ. Math. J. 21 (1972), 979–1000.CrossRefGoogle Scholar