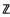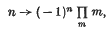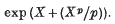Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Diamond, Jack
1984.
Number Theory.
Vol. 1052,
Issue. ,
p.
168.
Conrad, Keith
2000.
A q-Analogue of Mahler Expansions, I.
Advances in Mathematics,
Vol. 153,
Issue. 2,
p.
185.
Narkiewicz, Władysław
2012.
Rational Number Theory in the 20th Century.
p.
307.
Shapiro, Ilya
2012.
Frobenius map and the p -adic Gamma function.
Journal of Number Theory,
Vol. 132,
Issue. 8,
p.
1770.
Menken, Hamza
and
Körükçü, Adviye
2013.
Some Properties of the -Extension of the -Adic Gamma Function.
Abstract and Applied Analysis,
Vol. 2013,
Issue. ,
p.
1.
Chatterjee, Tapas
and
Garg, Sonam
2024.
Transcendental nature of p-adic digamma values.
Research in Number Theory,
Vol. 10,
Issue. 4,