Hostname: page-component-cd9895bd7-gvvz8 Total loading time: 0 Render date: 2024-12-25T09:05:07.763Z Has data issue: false hasContentIssue false
  • English
  • Français

Two non-linear birth and death processes

Published online by Cambridge University Press:  09 April 2009

J. A. Bather
J. A. Bather
Affiliation:
Statistical Laboratory, University of Cambridge.
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

The random processes discussed here may be specified in the following way. A fixed population of N members is spilt into two distinct classes. Individuals move about randomly between the classes, and we are interested in the size of each class at any time, rather than in the behaviour of particular individuals. Let i(t) and N —i(t) be the numbers present in the repective classes at the time t. It is assumed that the process {i(t), t ≧ 0} is Markovian, and that transitions between the states j = 0, 1, … N, occur according to the conditional probabilities;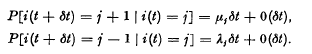 and.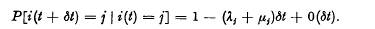

Type
Research Article
Information
Journal of the Australian Mathematical Society , Volume 3 , Issue 1 , February 1963 , pp. 104 - 116
Copyright
Copyright © Australian Mathematical Society 1963

References

[1]Ledermann, W. and Reuter, G. E. H.Spectral theory for the differential equqt ions of simple birth and death processes. Phil. Trans. Roy. Soc. London. A. Vol. 246, No. 914, 1954, p. 321.Google Scholar
[2]Moran, P. A. P.Randon Precesses in genetics. Proc. Camb. Philos. Soc. Vol 54. 1958, p. 60.CrossRefGoogle Scholar
[3]Bartlett, M. S.Some evolutionary stochastic processes. J. R. S. S. B. Vol XI, No 2, 1949, p. 220. Note added in proof: For a more direct determination of the eigenvalues for Moran's model, the reader is referred to: J. Gani On the stochastic matrix in a genetic model of Moran Biomerika. Vol 48, 1961, p. 203.Google Scholar