Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Bélair, Luc
and
Haskell, Deirdre
1996.
Dependence on parameters in analogues of hilberts seventeenth problem.
Communications in Algebra,
Vol. 24,
Issue. 7,
p.
2407.
Guzy, Nicolas
2005.
p-Convexly valued rings.
Journal of Pure and Applied Algebra,
Vol. 199,
Issue. 1-3,
p.
111.
Lempp, Steffen
2008.
2007 European Summer Meeting of the Association for Symbolic Logic: Logic Colloquium '07.
Bulletin of Symbolic Logic,
Vol. 14,
Issue. 1,
p.
123.
Darnière, Luck
and
Tressl, Marcus
2020.
Defining integer-valued functions in rings of continuous definable functions over a topological field.
Journal of Mathematical Logic,
Vol. 20,
Issue. 03,
p.
2050014.


 (
(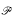 by a prime ideal
by a prime ideal 