We present here the first study on the stability of plane Poiseuille flow when the fluid is stratified in density perpendicularly to the plane of horizontal shear. Using laboratory experiments, linear stability analyses and direct numerical simulations, we describe the appearance of an instability that results from a resonance of internal gravity waves and Tollmien–Schlichting waves carried by the flow. This instability takes the form of long meanders confined in thin horizontal layers stacked along the vertical axis.

High-resolution simulations of temporally evolving mixing layers, for convective Mach numbers ranging from $M_c=0.2$ to $M_c=2.0$ with density ratios $s=1$ and $s=7$, are analysed to characterize compressibility effects on the structure and evolution of turbulence in this compressible flow. Published experimental results are used to validate simulation results. Examination of the turbulence scales in the present data suggests an internal regulation mechanism. Correlated eddying motions were found to be in support of a ‘sonic eddy hypothesis’. Eddy scales in all spatial directions are found to be a progressively smaller fraction of the overall mixing-layer thickness with increasing $M_c$, forming independent layers of eddying motions at high $M_c$. These reduced spatial scales serve to reduce the effective velocity scale for turbulent motions, suppressed Reynolds stresses, turbulent kinetic energy (TKE) production and dissipation, and the mixing-layer thickness growth rate.

Flapping-wing-based propulsion is ubiquitous in Nature, and it is free in all directions. In this work, the hydrodynamic behaviour of an unconstrained flapping foil, which can self-propel in both longitudinal and lateral directions, is numerically studied. It is found that the flapping foil can keep self-propelling in a straight line along the longitudinal direction, together with a passive oscillation in the lateral direction. Moreover, the effects of multiple parameters on the performance of the flapping swimmer are investigated, including the flapping frequency and amplitude, the mass ratio between foil and fluid, and the thickness–chord ratio of the foil. It is shown that the propulsive speed, the power consumption and the lateral oscillating motion obey some simple scaling laws. The results obtained here may provide some light on understanding biological flapping-wing-based propulsion.

This paper presents a quantitative evaluation of the Reynolds analogy factor $s=2c_h/c_f$ for self-similar turbulent boundary layers with pressure gradients in the streamwise direction via direct numerical simulation. Both sub- and supersonic cases are considered at nearly adiabatic wall conditions. The Reynolds analogy factor is found to be greatly increased for adverse-pressure-gradient cases and decreased for favourable-pressure-gradient cases. Although the boundary layers considered cover a comparatively large Reynolds-number range from small to moderate Reynolds numbers, no Reynolds-number dependency of $s$ is found in the parameter range investigated. Mach-number influences on $s$ are found to be small; $s$ decreases slightly with increasing Mach number. The influence of pressure gradients on $s$ turns out to be well approximated by the analytical relation derived by So (Intl J. Heat Mass Transfer, vol. 37, 1994, pp. 27–41) for incompressible flow if a fixed, calibrated Reynolds number is used. Moreover, the effects of non-self-similarity prior to the self-similar region are assessed.

Laminar flows through pipes driven at steady, pulsatile or oscillatory rates undergo a subcritical transition to turbulence. We carry out an extensive linear non-modal stability analysis of these flows and show that for sufficiently high pulsation amplitudes the stream-wise vortices of the classic lift-up mechanism are outperformed by helical disturbances exhibiting an Orr-like mechanism. In oscillatory flow, the energy amplification depends solely on the Reynolds number based on the Stokes-layer thickness, and for sufficiently high oscillation frequency and Reynolds number, axisymmetric disturbances dominate. In the high-frequency limit, these axisymmetric disturbances are exactly similar to those recently identified by Biau (J. Fluid Mech., vol. 794, 2016, R4) for oscillatory flow over a flat plate. In all regimes of pulsatile and oscillatory pipe flow, the optimal helical and axisymmetric disturbances are triggered in the deceleration phase and reach their peaks in typically less than a period. Their maximum energy gain scales exponentially with Reynolds number of the oscillatory flow component. Our numerical computations unveil a plausible mechanism for the turbulence observed experimentally in pulsatile and oscillatory pipe flow.

In this paper we study the receptivity of the boundary layer to suction/blowing in marginally separated flows, like the one on the leading edge of a thin aerofoil. We assume that the unperturbed laminar flow is two-dimensional, and investigate the response of the boundary layer to two-dimensional as well as to three-dimensional perturbations. In both cases, the perturbations are assumed to be weak and periodic in time. Unlike conventional boundary layers, the marginally separated boundary layers cannot be treated using the quasi-parallel approximation. This precludes the normal-mode representation of the perturbations. Instead, we had to solve the linearised integro-differential equation of the marginal separation theory, which was done numerically. For two-dimensional perturbations, the results of the calculations show that the perturbations first grow in the inside of the separation region, but then start to decay downstream. For three-dimensional perturbations, instead of dealing with the integro-differential equation of marginal separation, we found it convenient to work with the Fourier transforms of the fluid-dynamic functions. The equations for the Fourier transforms are also solved numerically. Our calculations show that a three-dimensional wave packet forms downstream of the source of perturbations in the boundary layer.

The mean flow induced by a three-dimensional propagating internal gravity wave beam in a uniformly stratified fluid is studied experimentally and theoretically. Previous related work concentrated on the early stage of mean-flow generation, dominated by the phenomenon of streaming – a horizontal mean flow that grows linearly in time – due to resonant production of mean potential vorticity in the vicinity of the beam. The focus here, by contrast, is on the long-time mean-flow evolution. Experimental observations in a stratified fluid tank for times up to $t=120T_0$, where $T_0$ is the beam period, reveal that the induced mean flow undergoes three distinct stages: (i) resonant growth of streaming in the beam vicinity; (ii) saturation of streaming and onset of horizontal advection; and (iii) establishment of a quasi-steady state where the mean flow is highly elongated and stretches in the along-tank horizontal direction. To capture (i)–(iii), the theoretical model of Fan et al. (J. Fluid Mech., vol. 838, 2018, R1) is extended by accounting for the effects of horizontal advection and viscous diffusion of mean potential vorticity. The predictions of the proposed model, over the entire mean-flow evolution, are in excellent agreement with the experimental observations as well as numerical simulations based on the full Navier–Stokes equations.

Surfactants can immobilize fluid–liquid interfaces under shear stress. We investigate the impact of insoluble surfactants on shear flow along a superhydrophobic surface in Cassie state, with gas trapped in grooves oriented perpendicular to the flow direction. Assuming convection-dominated transport along the gas–liquid interface, analytical results for the surfactant distribution on a groove and the corresponding flow field in its vicinity are derived both for a single groove and for an array of evenly spaced grooves. The results are elaborated for the case where the surface tension depends linearly on the surfactant concentration, which is characteristic for dilute coverage of the gas–liquid interface. For an array of grooves, the relation between the applied shear stress and the effective slip length on the microstructured surface is investigated.

We study the axisymmetric evolution of a liquid film on a solid sphere governed by gravity, capillarity and viscous forces. The lubrication equations established in spherical coordinates are numerically solved using finite elements and local similarity solutions are obtained. Results show that the evolution behaves differently at early and late stages. At the early stage, the interface evolves in such a way that the capillary effect can be ignored. At the late stage, there emerge four zones from top to bottom: a thin film, a ridge ring, a dimple ring and a pendant drop. Each zone is governed by the balance of different forces, and hence is characterized by an individual physical mechanism. Consequently, the pendant drop is quasi-static, and the film thicknesses of other regions follow different scaling laws. The position of the dimple remains unchanged at the late stage.

We consider resonant triad interactions between surface and internal gravity waves propagating in two horizontal dimensions in a two-layer system with a free surface. As the system supports both surface and internal wave modes, two different types of resonant triad interactions are possible: one with two surface and one internal wave modes and the other with one surface and two internal wave modes. The resonance conditions are studied in detail over a wide range of physical parameters (density and depth ratios). Explicitly identified are the spectral domains of resonance whose boundaries represent one-dimensional resonances (class I–IV). To study the nonlinear interaction between two-dimensional surface and internal waves, a spectral model is derived from an explicit Hamiltonian system for a two-layer system after decomposing the surface and interface motions into the two wave modes through a canonical transformation. For both types of resonances, the amplitude equations are obtained from the reduced Hamiltonian of the spectral model. Numerical solutions of the explicit Hamiltonian system using a pseudo-spectral method are presented for various resonance conditions and are compared with those of the amplitude equations.

A high-fidelity simulation of the shock/transitional boundary layer interaction caused by a $15^\circ$ axisymmetrical compression ramp is performed at a free stream Mach number of 5 and a transitional Reynolds number. The inlet of the computational domain is perturbed with a white noise in order to excite convective instabilities. Coherent structures are extracted using spectral proper orthogonal decomposition (SPOD), which gives a mathematically optimal decomposition of spatio-temporally correlated structures within the flow. The mean flow is used to perform a resolvent analysis in order to study non-normal linear amplification mechanisms. The comparison between the resolvent analysis and the SPOD results provides insight on both the linear and nonlinear mechanisms at play in the flow. To carry out the analysis, the flow is separated into three main regions of interest: the attached boundary layer, the mixing layer and the reattachment region. The observed transition process is dependent on the linear amplification of oblique modes in the boundary layer over a broad range of frequencies. These modes interact nonlinearly to create elongated streamwise structures which are then amplified by a linear mechanism in the rest of the domain until they break down in the reattachment region. The early nonlinear interaction is found to be essential for the transition process.

In the linear approximation, we study wave motions of a compressed elastic ice sheet caused by the motion of a two-dimensional dipole in the water beneath the sheet. The fluid flow is described by the potential theory, while the ice sheet is modelled through a thin elastic plate floating on the water surface. The solution for the vertical displacement of the ice sheet is derived for a transient dipole undergoing arbitrary two-dimensional motion. Three cases are considered in detail when the dipole moves horizontally with a uniform speed at some depth or horizontally oscillates, or moves and oscillates. The formulae for the plate displacement are derived for the fluid of finite depth, but then analysed in detail for the infinitely deep case. We show that the character of the solutions is different in the different domains of the parameter plane and classify the possible cases. Then we calculate the wave patterns on the plate for the different regimes of dipole motion and typical values of plate parameters. The studied problem can be considered as the simplified model of motion of a circular cylinder in a water under an ice cover. In the last section we compare the characteristics of wave motions onsetting in the far-field zone of the flow around a circular cylinder and its dipole approximation and show that the difference in the wave characteristics and force loads for these two cases is small and quickly vanishes when the ice plate thickness increases. In conclusion, we present estimates of amplitudes and wavelengths of wave perturbations for the real oceanic conditions.

Cross-stream migration of a droplet in an incipient flow turns out to be of outstanding importance in several emerging applications encompassing chemistry, engineering and biology. Here, we bring out the confluence of confinement, oscillatory axial pressure gradient and steady axial electric field towards controlling spatiotemporal characteristics of cross-stream migration of droplets in a micro-confined fluidic environment, bearing immense implications in in vitro modelling of bio-analytical procedures. Under the sole influence of an oscillatory axial pressure gradient, the time taken by a droplet to achieve a steady-state transverse position is significantly long and the direction of the droplet's motion cannot be altered at will. However, confinement-modulated electrohydrodynamic interactions enable overcoming this constraint, even when the applied electric field is orthogonal to the intended direction of droplet migration, a proposition that is not feasible in an unbounded domain. Our results reveal that depending on the relative electrical properties of the droplet and the carrier phases and a competing influence of electrical, viscous and capillary stresses, the rate of transverse migration can be controlled by effectively modulating the axial oscillations in its cross-stream motion. Beyond a threshold value of the applied electric field, simultaneous enhancement in the droplet migration rate and reversal in the direction of its lateral migration become possible, which cannot otherwise be achieved by the oscillatory pressure field alone. Furthermore, the oscillatory characteristics in the droplet migration can be dampened out completely by exploiting the addressed physical interplay. Results from in-house experiments corroborate our theoretical conjecture.

The mechanics of extreme intensity events in the buffer and logarithmic layers of a turbulent channel at $Re_\tau =2000$ is investigated. The 99.9th percentile of the most intense events in the dissipation of turbulent kinetic energy is analysed by means of conditional space–time proper orthogonal decomposition. The computed spatio-temporal modes are coherent in space and over the considered time frame, and optimally capture the energy of the ensemble. The most energetic mode with transverse symmetric structure describes a turbulent burst event. The underlying mechanism is a varicose instability which generates localized extrema in the dissipation and production of turbulent kinetic energy and drives the formation of a hairpin vortex. The most energetic anti-symmetric mode is related to a sinuous-type instability that is situated in the shear layer between two very-large-scale streaks. Statistical results show the energy in the symmetric mode to exceed that in the anti-symmetric mode by a near constant factor for the considered wall distances. Both mechanisms occur throughout the range of wall distances in an effectively self-similar manner that is consistent with the attached-eddy hypothesis. By analogy with transitional flows, the results suggest that the events are induced by an exponential growth mechanism.

The shapes of two steadily rotating, equal circulation, two-dimensional hollow vortices are determined and their properties examined. By means of a numerical scheme that accounts for the doubly connected nature of the fluid domain, it is shown that a one-parameter family of solutions exists that is a continuation of a corotating point-vortex pair. With $b=2$ set as the distance between the vortex centroids, we find that each vortex reaches a maximum possible area of $0.796$ corresponding to $a/b=0.260$ where $a$ is a measure of the vortex core radius proposed by Meunier et al. (Phys. Fluids, vol. 14, 2002, pp. 2757–2766). Results are compared to those of a previous study by Saffman & Szeto (Phys. Fluids, vol. 23, 1980, pp. 2339–2342) in which two corotating patches of uniform vorticity are considered in place of the hollow vortices studied here. The general behaviour of the two systems is seen to be similar but some differences are highlighted, especially when the vortices become close to touching due to the accumulation of vorticity in thin extended fingers emanating from each of the vortices. The numerical scheme captures the family of equilibria very close to a critical configuration where these fingers tend to touch at the centre of rotation corresponding to $a/b \approx 0.283$. By a simple adaptation of the numerical scheme to compute $2$-fold rotationally symmetric equilibria for a single rotating hollow vortex we then show that its limiting configuration is one where a thin waist forms leading to two separate parts of its single boundary drawing close together. We give evidence that the limit of this single vortex configuration coincides with the limit of the two-vortex configuration. The limiting configuration itself turns out not to be physically admissible, leading to what we refer to as a topological singularity since no physical quantities blow up, indeed they appear to be continuous as the limiting state is approached from the two topologically distinct directions.

The generation of a tsunami wave by an aerial landslide is investigated through model laboratory experiments. We examine the collapse of an initially dry column of grains into a shallow water layer and the subsequent generation of waves. The experiments show that the collective entry of the granular material into water governs the wave generation process. We observe that the amplitude of the wave relative to the water height scales linearly with the Froude number based on the horizontal velocity of the moving granular front relative to the wave velocity. For all the different parameters considered here, the aspect ratio and the volume of the column, the diameter and density of the grains, and the height of the water, the granular collapse acts like a moving piston displacing the water. We also highlight that the density of the falling grains has a negligible influence on the wave amplitude, which suggests that the volume of grains entering the water is the relevant parameter in the wave generation.

We use well resolved numerical simulations with the lattice Boltzmann method to study Rayleigh–Bénard convection in cells with a fractal boundary in two dimensions for $Pr = 1$ and $Ra \in \left [10^7, 10^{10}\right ]$, where Pr and Ra are the Prandtl and Rayleigh numbers. The fractal boundaries are functions characterized by power spectral densities 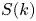$S(k)$ that decay with wavenumber, 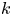$k$, as 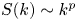$S(k) \sim k^{p}$ (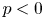$p < 0$). The degree of roughness is quantified by the exponent 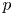$p$ with $p < -3$ for smooth (differentiable) surfaces and 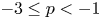$-3 \le p < -1$ for rough surfaces with Hausdorff dimension 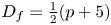$D_f=\frac {1}{2}(p+5)$. By computing the exponent 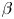$\beta$ using power law fits of 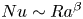$Nu \sim Ra^{\beta }$, where 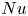$Nu$ is the Nusselt number, we find that the heat transport scaling increases with roughness through the top two decades of 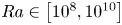$Ra \in \left [10^8, 10^{10}\right ]$. For 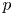$p$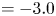$= -3.0$, 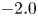$-2.0$ and $-1.5$ we find 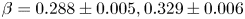$\beta = 0.288 \pm 0.005, 0.329 \pm 0.006$ and $0.352 \pm 0.011$, respectively. 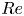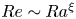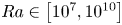We also find that the Reynolds number, $Re$, scales as $Re \sim Ra^{\xi }$, where $\xi \approx 0.57$ over $Ra \in \left [10^7, 10^{10}\right ]$, for all $p$ used in the study. For a given value of 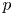$p$, the averaged 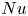$Nu$ and 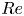$Re$ are insensitive to the specific realization of the roughness.

The ever-increasing need for optimized atmospheric-entry and hypersonic-cruise vehicles requires an understanding of the coexisting high-enthalpy phenomena. These phenomena strongly condition the development of instabilities leading to the boundary layer's transition to turbulence. The present article explores how shock waves, internal-energy-mode excitation, species interdiffusion, dissociation and ionization condition boundary-layer perturbation growth related to second-mode instabilities. Linear stability theory and the e$^{N}$ method are applied to laminar base flows over a $10^{\circ }$ wedge with an isothermal wall and free-stream conditions similar to three flight-envelope points in an extreme planetary return. The authors explore a wide range of boundary conditions and flow assumptions, on both the laminar base flow and the perturbation quantities, in order to decouple the various phenomena of interest. Under the assumptions of this study, the cooling of the laminar base flow due to internal-energy-mode excitation, dissociation and ionization is seen to be strongly destabilizing. However, species interdiffusion, dissociation and ionization acting on the perturbation terms are seen to have the opposite effect. The net result of these competing effects ultimately amounts to internal-energy-mode excitation and dissociation being destabilizing, and ionization being stabilizing. The appearance of unstable supersonic modes due to high-enthalpy effects is seen to be linked to the diffusion-flux perturbations, rather than the cooling of the laminar base flow (as is commonly believed). The use of the linearized shock boundary condition was seen to have a minor impact in the $N$-factor envelopes, despite the extremely low relative shock angle.

A modelling methodology is proposed and applied to effectively decouple many of the multiple physical phenomena simultaneously coexisting in boundary-layer-transition problems in the presence of an ablating thermal protection system. Investigations are based on linear stability theory and the semi-empirical $\textrm {e}^{\textrm {N}}$ method, and study the marginal contribution to second-mode-wave amplitudes of internal-energy-mode excitation, ablation-induced outgassing, ablation- and radiation-induced surface cooling, air- and carbon-species dissociation reactions, the interdiffusion of dissimilar species, surface chemistry and radiation and perturbation–shock interactions. The contributions of these phenomena are isolated by deploying a variety of flow assumptions, mixtures and boundary conditions with marginal increases in modelling complexity and generality. Internal-energy-mode excitation is seen to be the major contributor to the perturbation amplitudes for most conditions considered, whereas ablation-induced outgassing or the ablation- and radiation-induced modification of the surface-temperature distribution display a minor effect. Other phenomena are seen to have a variable contribution depending on the trajectory point, owing to the different ablation rates with which the thermal protection system decomposes. This is the case with the diffusion of carbon species injected through the surface, and the dissociation of air and carbon species. The use of a radiative equilibrium, rather than a homogeneous boundary condition on the temperature perturbation amplitude, is seen to increase the predicted growth of second-mode waves at all the trajectory points. Perturbation–shock interactions remarkably modify instability development only in scenarios with significant unstable supersonic modes. The substitution of all ablation subproducts for a single non-reacting species ($\textrm {CO}_{2}$) was acceptable as long as the flow chemistry can be assumed frozen. The use of inaccurate transport and diffusion models, rather than the state of the art, is seen to have a variable effect on the predictions, yet generally smaller than what was observed in previous work.

We examine a turbulent distributed wall-source plume: the flow resulting from a uniform vertical wall source of buoyancy such as that produced by an evenly heated or cooled vertical wall. The vertically distributed buoyancy source is created by forcing dense salt water solution through a porous wall. Velocity measurements on a vertical plane normal to the wall are first presented examining the full height of the wall in order to identify the region in which the bulk flow has become fully turbulent, self-similar and reached an invariant balance between the fluxes of volume, momentum and buoyancy. Simultaneous velocity and buoyancy field measurements are then presented in this region and an entrainment coefficient of $\alpha = 0.068 \pm 0.006$ is determined. This value is small compared to that of buoyancy-driven unbounded flows, e.g. a free line plume, and we reason this to be due to the presence of a rigid boundary restricting meandering and turbulence production, rather than the effect of the vertically distributed source of buoyancy. Turbulent velocity and buoyancy statistics are presented and, in order to gain physical insights into the flow behaviour, the results are compared to those of other canonical buoyancy-driven free and wall-bounded flows. We show that the bulk mixing of distributed wall-source plumes can be captured by consideration of the characteristic vertical velocities and a constant entrainment coefficient. This mixing is inhibited both by the presence of a rigid boundary and the reduced characteristic velocities (compared to those of wall line plumes).