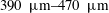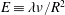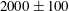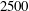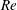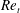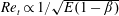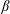Experiments are performed to characterize the onset of laminar–turbulent transition in the flow of high-molecular-weight polymer solutions in rigid microtubes of diameters in the range 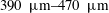$390~\unicode[STIX]{x03BC}\text{m}{-}470~\unicode[STIX]{x03BC}\text{m}$ using the micro-PIV technique. By considering flow in tubes of such small diameters, the present study probes higher values of elasticity numbers (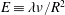$E\equiv \unicode[STIX]{x1D706}\unicode[STIX]{x1D708}/R^{2}$) compared to existing studies, where $\unicode[STIX]{x1D706}$ is the longest relaxation time of the polymer solution, $R$ is the tube radius and $\unicode[STIX]{x1D708}$ is the kinematic viscosity of the polymer solution. 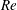For the Newtonian case, our experiments indicate that the natural transition (without the aid of any forcing mechanism) occurs at Reynolds number ($Re$) 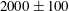$2000\pm 100$. As the concentration of polymer is increased, initially there is a delay in the onset of the transition and the transition Reynolds number increases to 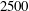$2500$. Further increase in concentration of the polymer results in a decrease in the Reynolds number for transition. At sufficiently high concentrations, the added polymer tends to destabilize the flow and the transition is observed to happen at 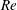$Re$ as low as $800$. It is also observed that the addition of polymers, regardless of their concentration, reduces the magnitude of the velocity fluctuations after transition. Dye-stream visualization is further used to corroborate the onset of transition in the flow of polymer solutions. The present work thus shows that addition of polymer, at sufficiently high concentrations, destabilizes the flow when compared to that of a Newtonian fluid, thereby providing additional evidence for ‘early transition’ or ‘elasto-inertial turbulence’ in the flow of polymer solutions. The data for the transition Reynolds number 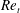$Re_{t}$ from our experiments (for tubes of different diameters, and for two different polymers at varying concentrations) collapse well according to the scaling relation 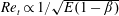$Re_{t}\propto 1/\sqrt{E(1-\unicode[STIX]{x1D6FD})}$, where 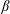$\unicode[STIX]{x1D6FD}$ is the ratio of solvent viscosity to the viscosity of the polymer solution.

Water entry has been studied for over a century, but few studies have focused on multiple droplets impacting on a liquid bath sequentially. We connect multi-droplet streams, jets and solid objects with physical-based scaling arguments that emphasize the intrinsically similar cavities. In particular, the cavities created by the initial impact of both droplet streams and jets on an initially quiescent liquid pool exhibit the same types of cavity seal as hydrophobic spheres at low Bond number, some of which were previously unseen for jets and droplet streams. Low-frequency droplet streams exhibit an additional three new cavity seal types unseen for jets or solid spheres that can be predicted with a new non-dimensional frequency. The cavity depth and cavity velocity for both droplet and jet impact are rationalized by an energy scaling analysis and the Bernoulli equation.

Surface tension causes the edge of a fluid sheet to retract. If the sheet is also stretched along its edge then the flow and the rate of retraction are modified. A universal similarity solution for the Stokes flow in a stretched edge shows that the scaled shape of the edge is independent of the stretching rate, and that it decays exponentially to its far-field thickness. This solution justifies the use of a stress boundary condition in long-wavelength models of stretched viscous sheets, and gives the detailed shape of the edge of such a sheet, resolving the position of the sheet edge to the order of the thickness.

Control of laminar-to-turbulent transition on a swept-wing is achieved by base-flow modification in an experimental framework, up to a chord Reynolds number of 2.5 million. This technique is based on the control strategy used in the numerical simulation by Dörr & Kloker (J. Phys. D: Appl. Phys., vol. 48, 2015b, 285205). A spanwise uniform body force is introduced using dielectric barrier discharge plasma actuators, to either force against or along the local cross-flow component of the boundary layer. The effect of forcing on the stability of the boundary layer is analysed using a simplified model proposed by Serpieri et al. (J. Fluid Mech., vol. 833, 2017, pp. 164–205). A minimal thickness plasma actuator is fabricated using spray-on techniques and positioned near the leading edge of the swept-wing, while infrared thermography is used to detect and quantify transition location. Results from both the simplified model and experiment indicate that forcing along the local cross-flow component promotes transition while forcing against successfully delays transition. This is the first experimental demonstration of swept-wing transition delay via base-flow modification using plasma actuators.