The system allowing a user to operate at the server sed.sao.ru with simulated curves of spectral energy distributions (SED) and to estimate ages and redshifts from photometric data is described.

Recent investigations of high-redshift QSO morphology are reviewed and compared. The PSF-removal is difficult and results are given in several different forms. The reliability of results from HST and ground-based AO are compared, and several caveats to conventional wisdom are given.

The presence or absence of a nucleus is considered for a sample of galaxies limited by distance, not by flux. For the 365 nearest galaxies situated within a distance of ~7 Mpc, classification of their central regions is based on large-scale images obtained with the Hubble Space Telescope as well as with the 6-m and other large ground-based telescopes. Occurrence of nucleated galaxies is considered as a function of their luminosity, morphological type and other global properties.

High angular resolution observations from WFPC and STIS now allow well-constrained dynamical measurement of the masses of supermassive black holes (SMBH) in nearby galaxies. An initial statistical analysis by Magorrian et al. showed that 97% of bulges host SMBH. Black hole mass is correlated moderately with bulge luminosity and strongly with the velocity dispersion of the whole bulge, suggesting that black hole formation may be an intrinsic aspect of bulge formation. Black hole masses for AGN determined from reverberation mapping fall on the same relationship with bulge velocity dispersion as those determined from stellar dynamical measurements. The prospect is therefore that the large-scale distribution of black hole masses in distant quasars may be determined through relatively straightforward measurement. Integral constraints show consistency between the total AGN luminosity density and the total volume density in SMBH contained in galaxy bulges. The strong peak of the high-luminosity quasar luminosity function at early cosmic time is consistent with the association of the build-up of SMBH through accretion and bulge formation. Alternate scenarios requiring substantial build-up of the most massive black holes at later cosmic times are more difficult to reconcile with the evolution of the LF.

It is still an open question whether the super-massive black holes thought to be present in quasars are of primordial nature, or whether there is a viable way of forming them in the very short time scale (less than a billion years) permitted by the observational data. In this contribution, we present a way in which a galaxy-galaxy merger can provide not only the “fuel” for quasar activity, but can also build a super-massive black hole, i.e., “the engine”, in the first place.

In this paper results from a monitoring programme of a large sample of quasars comprising regular yearly observations over a period of 23 years are presented. Structure functions of the light curves are calculated and compared with predictions for models of quasar variability of current interest. These include recently published models of varibility from accretion disk instability, variability from starbursts or supernovae, and variations caused by the microlensing effect of compact bodies along the line of sight. The analysis favours the accretion disk model for low luminosity AGN, but suggests that the variations of more luminous quasars are dominated by microlensing.

We report on implications for the geometrical and kinematic parameters of BLR gas on the basis of short timescale variability in the broad Hβ profile.

Data on rapid variations have been obtained at the 6-m telescope of the SAO (Asatrian, Khachikian & Notni, 1999). To search for variations in the profile shape, difference spectra (first minus second epoch) were examined. We believe that the structure of the underlying stellar continuum and the atmospheric features do not affect the Hβ difference profiles of 3C 390.3 significantly.

Variations occurred simultaneously on the blue and red sides of Hβ on a timescale of ~ 1.452 hours and take the form of three narrow, positive and negative small bumps drifting across the line profile in the difference spectrum. The positions of the bumps are −2300, +4700 (negative) and −3700 kms2212;1 (positive).

We report on implications for the geometrical and kinematic parameters of BLR gas on the basis of short timescale variability in the broad Hα profile shape. Data on rapid variations have been obtained at the 2.6−m telescope of the BAO (Asatrian, Khachikian & Notni, 1999). To search for variations in the profile, difference spectra (second minus first epoch) were examined. We believe that the structure of the underlying stellar continuum and the atmospheric features do not affect significantly the Hα difference profiles of Mark 6. Variations occurred simultaneously on the blue and red sides of Hα on a time scale of ≃ 50.7 minutes and take the form of three narrow, positive small bumps on each side in the difference spectrum. The positions of the bumps are −4400, −3100, −1700 and +1900, +4200 and +6600 kms−1. These changes may indicate the response of circularly rotating emitting gas at three orbits to a light pulse from a central source. In this case the pairs of blue and red bumps observed at −4400 and +6600, −3100 and +4200, and −1700 and 1900 km s−1 are formed in two opposite zones of gas close to the line of nodes. On the assumption that these orbits lie around a central massive object, orbital parameters (radii, velocities and inclination angles of orbital planes) of the clouds and the central mass can be found. The shift of each bump is defined by the combination of the relativistic Doppler effect due to the Keplerian orbital motion and the gravitational redshift. The six observed radial velocities are determined by six parameters: the orbital radii, R1, R2, R3 (or velocities, Vi, V2, V3) and the inclination angles i1, i2, i3 of the rotation planes. Thus, the expressions for the radial velocities form a system of six algebraic equations with six unknowns and can be solved. Using the difference of the orbital radii in absolute units (R3 − R1 = Δt C, where ΔtC, ≃ 50.7 minutes and C is the speed of light) we can derive the central mass M. Analytical solution gives: 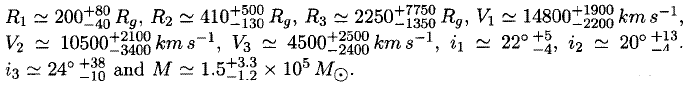Error limits for the results are determined by the uncertainty of the input radial velocities, 300 km s−1. However, the value of the mass obtained is smaller by two orders of magnitude than the estimate for the dynamical mass of the nucleus of Mark 6 (M = 1 × 107M⊙ Dibai 1984). Such a low mass is excluded, therefore.

The very first spectrograms of 40 Seyfert galaxies are discussed. All these objects were taken from Markarian’s lists of galaxies. They were first investigated and classified at Fesenkov Astrophysical Institute during 1973-1988. Spectral study was carried out with a slit spectrograph, attached to the 70-cm telescope and equipped with a three cascades image-tube. Repeated observations of 22 galaxies were obtained in 1985-2000. An analysis of possible spectral variability of the studied galaxies over 10-26 years was made. A list of objects which are the most interesting for further observations is presented.

The analysis of multicolor observations of BL Lac obtained in the 1997 and 1999 outbursts shows that in both cases the spectral energy distribution of the variable source was unchanged in the course of outburst. The sources have power-law spectra with slightly different spectral indices (the spectrum was flatter in the more powerful outburst of 1997). The variable source is most probably of synchrotron nature.

In all probability, AGN are one of the most convincing observable gleams of the Superforce in the Universe.

Seyfert galaxies with Z-shaped emission filaments in the Narrow Line Region (NLR) are considered. We assume that observable Z-shaped structures and the velocity pattern of the NLR may be explained as tri-dimensional helical waves in the ionization cone.

The correlation of optical and X-ray radiation of some AGN is studied. We used low signal-to-noise data from the All-Sky Monitor of the Rossi X-ray Timing Explorer (2-10 KeV) and broad-band photometry of the optical continuum obtained mainly during the last several years through the "AGN Watch" program.

We consider an accreting central symmetric object that is near to the Eddington limit, i.e., the luminosity pressure is balancing the gravity. In special cases, a layer of accreting matter will be formed near the surface of a centrally symmetric object. We discuss the conditions of formation of the layer, its inner structure and stability. If the luminosity pressure will be reduced for some reason, then the layer will collapse immediately, releasing an enormous quantity (up to 1042 erg/sec) of energy. We discuss some possible scenarios for energy releases.

The variations of the Hβ broad emission line and continuum flux in 3C 390.3 are investigated. Our results favor the formation of the broad Hβ line of 3C 390.3 n an accretion disk.

Spectra of Mrk 474 have been obtained in order to search for short-term (less than one day) variability in the broad Hβ and Hα profiles. Observations were carried out using a slit spectrograph and a three-cascade image tube attached to the 70-cm reflector. The processing of the series of spectrograms, obtained on 1999 April 25, showed a rapid (less than 20 minutes) and powerful increase of the flux in the region of [O III]λ5007. The increase of the flux near maximum (5060Å ± 10Å)appeared to be equal approximately to 300%. The value similar to the FWHM for the long-wavelength excess of the flux was equal to 170Å ± 20Å. The dying-out of the long-wavelength excess lasted approximately two hours.

We live in an exciting era that offers increasing opportunities for people all over the world to make discoveries about the Universe using interconnected archives on the Internet as a primary research tool. We review how NED (http://ned.ipac.caltech.edu) can be used in concert with globally distributed online archives to perform multi-wavelength, crosscorrelated studies of AGNs and other galaxy types. The present status and planned evolution of NED capabilities are discussed.

The aim of this paper is to describe new statistical methods for determination of the correlations among and distributions of physical parameters from a multivariate data with general and arbitrary truncations and selection biases. These methods, developed in collaboration with B. Efron of Department of Statistics at Stanford, can be used for analysis of combined data from many surveys with different and varied observational selection criteria. For clarity I will use the luminosity function of AGNs and its evolution to demonstrate the methods. I will first describe the general features of data truncation and present a brief review of past methods of analysis. Then I will describe the new methods and results from simulations testing their accuracy. Finally I will present the results from application of the methods to a sample of quasars.

The First Byurakan Survey (FBS) is the largest spectral survey in the Northern sky. Its plates contain low-dispersion spectra for some 20,000,000 objects. The FBS spectra allow selection of objects by their color, broad emission or absorption lines, or SED; to discover, classify and investigate them. The FBS was conducted originally to search for UVX galaxies (1500 Markarian galaxies were discovered). Selection of blue stellar objects, red stars, and identification of IRAS sources have also been done by means of the FBS spectra. All these projects have been carried out by visual inspection of the plates. Digitization will give new possibilities to search for many new objects: new bright QSOs (m<18m), new Markarian (UVX) galaxies, BCDGs, optical counterparts of IR, radio, X-ray and other sources, late-type stars, planetary nebulae, emission-line stars, and white dwarfs; and to study star clusters and clusters of galaxies. The digitized FBS will be available via the Internet and on CDs. Software and an appropriate interface for working with the data will be provided.

This conference on AGN Surveys has proved to be a significant milestone in our understanding of the redshift distribution of optically selected QSOs, and in our initial understanding of the cosmic distribution of AGN from the first far-infrared and X-ray deep fields. It has also set the stage for continuing debates concerning the multiwave-length properties of AGN, the cosmological distribution of “obscured” AGN, and the “orientation versus evolution” debate on the nature of the sources discovered at different wavelengths. Much of this debate could have been anticipated from previous studies of the complete samples of optically-selected AGN provided to us by the pioneering work carried out by the staff of the Byurakan Astronomical Observatory over the past 40 years.