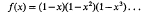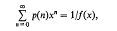Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Thanigasalam, K.
1974.
Congruence Properties of Certain Restricted Partitions.
Mathematics Magazine,
Vol. 47,
Issue. 3,
p.
154.
Andrews, George E.
1974.
Applications of Basic Hypergeometric Functions.
SIAM Review,
Vol. 16,
Issue. 4,
p.
441.
Fomenko, O. M.
1980.
Applications of the theory of modular forms to number theory.
Journal of Soviet Mathematics,
Vol. 14,
Issue. 4,
p.
1307.
Ramanathan, K G
1980.
Ramanujan and the congruence properties of partitions.
Proceedings of the Indian Academy of Sciences - Section A,
Vol. 89,
Issue. 3,
Gordon, B.
and
Hughes, K.
1981.
Analytic Number Theory.
Vol. 899,
Issue. ,
p.
333.
Costello, Patrick J.
1982.
Density problems involving 𝑝ᵣ(𝑛).
Mathematics of Computation,
Vol. 38,
Issue. 158,
p.
633.
Gordon, Basil
1983.
Ramanujan congruences for p-k (mod 11').
Glasgow Mathematical Journal,
Vol. 24,
Issue. 2,
p.
107.
Debnath, Lokenath
1987.
Srinivasa Ramanujan (1887‐1920) A centennial tribute.
International Journal of Mathematical Education in Science and Technology,
Vol. 18,
Issue. 6,
p.
821.
Garvan, F. G.
1988.
New combinatorial interpretations of Ramanujan’s partition congruences mod 5,7 and 11.
Transactions of the American Mathematical Society,
Vol. 305,
Issue. 1,
p.
47.
Garvan, Frank
Kim, Dongsu
and
Stanton, Dennis
1990.
Cranks andt-cores.
Inventiones Mathematicae,
Vol. 101,
Issue. 1,
p.
1.
Eichhorn, Dennis
and
Ono, Ken
1996.
Analytic Number Theory.
p.
309.
Garvan, F. G.
2001.
More cranks andt-cores.
Bulletin of the Australian Mathematical Society,
Vol. 63,
Issue. 3,
p.
379.
Ahlgren, Scott
and
Ono, Ken
2001.
Congruence properties for the partition function.
Proceedings of the National Academy of Sciences,
Vol. 98,
Issue. 23,
p.
12882.
Berndt, Bruce C.
and
Ono, Ken
2001.
The Andrews Festschrift.
p.
39.
Das Adhikari, Sukumar
and
Mukhopadhyay, Anirban
2001.
Partition function congruences: Some flowers and seeds from ‘Ramanujan's garden’.
Expositiones Mathematicae,
Vol. 19,
Issue. 3,
p.
193.
Ono, Ken
2001.
Special Functions 2000: Current Perspective and Future Directions.
p.
243.
Lovejoy, Jeremy
and
Ono, Ken
2002.
Extension of Ramanujan's congruences for the partition function modulo powers of 5.
Journal für die reine und angewandte Mathematik (Crelles Journal),
Vol. 2002,
Issue. 542,
Andrews, George E.
2003.
Number Theory and Modular Forms.
Vol. 10,
Issue. ,
p.
385.
Kronholm, Brandt
2005.
On congruence properties of 𝑝(𝑛,𝑚).
Proceedings of the American Mathematical Society,
Vol. 133,
Issue. 10,
p.
2891.
Alladi, Krishnaswami
Andrews, George E.
Ono, Ken
and
McIntosh, Richard J.
2006.
On the work of Basil Gordon.
Journal of Combinatorial Theory, Series A,
Vol. 113,
Issue. 1,
p.
21.