Published online by Cambridge University Press: 07 November 2017
We give conditions under which non-uniformly expanding maps exhibit lower bounds of polynomial type for the decay of correlations and for a large class of observables. We show that if the Lasota–Yorke-type inequality for the transfer operator of a first return map is satisfied in a Banach space 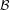 ${\mathcal{B}}$, and the absolutely continuous invariant measure obtained is weak mixing, in terms of aperiodicity, then, under some renewal condition, the maps have polynomial decay of correlations for observables in
${\mathcal{B}}$, and the absolutely continuous invariant measure obtained is weak mixing, in terms of aperiodicity, then, under some renewal condition, the maps have polynomial decay of correlations for observables in 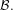 ${\mathcal{B}}.$ We also provide some general conditions that give aperiodicity for expanding maps in higher dimensional spaces. As applications, we obtain lower bounds for piecewise expanding maps with an indifferent fixed point and for which we also allow non-Markov structure and unbounded distortion. The observables are functions that have bounded variation or satisfy quasi-Hölder conditions and have their support bounded away from the neutral fixed points.
${\mathcal{B}}.$ We also provide some general conditions that give aperiodicity for expanding maps in higher dimensional spaces. As applications, we obtain lower bounds for piecewise expanding maps with an indifferent fixed point and for which we also allow non-Markov structure and unbounded distortion. The observables are functions that have bounded variation or satisfy quasi-Hölder conditions and have their support bounded away from the neutral fixed points.