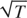The issue of identification of covariance structures, which arises in
a number of different contexts, has been so far linked to conditions on
the true parameters to be estimated. In this paper, this limitation is
removed.
As done by Johansen (1995, Journal of
Econometrics 69, 112–132) in the context of linear models, the
present paper provides necessary and sufficient conditions for the
identification of a covariance structure that depends only on the
constraints and can therefore be checked independently of estimated
parameters.
A structure condition is developed, which only depends on the
structure of the constraints. It is shown that this condition, if coupled
with the familiar order condition, provides a sufficient condition for
identification. In practice, because the structure condition holds if and
only if a certain matrix, constructed from the constraint matrices, is
invertible, automatic software checking for identification is feasible
even for large-scale systems.
Most of the paper focuses on structural vector autoregressions, but
extensions to other statistical models are also briefly discussed.I thank all the participants at the meeting held
in Pavia on June 11, 2004, in honor of Carlo Giannini for their comments;
it goes without saying that Carlo himself provided not only acute
observations on the day but also the main inspiration for this piece of
work. Sadly, Carlo passed away on September 11, 2004, and this paper is
dedicated to his memory. Pär Österholm spotted several mistakes
in an earlier version and helped me clarify some implementation details.
Thanks are also due to Gianni Amisano, Bruce Hansen, Giulio Palomba, Paolo
Paruolo, and two anonymous referees. The usual disclaimer obviously
applies.